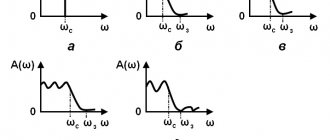
Harmonic and non-harmonic signals.
And first, let’s take a closer look at how signals are classified. First of all, we are interested in periodic signals. Their shape repeats after a certain time interval T, called a period. Periodic signals, in turn, are divided into two large classes - harmonic and non-harmonic. A harmonic signal is a signal that can be described by the following function:
y = A cos(wt + \phi)
Here A is the signal amplitude, w is the cyclic frequency, and \phi is the initial phase. A logical question may arise - isn't a sine wave a harmonic signal? Of course, it is, the fact is that sin\alpha = cos(\frac{\pi}{2}\medspace-\medspace \alpha) - that is, the signals differ in the initial phase, accordingly, a sinusoidal signal does not contradict the definition that we given for harmonic vibrations.
The second subclass of periodic signals are non-harmonic oscillations . Here is an example of a non-harmonic signal:
As you can see, despite its appearance, the signal remains periodic, that is, its shape repeats at a time interval equal to the period.
To work with such signals and study them, there is a certain technique, which consists in decomposing the signal into a Fourier series . The bottom line is that a non-harmonic periodic signal (if certain conditions are met) can be represented as a sum of harmonic oscillations with certain amplitudes, frequencies and initial phases. An important nuance is that all harmonic oscillations that participate in the summation must have frequencies that are multiples of the frequency of the original non-harmonic signal. This may not be entirely clear yet, so let’s look at a practical example and take a closer look. And for example, we use the signal that is shown in the figure just above. It can be represented as follows:
u(t) = u_1(t) + u_2(t) = 2 sin(t) + 1.5 sin(2t)
Let's display all these signals on one chart:
The functions u_1(t), u_2(t) are called harmonics of the signal, and the one whose period is equal to the period of the non-harmonic signal is called the first or fundamental harmonic . In this case, the first harmonic is the function u_1(t) (its frequency is equal to the frequency of the non-harmonic signal under study; accordingly, their periods are equal). And the function u_2(t) = 1.5 sin(2t) is nothing more than the second harmonic of the signal (its frequency is twice as high). In the general case, a non-harmonic signal is decomposed into an infinite number of harmonics:
u(t) = U_0 + \sum_{i=0}^{\infty}{U_{k}\thinspace sin(\thinspace kwt + \phi_k\thinspace )}
Here U_k is the amplitude, and \phi_k is the initial phase of the k-th harmonic. As we mentioned a little earlier, the frequencies of all harmonics are multiples of the frequency of the first harmonic, in fact, this is what we see in this formula. U_0 is the zero harmonic, its frequency is 0, it is equal to the average value of the function over the period. Why average? Look - the average value of the sine function over the period is 0, which means that when averaging in this formula, all terms except U_0 will be equal to 0