Each measurement is a comparison of the measured quantity with another homogeneous quantity, which is considered unitary. Theoretically, the units for all quantities in physics can be chosen to be independent of each other. But this is extremely inconvenient, since for each value one should enter its own standard. In addition, in all physical equations that reflect the relationship between different quantities, numerical coefficients would arise.
The main feature of the currently used systems of units is that there are certain relationships between units of different quantities. These relationships are established by the physical laws (definitions) that relate the measured quantities to each other. Thus, the unit of speed is chosen in such a way that it is expressed in terms of units of distance and time. When selecting speed units, the speed definition is used. The unit of force, for example, is established using Newton's second law.
When constructing a specific system of units, several physical quantities are selected, the units of which are set independently of each other. Units of such quantities are called basic. The units of other quantities are expressed in terms of the basic ones, they are called derivatives.
Table of units of measurement “Space and time”
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Length | l, s, d | meter | m | The extent of an object in one dimension. | |
| Square | S | square meter | m2 | The extent of an object in two dimensions. | |
| Volume, capacity | V | cubic meter | m3 | The extent of an object in three dimensions. | extensive quantity |
| Time | t | second | With | Duration of the event. | |
| Flat angle | α , φ | radian | glad | The amount of change in direction. | |
| Solid angle | α , β , γ | steradian | Wed | Part of space | |
| Linear speed | v | meter per second | m/s | The speed of changing body coordinates. | vector |
| Linear acceleration | a,w | meters per second squared | m/s2 | The rate of change in the speed of an object. | vector |
| Angular velocity | ω | radians per second | rad/s = (s−1) | Angle change rate. | |
| Angular acceleration | ε | radian per second squared | rad/s2 = (s−2) | Rate of change of angular velocity |
Table of units of measurement "Mechanics"
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Weight | m | kilogram | kg | A quantity that determines the inertial and gravitational properties of bodies. | extensive quantity |
| Density | ρ | kilogram per cubic meter | kg/m3 | Mass per unit volume. | intensive quantity |
| Surface density | ρA | Mass per unit area. | kg/m2 | Ratio of body mass to surface area | |
| Linear density | ρl | Mass per unit length. | kg/m | Ratio of body mass to its linear parameter | |
| Specific volume | v | cubic meter per kilogram | m3/kg | Volume occupied by a unit mass of a substance | |
| Mass flow | Qm | kilogram per second | kg/s | The mass of a substance that passes through a given cross-sectional area of a flow per unit time | |
| Volume flow | Qv | cubic meter per second | m3/s | Volume flow of liquid or gas | |
| Pulse | P | kilogram-meter per second | kg•m/s | Product of mass and speed of a body. | extensive, conserved quantity |
| Momentum | L | kilogram-meter squared per second | kg•m2/s | A measure of the rotation of an object. | conserved quantity |
| Moment of inertia | J | kilogram meter squared | kg•m2 | A measure of the inertia of an object during rotation. | tensor quantity |
| Strength, weight | F, Q | newton | N | An external cause of acceleration acting on an object. | vector |
| Moment of power | M | newton meter | N•m = (kg m2/s2) | The product of a force and the length of a perpendicular drawn from a point to the line of action of the force. | vector |
| Impulse force | I | newton second | N•s | Product of force and the duration of its action | vector |
| Pressure, mechanical stress | p , σ | pascal | Pa = ( kg/(m s2)) | Force per unit area. | intensive quantity |
| Job | A | joule | J = (kg m2/s2) | Dot product of force and displacement. | scalar |
| Energy | E, U | joule | J = (kg m2/s2) | The ability of a body or system to do work. | extensive, conserved quantity, scalar |
| Power | N | watt | W = (kg m2/s3) | Rate of change of energy. |
What is strength?
If a body accelerates, then something acts on it. How to find this “something”? For example, what kind of forces act on a body near the surface of the earth? This is the force of gravity directed vertically downward, proportional to the mass of the body and for heights much smaller than the radius of the earth ${\large R}$, almost independent of the height; it is equal
${\large F = \dfrac {G \cdot m \cdot M}{R^2} = m \cdot g }$
Where
${\large g = \dfrac {G \cdot M}{R^2} }$
the so-called acceleration of gravity. In the horizontal direction the body will move at a constant speed, but the movement in the vertical direction is according to Newton's second law:
${\large m \cdot g = m \cdot \left ( \dfrac {d^2 \cdot x}{d \cdot t^2} \right ) }$
after contracting ${\large m}$, we find that the acceleration in the direction ${\large x}$ is constant and equal to ${\large g}$. This is the well-known motion of a freely falling body, which is described by the equations
${\large v_x = v_0 + g \cdot t}$
${\large x = x_0 + x_0 \cdot t + \dfrac {1}{2} \cdot g \cdot t^2}$
Table of units of measurement “Periodic phenomena, oscillations and waves”
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Period | T | second | With | The period of time during which the system makes one complete oscillation | |
| Batch frequency | v, f | hertz | Hz = (s−1) | The number of repetitions of an event per unit of time. | |
| Cyclic (circular) frequency | ω | radians per second | rad/s | Cyclic frequency of electromagnetic oscillations in an oscillatory circuit. | |
| Rotation frequency | n | second to the minus first power | s-1 | A periodic process equal to the number of complete cycles completed per unit of time. | |
| Wavelength | λ | meter | m | The distance between two points in space closest to each other at which the oscillations occur in the same phase. | |
| Wave number | k | meter to the minus first power | m-1 | Spatial wave frequency |
Some practical issues and inductor designs
In practice, various designs of inductors are used. Depending on the purpose and area of application, the devices can be made in different ways, but the effects that occur in real coils must be taken into account.
Inductor quality factor
A real coil, in addition to inductance, has several other parameters, and one of the most important is the quality factor. This value determines the losses in the coil and depends on:
- ohmic losses in the winding wire (the higher the resistance, the lower the quality factor);
- dielectric losses in wire insulation and winding frame;
- screen losses;
- core losses.
All these quantities determine the loss resistance, and the quality factor is a dimensionless quantity equal to Q=ωL/Rloss, where:
- ω = 2*π*F – circular frequency;
- L – inductance;
- ωL is the reactance of the coil.
Table of units of measurement “Thermal phenomena”
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Temperature | T | kelvin | TO | The average kinetic energy of the object's particles. | Intensive value |
| Temperature coefficient | α | kelvin to the minus first power | K-1 | Dependence of electrical resistance on temperature | |
| Temperature gradient | gradT | kelvin per meter | K/m | Change in temperature per unit length in the direction of heat propagation. | |
| Heat (amount of heat) | Q | joule | J = (kg m2/s2) | Energy transferred from one body to another by non-mechanical means | |
| Specific heat | q | joule per kilogram | J/kg | The amount of heat that must be supplied to a substance taken at its melting point in order to melt it. | |
| Heat capacity | C | joule per kelvin | J/C | The amount of heat absorbed (released) by a body during the heating process. | |
| Specific heat | c | joule per kilogram kelvin | J/(kg•K) | Heat capacity of a unit mass of a substance. | |
| Entropy | S | joule per kilogram | J/kg | A measure of the irreversible dissipation of energy or the uselessness of energy. |
Examples of typical currents
Current values can be read on information plates on electrical receivers or in the manuals for these devices. The table below shows typical values of electric currents for various electrical receivers.
| Consumer | Current strength |
| Electric thermometer | about 0.00001 mA |
| Headphones | 1 mA |
| Incandescent lamp 60 W | 0.26 A |
| Incandescent lamp 75 W | 0.33 A |
| Fridge | 0.8 A |
| Smartphone charger (fast charging) | 2 A |
| Personal Computer | 0.87 - 2.6 A |
| Microwave | 3.5 A |
| Vacuum cleaner | 4 - 9 A |
| Washing machine | 6 - 10 A |
| Electric melting furnace | 15000 A |
| Thunderstorm lightning | 10,000 - 100,000 A (average 36,000 A) |
Table of units of measurement "Molecular physics"
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Quantity of substance | v, n | mole | mole | The number of similar structural units that make up a substance. | Extensive value |
| Molar mass | M , μ | kilogram per mole | kg/mol | The ratio of the mass of a substance to the number of moles of that substance. | |
| Molar energy | Hmol | joule per mole | J/mol | Energy of a thermodynamic system. | |
| Molar heat capacity | resins | joule per mole kelvin | J/(mol•K) | The heat capacity of one mole of a substance. | |
| Molecular concentration | c, n | meter to the minus third power | m-3 | The number of molecules contained in a unit volume. | |
| Mass concentration | ρ | kilogram per cubic meter | kg/m3 | The ratio of the mass of a component contained in a mixture to the volume of the mixture. | |
| Molar concentration | resins | mole per cubic meter | mol/m3 | The content of the component relative to the entire mixture. | |
| Ion mobility | V , μ | square meter per volt second | m2/(V•s) | The proportionality coefficient between the drift velocity of carriers and the applied external electric field. |
What does voltage depend on?
Current unit
The voltage indicator recorded on a section of the electrical circuit depends on a number of factors, for example, on the connected load (resistance). Also influenced by the characteristics of the material from which the conductor element is made, the ambient temperature and the network components themselves.
Josephson effect
This is the name for the phenomenon of superconducting current passing through a layer of thin dielectric material, isolating one superconducting object from another. In the scientific work of the scientist whose name the effect is named, it was suggested that this phenomenon is observed only when using a superthin layer (significantly smaller than the superconducting coherence length). Later experiments demonstrated that it also manifests itself when using much thicker layers.
The use of this phenomenon will allow for high-precision measurements of voltage, as well as magnetic fields. The latter is made possible due to the huge dependence of the electric current, critical for the connection used in the interferometer, on the external magnetic field. When a Josephson junction is maintained at a constant voltage, it can act as a generator of electromagnetic wave radiation. You can also organize an installation with the opposite, absorbing effect. Moreover, both generation and reception are capable of operating in a frequency range inaccessible to other means.
Research is also underway into the effect under consideration and the magnetic field transfer phenomena based on it for the transmission and accumulation of data (quantum computers). The first experimental processor of this type was designed by Japanese engineers. In 2014, employees of the physics department of Moscow State University designed a microcircuit for a computer using the properties of superconductors and this effect.
Table of units of measurement "Electricity and magnetism"
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Current strength | I | ampere | A | Charge flowing per unit time. | |
| Current Density | j | ampere per square meter | A/m2 | The strength of the electric current flowing through a surface element of unit area. | Vector quantity |
| Electric charge | Q, q | pendant | Cl = (A s) | The ability of bodies to be a source of electromagnetic fields and to take part in electromagnetic interaction. | extensive, conserved quantity |
| Electric dipole moment | p | coulomb meter | Kl•m | Electrical properties of a system of charged particles in the sense of the field it creates and the effect of external fields on it. | |
| Polarization | P | pendant per square meter | C/m2 | Processes and states associated with the separation of any objects, mainly in space. | |
| Voltage | U | volt | IN | Change in potential energy per unit charge. | scalar |
| Potential, EMF | φ, σ | volt | IN | The work of external forces (non-Coulomb) to move a charge. | |
| Electric field strength | E | volt per meter | V/m | The ratio of the force F acting on a stationary point charge placed at a given point in the field to the magnitude of this charge q | |
| Electrical capacity | C | farad | F | A measure of a conductor's ability to store electrical charge | |
| Electrical resistance | R,r | ohm | Ohm = (m2 kg/(s3 A2)) | resistance of an object to the passage of electric current | |
| Electrical resistivity | ρ | ohm meter | Ohm•m | The ability of a material to prevent the passage of electric current | |
| Electrical conductivity | G | Siemens | Cm | The ability of a body (medium) to conduct electric current | |
| Magnetic induction | B | tesla | Tl | Vector quantity, which is the force characteristic of the magnetic field | Vector quantity |
| Magnetic flux | F | weber | Wb = (kg/(s2 A)) | A value that takes into account the intensity of the magnetic field and the area it occupies. | |
| Magnetic field strength | H | ampere per meter | Vehicle | The difference between the magnetic induction vector B and the magnetization vector M | Vector quantity |
| Magnetic moment | pm | ampere square meter | A•m2 | A quantity characterizing the magnetic properties of a substance | |
| Magnetization | J | ampere per meter | Vehicle | A quantity characterizing the magnetic state of a macroscopic physical body. | vector quantity |
| Inductance | L | Henry | Gn | The proportionality coefficient between the electric current flowing in any closed circuit and the total magnetic flux | |
| Electromagnetic energy | N | joule | J = (kg m2/s2) | Energy contained in an electromagnetic field | |
| Volumetric energy density | w | joule per cubic meter | J/m3 | Electric field energy of a capacitor | |
| Active power | P | watt | W | AC power | |
| Reactive power | Q | var | var | A quantity characterizing the loads created in electrical devices by fluctuations in the energy of the electromagnetic field in the alternating current circuit | |
| Full power | S | watt-ampere | W•A | Total power, taking into account its active and reactive components, as well as deviations of the current and voltage waveforms from harmonic |
Moment of power
The moment of force is the vector product of the radius vector drawn from the axis of rotation to the point of application of the force and the vector of this force. Those. According to the classical definition, the moment of force is a vector quantity. Within the framework of our problem, this definition can be simplified to the following: the moment of force ${\large \overrightarrow{F}}$ applied to a point with coordinate ${\large x_F}$, relative to the axis located at point ${\large x_0 }$ is a scalar quantity equal to the product of the force modulus ${\large \overrightarrow{F}}$ and the force arm - ${\large \left | x_F — x_0 \right |}$. And the sign of this scalar quantity depends on the direction of the force: if it rotates the object clockwise, then the sign is plus, if counterclockwise, then the sign is minus.
It is important to understand that we can choose the axis arbitrarily - if the body does not rotate, then the sum of the moments of forces about any axis is zero. The second important note is that if a force is applied to a point through which an axis passes, then the moment of this force about this axis is equal to zero (since the arm of the force will be equal to zero).
Let us illustrate the above with an example in Fig. 2. Let us assume that the system shown in Fig. 2 is in equilibrium. Consider the support on which the loads stand. It is acted upon by 3 forces: ${\large \overrightarrow{N_1},\ \overrightarrow{N_2},\ \overrightarrow{N},}$ points of application of these forces A , B and C , respectively. The figure also contains forces ${\large \overrightarrow{N_{1}^{gr}},\ \overrightarrow{N_2^{gr}}}$. These forces are applied to the loads, and according to Newton's 3rd law
${\large \overrightarrow{N_{1}} = — \overrightarrow{N_{1}^{gr}}}$
${\large \overrightarrow{N_{2}} = — \overrightarrow{N_{2}^{gr}}}$
Now consider the condition for the equality of the moments of forces acting on the support relative to the axis passing through point A (and, as we agreed earlier, perpendicular to the plane of the drawing):
${\large N \cdot l_1 — N_2 \cdot \left ( l_1 +l_2 \right ) = 0}$
Please note that the moment of the force ${\large \overrightarrow{N_1}}$ was not included in the equation, since the arm of this force relative to the axis in question is equal to ${\large 0}$. If for some reason we want to choose an axis passing through point C , then the condition for equality of moments of force will look like this:
${\large N_1 \cdot l_1 — N_2 \cdot l_2 = 0}$
It can be shown that, from a mathematical point of view, the last two equations are equivalent.
Table of units of measurement “Optics, electromagnetic radiation”
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| The power of light | J, I | candela | cd | The amount of light energy emitted in a given direction per unit time. | Luminous, extensive value |
| Light flow | F | lumen | lm | Physical quantity characterizing the amount of “light” power in the corresponding radiation flux | |
| Light energy | Q | lumen-second | lm•s | Physical quantity characterizes the ability of energy transferred by light to cause visual sensations in a person | |
| Illumination | E | luxury | OK | The ratio of the luminous flux incident on a small area of a surface to its area. | |
| Luminosity | M | lumen per square meter | lm/m2 | Luminous quantity representing luminous flux | |
| Brightness | L,B | candela per square meter | cd/m2 | Luminous intensity emitted per unit surface area in a specific direction | |
| Radiation energy | E,W | joule | J = (kg m2/s2) | Energy transferred by optical radiation |
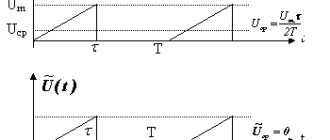
Voltage determination
When performing electrical installation work, a specialist is faced with different types of voltage. For example, sockets in apartments and private houses are sources of alternating voltage. It can be lowered or raised by a transformer, or rectified by a special device. Friction stress is measured in laboratory conditions using the electrochemical method. The technician needs to know about the features of measuring different types of voltage.
Constant pressure
It can be measured using magnetoelectric devices. Nowadays you can find high-precision instruments equipped with a digital display on sale. The easiest way is to directly connect the device to the area where you want to measure. In this case, the following rules must be observed:
- The limit value must exceed the intended maximum. In the case when measuring work is carried out without knowledge of this parameter, it is necessary to set a maximum limit and gradually reduce it.
- Pay attention to the polarity of the connection. Otherwise, the pointer on a dial instrument will tilt in the opposite direction, and on a digital instrument, a negative number will appear on the screen.
Laboratory voltmeter
AC voltage
In this case, different types of measuring instruments are used, with the exception of magnetoelectric ones. They work with such devices only by connecting to the output of a rectifier.
Table of units of measurement "Acoustics"
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Sound pressure | p | pascal | Pa | Variable excess pressure arising in an elastic medium when a sound wave passes through it | |
| Volume velocity | c, V | cubic meter per second | m3/s | The ratio of the volume of raw materials supplied to the reactor per hour to the volume of catalyst | |
| Sound speed | v, u | meter per second | m/s | Velocity of propagation of elastic waves in a medium | |
| Sound intensity | l | watt per square meter | W/m2 | A quantity characterizing the power transferred by a sound wave in the direction of propagation | scalar physical quantity |
| Acoustic impedance | Za, Ra | pascal second per cubic meter | Pa•s/m3 | The ratio of the amplitude of sound pressure in a medium to the vibrational speed of its particles when a sound wave passes through the medium | |
| Mechanical resistance | Rm | newton second per meter | N•s/m | Indicates the force required to move a body at each frequency |
Table of units of measurement “Atomic and nuclear physics. Radioactivity"
| Physical quantity | Symbol | Unit of measurement of physical quantity | Unit change physical led | Description | Notes |
| Mass (rest mass) | m | kilogram | kg | The mass of an object at rest. | |
| Mass defect | Δ | kilogram | kg | A quantity expressing the influence of internal interactions on the mass of a composite particle | |
| Elementary electric charge | e | pendant | Cl | The minimum portion (quantum) of electric charge observed in nature in free long-lived particles | |
| Communication energy | Est | joule | J = (kg m2/s2) | The difference between the energy of a state in which the constituent parts of the system are infinitely distant | |
| Half-life, average lifetime | T, τ | second | With | The time during which the system decays in the approximate ratio of 1/2 | |
| Effective cross section | σ | square meter | m2 | A quantity characterizing the probability of interaction of an elementary particle with an atomic nucleus or another particle | |
| Nuclide activity | A | becquerel | Bk | A value equal to the ratio of the total number of decays of radioactive nuclide nuclei in the source to the decay time | |
| Energy of ionizing radiation | E,W | joule | J = (kg m2/s2) | Type of energy released by atoms in the form of electromagnetic waves (gamma or x-rays) or particles | |
| Absorbed dose of ionizing radiation | D | gray | Gr | The dose at which 1 joule of ionizing radiation energy is transferred to a mass of 1 kg | |
| Equivalent dose of ionizing radiation | H , Deck | sievert | Sv | Absorbed dose of any ionizing radiation equal to 100 erg per 1 gram of irradiated substance | |
| Exposure dose of X-ray and gamma radiation | X | pendant per kilogram | C/kg | ratio of the total electric charge of ions of the same sign from external gamma radiation |
Permissible doses of radiation
-
0.57 μSv/hour for five consecutive years - the maximum permissible total annual dose received from all technogenic sources is
1 mSv/year
In subsequent years, background radiation should not exceed 0.12 μSv/hour
The value of 1 mSv/year should in total include all episodes of man-made exposure to radiation on humans. This includes all types of medical examinations and procedures, including fluorography, dental x-rays, and so on. This also includes flying on airplanes, going through security at the airport, obtaining radioactive isotopes from food, and so on.