We often hear the word resonance: “public resonance”, “event that caused resonance”, “resonant frequency”. Quite familiar and ordinary phrases. But can you say exactly what resonance is?
If the answer jumped out at you, we are truly proud of you! Well, if the topic “resonance in physics” raises questions, then we advise you to read our article, where we will talk in detail, clearly and briefly about such a phenomenon as resonance.
Before talking about resonance, you need to understand what oscillations are and their frequency.
The phenomenon of resonance in life and technology.
The phenomenon of resonance can play both a positive and negative role.
It is known, for example, that even a child can swing the heavy “tongue” of a large bell, but only if he pulls the rope in time with the free vibrations of the “tongue.”
The action of a reed frequency meter is based on the use of resonance. This device is a set of elastic plates of various lengths reinforced on a common base. The natural frequency of each plate is known. When the frequency meter comes into contact with an oscillatory system, the frequency of which needs to be determined, the plate whose frequency coincides with the measured frequency begins to vibrate with the greatest amplitude. By noticing which plate has entered resonance, we will determine the oscillation frequency of the system.
The phenomenon of resonance can also be encountered when it is completely undesirable. For example, in 1750, near the city of Angers in France, a detachment of soldiers walked in step across a 102 m long chain bridge. The frequency of their steps coincided with the frequency of free vibrations of the bridge. Because of this, the vibration range of the bridge increased sharply (resonance occurred), and the chains broke. The bridge collapsed into the river.
In 1830, a suspension bridge near Manchester in England collapsed for the same reason while a military detachment was marching across it.
In 1906, the Egyptian Bridge in St. Petersburg, across which a cavalry squadron was passing, collapsed due to resonance.
Now, to prevent such cases, military units, when crossing the bridge, are ordered to “knock their feet”, to walk not in formation, but at a free pace.
If a train passes through a bridge, then, in order to avoid resonance, it passes it either at a slow speed, or, conversely, at maximum speed (so that the frequency of the wheels hitting the rail joints does not turn out to be equal to the natural frequency of the bridge).
The car itself (oscillating on its springs) also has its own frequency. When the frequency of impacts of its wheels at the rail joints turns out to be equal to it, the car begins to sway violently.
Resonance phenomenon
The phenomena of resonance are associated with the periodic oscillatory movement of electrons in a circuit and consist in the fact that electrons in a given oscillatory circuit most easily “swing” with a certain frequency, which we call resonant. We encounter periodic oscillatory motion everywhere. The oscillation of a pendulum, the vibration of a string, the movement of a swing are all examples of oscillatory motion.
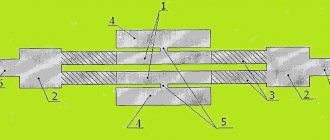
For example, consider the oscillatory system shown in Figure 1. This system, as we will see later, has much in common with an electrical oscillatory circuit. It consists of a spring and a massive ball attached to a rod.
Figure 1. Mechanical model of an oscillatory circuit. Mass-inductance, flexibility-capacitance, friction-resistance.
If we pull the ball down from its equilibrium position, then under the action of the spring it will immediately rush back; however, having acquired a certain speed, the ball will not stop at the equilibrium point, but by inertia will jump further, which will cause new deformation (compression) of the spring. Then this process will be repeated in the opposite direction, etc. The ball will oscillate in one direction and the other until the entire supply of energy imparted to the spring when the ball is deflected is spent on friction.
It is easy to notice that when the ball oscillates, the energy imparted to the system constantly transfers from the energy of deformation (compression and extension) of the spring into the energy of motion of the ball and back. In mechanics, the first type of energy is called potential energy, and the second type is called kinetic energy.
While the ball is in one of its extreme positions, it stops for a moment. At this moment, the energy of its movement is zero. But the spring at this moment is very strongly deformed: either compressed or stretched; it therefore contains the greatest amount of energy. At the same moment when the ball passes through the equilibrium position with the greatest speed, it has the greatest energy, but the energy of the spring at this moment is zero, since it is not compressed or stretched.
By deflecting the ball at various distances and observing each time the frequency of subsequent free oscillations of the system, we will notice that the frequency of oscillations of the system remains the same all the time. In other words, it does not depend on the magnitude of the initial deviation. We will call this frequency the natural frequency of the system.
If we had at our disposal not one such system, but several, then we could be convinced that the natural frequency of free oscillations of the system decreases with increasing mass of the ball and increases with increasing elasticity, i.e., with decreasing flexibility of the spring. This dependence can also be detected using a simpler example with vibrating strings of various thicknesses and varying degrees of tension.
If we want to swing the ball with the least amount of effort, then we will, of course, try, firstly, to establish a strict periodicity of our pushes, that is, we will try to ensure that the pushes follow each other after a certain time, and secondly, we will try, so that the time interval between shocks is equal to the period of natural oscillations of the system (Figure 2).
Figure 2. Mechanical model of an oscillatory circuit with undamped oscillations. The frequency of the forced force is equal to the natural frequency of the system (resonance).
In order to swing the oscillatory system with the least amount of effort, it is necessary to make the frequency of the driving force equal to the natural frequency of oscillation of the system. This rule is very well known to all of us since childhood, when we used it while swinging on a swing.
Figure 3. The phenomenon of resonance using the example of a swing.
So, when the frequency of the driving force coincides with the natural frequency of oscillations of the system, the amplitude of the oscillations becomes greatest.
Thus, it must be said that the coincidence of the frequency of the driving force with the natural frequency of oscillations of the system is resonance .
You don't have to look far for examples of resonance. Window glass that shakes with a certain frequency every time a tram or truck passes by; the trembling of the string of a musical instrument after we touch an adjacent string tuned in unison with the first, etc. - all these are resonance phenomena.
Let's charge the capacitor with some amount of electricity (Fig. 4, a) and then close it to the inductor (Fig. 4, b). The capacitor will begin to discharge immediately. A discharge current will flow through the inductor, and the appearance of current in the coil will lead to the appearance of a magnetic field around it. In this case, a self-induction emf will arise in the coil, which will delay the discharge of the capacitor. When the capacitor is discharged, the current in the coil will not stop, since it will now be supported by the self-induction emf due to the energy stored in the magnetic field of the coil during the discharge of the capacitor. This continuing current will recharge the capacitor in the opposite direction, that is, the plate that was previously positive will become negative, and vice versa (Fig. 4, c).
Figure 4. Free vibrations. At the top - electrical, at the bottom - mechanical.
After this, the capacitor will again begin to discharge, recharge again (Fig. 4, d, e), etc. The current oscillations in the circuit will continue until all the electrical energy imparted to the circuit when charging the capacitor is converted into thermal energy. This will happen the sooner, the greater the active resistance of the circuit.
So, the discharge of a capacitor through an inductor is an oscillatory process. During this process, the capacitor is charged and discharged several times, energy alternately transfers from the electric field of the capacitor to the magnetic field of the coil and vice versa.
Figure 5. Oscillations in an oscillatory circuit.
The current fluctuations that occur during this discharge are of a damped nature (Fig. 6).
Figure 6. Damped oscillations in a circuit.
The oscillation frequency for the selected values of capacitance and inductance is a completely definite value and is called the natural frequency of the circuit. The circuit's natural frequency will be greater, the smaller the capacitance and inductance of the circuit.
If an alternating current source is introduced into the oscillatory circuit, the frequency of which coincides with the natural frequency of the circuit, then the oscillations in the circuit will reach the greatest value, i.e., the phenomenon of resonance will occur.
A far-reaching parallel can be drawn between electrical and mechanical vibrations.
In table
1 on the left are electrical quantities and phenomena, and on the right are similar quantities and phenomena from the field of mechanics in relation to our mechanical model of the oscillatory circuit. Analogy of electrical and mechanical quantities
| Electrical quantities | Mechanical quantities |
| Oscillatory circuit inductance | Ball mass; |
| Oscillatory circuit capacitance | Spring flexibility |
| Loop resistance | Mechanical friction |
| Capacitor plates | Springs |
| Capacitor charge | Deformation (compression and extension) of springs |
| Positive plate charge | Spring compression |
| Negative plate charge | Spring stretch |
| Current strength | Ball speed |
| Current direction | Ball movement direction |
| Electromotive force of self-induction | Ball inertia force |
| Amplitude (largest instantaneous current value) | Amplitude (the largest deviation of the ball from its equilibrium position) |
| Frequency (cycles per second) | Frequency (number of oscillations per second) |
| Resonance (coincidence of the frequency of the external EMF with the natural frequency of the horn) | Resonance (coincidence of the frequency of the driving force shocks with the natural frequency of oscillations of the ball) |
The various moments of electrical oscillation and the corresponding moments of oscillation of our mechanical model of the oscillatory circuit are depicted in Fig. 4.
DID YOU LIKE THE ARTICLE? SHARE WITH YOUR FRIENDS ON SOCIAL NETWORKS!
Related materials:
- Coil inductive reactance
- Inductor in an AC circuit
- Capacitor in an alternating current circuit. Capacitance of a capacitor.
- AC circuit resistance
- AC circuit impedance
- Ohm's law for alternating current
- Voltage resonance in a series oscillating circuit
- Resonance of currents in a parallel oscillatory circuit
- Pulsating current
- Non-sinusoidal current
Add a comment
Under what conditions does it occur?
The condition for this phenomenon to occur is equal conductor frequencies, where BL=BC. That is, capacitive and inductive conductivity must be equal. Only then is a similar phenomenon of current resonance observed in an electrical circuit. It can be either positive or negative. Any radio receiver has an oscillating circuit, which, due to inductive or capacitive changes, is tuned to the desired radio wave signal. In another case, this leads to voltage surges or current in the circuit and an emergency situation occurs.
In laboratory conditions, it occurs at a time when the capacitance changes and the inductance of the coil L does not change. In this case, the formula looks like Bc=C
Under what conditions does it occur?
Physics Glossary
|
Resonance. resonance
- (French resonance, from Latin resono - I respond) - a frequency-selective response of an oscillatory system to a periodic external influence, during which a sharp increase in the amplitude of stationary oscillations occurs. It is observed when the frequency of external influence approaches certain values characteristic of a given system. In linear oscillatory systems, the number of such resonant frequencies corresponds to the number of degrees of freedom, and they coincide with the frequencies of natural oscillations. In nonlinear oscillatory systems, the reactive and dissipative parameters of which depend on the magnitude of the external influence, resonance can manifest itself both as a response to an external force action and as a reaction to a periodic change in parameters. In a strict sense, the term “resonance” refers only to the case of force.
Resonance in linear systems with one degree of freedom
An example of the simplest case of resonance is represented by forced oscillations excited by an external source - a harmonic emf. ~ E0cospt with amplitude E0 and frequency p
- in an oscillatory circuit (Fig. 1, a).
Rice. 1. Oscillatory systems with one degree of freedom: serial (a) and parallel (b) oscillatory circuits, mathematical pendulum (c) and elastic oscillator (d),
Amplitude x and phase φ of forced oscillations of the charge [q(t) = xcos( p
t + φ)] are determined by the amplitude and frequency of the external force:
where F = E0/L, d = (R + Ri)/2L.
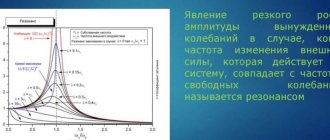
Dependence of amplitude x of stationary forced oscillations on frequency p
the driving force at a constant amplitude is called a resonance curve (Fig. 2).
In a linear oscillatory circuit, the resonance curves corresponding to different F are similar, and the phase-frequency characteristic f( p
) does not depend on the force amplitude.
The investment of energy into the oscillatory circuit is proportional to the first power, and the dissipation of energy is proportional to the square of the oscillation amplitude. This ensures limitation of the amplitudes of stationary forced oscillations at resonance. Frequency approximation p
to the natural frequency w0 is accompanied by an increase in the amplitude of forced oscillations, the more sharply, the lower the damping coefficient
d
.
At resonance, the current flowing through the circuit, I = p
xcos(
p
t + φ -
p
/2), is in phase with the emf.
third-party source (f = p
/2). A decrease in the amplitude of forced oscillations during inaccurate tuning is due to a violation of the in-phase current and voltage in the circuit.
An important characteristic of the resonant properties of an oscillatory system (oscillator) is the quality factor Q, which, by definition, is equal to p
the ratio of the energy stored in the system to the energy dissipated during the oscillation period. When exposed to a resonant frequency, the amplitude of forced oscillations x is Q-times greater than in the quasi-static case, at . The number of oscillation periods during which a stationary amplitude is established is also proportional to Q. Finally, the quality factor determines the frequency selectivity of resonant systems. The resonance bandwidth Dw, within which the amplitude of forced oscillations decreases by a factor of x, is inversely proportional to the quality factor: Dw = w0/Q = 2d.
When there is resonance in electrical circuits, the reactive part of the complex impedance goes to zero. In this case, in a series circuit, the voltage drops on the inductor and on the capacitor have an amplitude of QE0. However, they add up in antiphase and cancel each other out. In a parallel circuit (Fig. 1, b) at resonance, mutual compensation of currents in the capacitive and inductive branches occurs. Unlike series resonance, in which the external force action is carried out by a voltage source, in a parallel circuit resonance phenomena are realized only when the external force is set by a current source. Accordingly, resonance in a series circuit is called voltage resonance, and in a parallel circuit - current resonance. If a voltage generator is included in a parallel circuit instead of a current generator, then at the resonant frequency the conditions of not a maximum, but a minimum current will be met, since due to the compensation of currents in the branches containing reactive elements, the conductivity of the circuit turns out to be minimal (anti-resonance phenomenon).
The phenomenon of resonance in mechanical and other oscillatory systems has similar features. In linear systems, according to the principle of superposition, the system's response to a periodic non-sinusoidal impact can be found as the sum of responses to each of the harmonic components of the impact. If the period of the non-sinusoidal force is equal to T, then the resonant increase in oscillations can occur not only under the condition w0! 2p _
/T, but depending on the shape of E(t) and under the conditions w0 !
2 p
n/T, where n = 1, 2,… (harmonic resonance).
Resonance curves are determined by observing a change in the amplitude of forced oscillations or by slowly tuning the frequency p
driving force, or with a slow change in natural frequency w0.
With a high quality factor of the oscillator (Q1), both methods give almost identical results. The frequency characteristics obtained at a finite rate of frequency change differ from static resonance curves corresponding to infinitely slow tuning: in the dynamic frequency characteristics, a shift of the maximum in the direction of frequency tuning is observed, proportional to. m, where is the relaxation time of oscillations in the circuit, t* is the time during which the frequency p
is within the resonance band Dw. With rapid frequency tuning, as m increases, the height decreases and the resonance curves expand, and their shape becomes more asymmetrical (Fig. 3).
Rice. 3. Static and dynamic amplitude-frequency characteristics of resonance at different rates of frequency increase: p
(t)= w0 + t/m, m = 0(1), 0.0625 (g), 0.25(3), 0.695 (4).
Resonance in linear oscillatory systems with several degrees of freedom
Oscillatory systems with several degrees of freedom are a set of interacting oscillators. An example is a pair of oscillatory circuits connected through mutual induction (Fig. 4). Forced oscillations in such a system are described by the equations
Inductive coupling means that oscillations in individual circuits cannot occur independently of each other. However, for any oscillatory system with several degrees of freedom, one can find normal coordinates, which are linear combinations of independent variables. For normal coordinates, a system of equations similar to (2) is transformed into a chain of equations for forced oscillations of the same type as for single oscillatory circuits, with the difference that each of the normal coordinates is affected by forces applied, generally speaking, in different parts total oscillatory system. When considering the laws of motion in normal coordinates, all the laws of resonance in systems with one degree of freedom are valid.
Rice. 4. An oscillatory system with two degrees of freedom - a pair of circuits with coupling due to mutual induction.
The resonant increase in oscillations occurs in all parts of the oscillatory system at the same frequencies (Fig. 5), equal to the natural frequencies of the system. Normal frequencies do not coincide with partial ones, i.e. with their own. frequencies of the oscillators included in the overall system. If the frequency of the external force is equal to one of the partial frequencies, then resonance does not occur in the overall system. On the contrary, in this case the amplitudes of forced oscillations reach a minimum, similar to the case of antiresonance in a system with one degree of freedom. The ability to suppress oscillations, the frequency of which is equal to one of the partial ones, is used in electrical applications. filters and mechanical dampers. hesitation.
| Rice. 6 . Resonance curves of a double-circuit oscillatory system with gQ = 1(1), and 2(3); g = M/L, L1 = L2. |
In a system consisting of weakly coupled oscillators with identical partial frequencies, resonant maxima corresponding to close normal frequencies can merge, so that the frequency response has one maximum (Fig. 6). Increasing the coupling between oscillators leads to an increase in the interval between the normal frequencies of the system. The change in the shape of the resonance curves with increasing coupling coefficient is illustrated in Fig. 6. A system of oscillators with coupling close to critical has a frequency response that is flattened near resonance, and the steepness of its slopes is higher than that of a single oscillator with the same level of losses. This property is commonly used to create bandpass electrical filters.
Resonance in distributed oscillatory systems
In distributed systems (see System with distributed parameters), the amplitude and phase of oscillations depend on spatial coordinates. Linear distributed oscillatory systems are characterized by a set of normal frequencies and eigenfunctions that describe the spatial distribution of the amplitudes of natural oscillations. The resonant properties (quality factor) of distributed systems are determined not only by their own. attenuation, but also connection with the environment into which part of the oscillation energy (electrical, elastic and others) is emitted. In distributed systems with a high quality factor (Q1), forced oscillations are standing waves, the spatial distribution of amplitudes of which is a superposition of eigenfunctions (modes), and the phase of oscillations is the same at all points. The action of external forces with frequencies close to their own leads to a resonant increase in the amplitude of forced oscillations at all points in the volume of a distributed resonant system (resonator).
In distributed systems, all general properties of resonance remain valid. A feature of resonance in distributed systems (as well as in systems with several degrees of freedom) is the dependence of the amplitudes of forced oscillations not only on frequency, but also on the spatial distribution of the driving force. Resonance occurs if the spatial distribution of external force repeats the shape of its own. functions, and the frequency is equal to the corresponding normal frequency. If the spatial distribution of the external force is unfavorable, forced oscillations are not excited. This occurs, in particular, when a concentrated force is applied at points for which the amplitude of the corresponding normal vibration vanishes. Thus, by applying a concentrated force at a point that is a nodal point for the movement of the string, it is impossible to excite its oscillations, since the work done by the force will be zero. If the distribution of forces is such that the work done by them in different parts of the system has opposite signs and generally does not lead to a change in energy, forced oscillations are also not excited.
Resonance in nonlinear oscillatory systems
In elastic systems, the nonlinear element is a spring, for which the relationship between deformation and elastic force is nonlinear, i.e., Hooke’s law is violated. In electric systems, an example of a nonlinear dissipative element is a diode, the current-voltage characteristic of which does not obey Ohm’s law. Nonlinear reactive (energy-intensive) elements are capacitors with ferroelectrics or inductors with ferrite cores. The parameters of these elements are capacitance, inductance, resistance, as well as their own. frequency and coefficient damping in nonlinear systems can be considered functions of current or voltage. At the same time, in nonlinear systems the superposition principle does not apply.
In nonlinear systems, harmonic. force excites inharmonious. vibrations, in the spectrum of which there are multiple frequencies, so resonance at harmonics occurs p with a sinusoidal external. strength. In oscillation systems with a sufficiently high quality factor and frequency selectivity, max. The amplitude is that spectral component whose frequency is close to the resonance frequency. Considering only oscillations with a frequency close to the resonance one, in this case it is possible to obtain a family of resonance curves. For a system with nonlinear reactive (energy-intensive) elements at r! w0 these curves are shown in Fig. 7. The shape of the resonance curve depends on the amplitude of the driving force and, as it increases, it becomes more and more asymmetrical. Since the natural frequency Since the oscillations of a nonlinear oscillator depend on their amplitude, the maxima on the resonance curves shift towards higher or lower frequencies. Starting from a certain value of the force amplitude, the resonance curves acquire an ambiguous beak-shaped shape. In a certain frequency range, the stationary amplitude of forced oscillations turns out to depend on the history of the establishment of oscillations (the phenomenon of oscillation hysteresis). In this case, parts of the resonance curves corresponding to unstable states form a region of physically unrealizable regimes on the plane (x, p) (shaded in Fig. 7).
Rice. 7 . A family of amplitude-frequency curves in the case of nonlinear resonance at various amplitudes of the external force (F1 < F2 < < F3 < F4). The dotted line is an unstable section of the resonance curve. The region of unstable states is shaded. The arrows mark the points of abrupt changes in the amplitudes of oscillations when the frequency is tuned up (AB) and down (CD).
On the phenomenon of nonlinear resonance in widespread oscillations. systems can render creatures. influence of the effects of self-focusing and the formation of shock waves, especially in cases where a large number of waves fit along the length of the resonator.
Phenomena related to resonance
In nonlinear oscillations. external systems periodic the impact causes not only the excitation of forced oscillations, but also the modulation of energy-intensive and dissipative parameters. The phenomenon of excitation of oscillations during periodic modulation of energy-intensive parameters is called parametric resonance.
If the modulation depth of an energy-intensive parameter is insufficient to excite parametric resonance, in oscillation. the system partially compensates for losses. Resonant response to a weak signal with frequency p
!
w0 is the same as that of a linear oscillator with a higher quality factor. In addition, combination fluctuations are formed. frequencies m p
+ nwМ, where wМ is the modulation frequency of the parameter. When the frequency
p
and (wМ - p) coincide, forced oscillations in a parametrically regenerated system depend on the relationships between the phases of the parametric. influence and weak strength (signal). In this case, both an increase and a decrease in the amplitude of forced oscillations can occur compared to the absence of parametric parameters. regeneration (phenomena of “strong” and “weak” resonance).
The effect of loss regeneration and increasing the equivalent quality factor occurs in resonant systems with nonlinear losses, which contain elements with negative differential resistance or positive feedback circuits. Such systems are called potentially self-oscillating. If a potentially self-oscillating system is affected by a periodic force of significant amplitude with frequency p
, it can influence the damping of oscillations in the system so that during a certain fraction of the period of action of the damping force it becomes negative.
The result is potentially self-oscillation. the system is excited by oscillations at a frequency w close to its own, if the additional condition w = p
/n is satisfied. The case n = 1 corresponds to synchronization of the external self-oscillation frequency. by force. At n2 this phenomenon is called autoparametric excitation, by analogy with parametric resonance, in contrast to which with autoparametric. During excitation, modulation occurs not of the energy-intensive, but of the dissipative parameters of the system.
The term “resonance” is also used in relation to processes in quantum systems, when the frequency is external. influence (radiation) is equal to the frequency of the quantum transition, so the condition is satisfied
where is the energy of the n- and m-th levels of the quantum system, respectively. When (3) is fulfilled, the probabilities of quantum transitions increase sharply, which manifests itself as an increase in the intensity of energy exchange - absorption and emission (see Quantum electronics, Laser).
Resonance can cause instability and mechanical failure. engineering structures and electrical networks. In vibration transducers, resonance makes it possible to achieve amplitudes of elastic vibrations due to periodic action of a relatively weak force. In radiophysics and radio engineering, the phenomenon of resonance underlies many methods for filtering signals of different frequencies, detecting and receiving weak signals.
Literature on the phenomenon of resonance
- Gorelik G.S., Oscillations and waves, 2nd ed., M., 1959;
- Strelkov S.P., Introduction to the theory of oscillations, 2nd ed., M., 1964;
- Kharkevich A. A. Selected works, vol. 2, M., 1973;
- Fundamentals of the theory of oscillations, ed. V.V. Migulina, 2nd ed., M., 1988.
G. V. Belokopytov.
to the library to the table of contents FAQ on ethereal physics TOEE CHPP TPOI
Did you know,
that “dark matter” is as fictional as a black cat in a dark room. This is not physical reality, but a trick, a substitution. In reality, we are talking about the fact that relativistic formulas do not correspond to astronomical observations, giving an order of magnitude less mass and less energy. From here the conjuring conclusion was made that there is “dark matter” and “dark energy”, but not the conclusion that relativistic formulas do not correspond to reality. Read more in the FAQ on ethereal physics.
"Singing" stone
Not far from Baku, the capital of Azerbaijan, there is a desert with the famous “singing” stone. It is so famous that it received the name “Stone Tambourine”. This amazing block has the property that if you hit it with a stone, the sound will be as loud and clear as a bell. How does physics explain this example of sound resonance?
The impact leads to short-term deformation - immediately sound waves run in all directions from the point of collision. The size of the stone does not affect the speed of their divergence. However, the wave can propagate freely only in unlimited space. But we know that stone and air have boundaries (where they touch).
Types of resonance
There are a large number of types of resonance in physics. They are all similar in some ways and different in some ways, namely in their characteristics and the nature of their appearance. Among them are:
- mechanical and acoustic resonances;
- electric;
- optic;
- orbital fluctuations;
- atomic, particle and molecular.
Process graph in an oscillatory circuit
The following subsections will describe each of these types in more detail.
Mechanical and acoustic
The most popular and obvious mechanical type would be the resonant swing, which was mentioned earlier. If you push them at certain moments, taking into account their frequency, the scope of their movement will increase or die out if no force is applied.
Mechanical resonators are based on the conversion of potential energy into kinetic energy and vice versa. If we consider a pendulum, then all its energy is potential at rest. It converts to kinetic when he passes the bottom point at his maximum speed.
Devices for organizing resonance
Important! Some mechanical systems are capable of storing potential energy and using it in various forms. An example is a spring that stores compression, which is the binding energy of atoms
The acoustic type of resonance can be found in some musical instruments such as guitar, violin, and piano. They have a fundamental resonant frequency that depends on the length, mass and tension of the strings.
Acoustic resonance helps people find defects in pipelines
In addition to the fundamental frequency, the strings of these musical instruments have resonance at higher harmonic vibrations of the fundamental frequency. If a string is pulled, it will begin to vibrate at all frequencies that are inherent in a given impulse, but frequencies that do not coincide with the resonance will very quickly die out, and the human ear will hear only harmonic vibrations, which are notes.
Acoustic systems, microphones and loudspeakers do not tolerate resonance of individual parts of their housing, as this reduces the uniformity of their amplitude-frequency characteristics and degrades the quality of sound reproduction.
Strings create acoustic resonance
Resonance electrical
There is also resonance in electronics. It refers to the state or mode of a passive electrical circuit containing coils and capacitors, in which its input reactance and conductivity will be zero. This means that at resonance, the current at the input to the circuit, if any, will be in phase with the voltage.
Oscillatory circuitIn electricity, resonance is achieved when induction and reaction capacitance are balanced. This equality allows energy to circulate between the inductive elements and their magnetic field, and the electric field in the capacitor.
The resonance mechanism itself is based on the fact that the MF of the inductance creates an electric current, which charges the capacitor, discharging it and creating this magnetic field. The simplest device based on this interaction is an oscillatory circuit capable of producing resonance of voltages and currents.
Light optical resonance model
Optical resonance
And there is resonance in the optical range. One of its most popular examples is the Fabry-Perot resonator. It is formed by several mirrors, between which a so-called resonating standing wave is established. In addition, ring resonating systems with a traveling wave and microscopic resonators with standing waves are used.
Oscillatory circuit diagram
Orbital wobble
Oscillations in astrophysics are situations where there are two or more celestial objects that have certain orbital periods that correlate like small natural numbers. As a result of this effect, celestial objects exert a constant gravitational attraction on each other. It stabilizes their orbits.
There are also fluctuations in the orbits of celestial bodies.
In pores and frameworks
Porous media, such as zeolites, are today actively used in various fields of chemistry, especially in heterogeneous catalysis. In the last two decades, the minds of researchers have been occupied by a new type of porous media - metal-organic
(MOC), capable of holding atoms and molecules of various substances within its structure.
MOFs can be easily prepared from coordination compounds that are formed by self-assembly of metal ions and organic ligands. This class of materials has broad capabilities for “fine-tuning” the structure and functions of the framework for a specific task by changing the pore size and structure of the active metal center. These potential “materials of the future” can be used to bind and store carbon dioxide and other gases, capture harmful impurities from air and water, convert solar energy, and even “targeted” delivery of drugs in the body.
From this point of view, the IOC design looks extremely attractive, but its implementation is not so simple. The most difficult thing is to determine how certain changes in the process of “tuning” the MOF will affect its properties.
It is especially difficult to take into account the influence of the degree of mobility (“flexibility”) of the structural elements of the framework on its ability to absorb various molecules. Imagine that we are designing a garage for a vehicle and choosing the dimensions of the beams and gates in such a way that it is convenient to park the car. And suddenly it turns out that depending on the air temperature, the size of the doorway can be reduced by almost half. Of course, in this case, we absolutely need to know exactly how the size of the opening depends on external conditions. Similar situations often occur in practical applications of flexible MOFs.
Most MOFs are diamagnetic; therefore, as in the case of ILs, when studying them using ESR, it becomes necessary to use paramagnetic spin probes. They can be molecules that are able to fit inside the framework structure without introducing significant disturbances into it (for example, an organic radical or a paramagnetic metal ion).
Recently, the EPR spectroscopy laboratory developed a method for introducing a paramagnetic spin probe into the cavity of a flexible ZIF-8 MOF. This frame, widely known among specialists, consists of large cavities connected by smaller “windows”, and thanks to a set of unique properties, it is a promising material for the sorption and separation of various substances. An example is the separation of a mixture of propane and propylene, which is an important technological task, since propylene is used to produce widely demanded polyethylene. The high efficiency of this separation is ensured by fine-tuning the size of the ZIF-8 cavity windows. However, little was known about the actual “effective” size of these cavity windows until recently.
When analyzing the EPR spectra of the spin probe located inside the ZIF-8 cavity, it turned out that it is extremely sensitive to atmospheric oxygen filling the pores. By immersing the frame in liquid, you can observe how it is filled, and by varying the solvents, you can determine which of them fit into the cavities and which do not. In addition, based on data on the rate of filling of cavities, it is possible to estimate the rate of diffusion of molecules into the frame.
As a result, using a series of solvents, it was possible to determine the real size of the ZIF-8 cavity windows and, moreover, to find out that it depends quite strongly on temperature. At temperatures around 90 °C, the size of the windows increases sharply, which fundamentally changes the permeability of this MOF to molecules.
As a result, using the example of separating a mixture of three xylenes (an important technological problem, since one of the xylenes is the initial monomer for the production of polyethylene terephthalate (PET), a popular thermoplastic), an approach was developed that allows us to isolate each of the components of the mixture by varying the temperature of the sorbent.
In the case of MOFs, there are situations when one of its structural elements (metal ion or ligand) is itself paramagnetic. Then the EPR method can be applied to study its properties directly.
Thus, in the case of a paramagnetic ligand, it is possible to study the interactions of “guest” molecules with the framework itself, and of a paramagnetic metal - the geometry of its local environment in the framework, as well as identify and measure distances to neighboring atoms. This approach is equally effective both for studying changes in the structure of MOFs under external influences and for assessing the efficiency of adsorption of industrially important gases (hydrogen, carbon dioxide, etc.).
"Singing" stone
Not far from Baku, the capital of Azerbaijan, there is a desert with the famous “singing” stone. It is so famous that it received the name “Stone Tambourine”. This amazing block has the property that if you hit it with a stone, the sound will be as loud and clear as a bell. How does physics explain this example of sound resonance?
The impact leads to short-term deformation - immediately sound waves run in all directions from the point of collision. The size of the stone does not affect the speed of their divergence. However, the wave can propagate freely only in unlimited space. But we know that stone and air have boundaries (where they touch). When the wave reaches the boundary, it partially passes into another medium - from stone into air. The remaining part of the acoustic energy is reflected in the opposite direction.
Achieving Resonance Blur
To partially reduce or blur (soften) the resonance, one of the conditions for reducing the amplitude must be met. The effect of depreciation is to:
- reduce the quality factor of the CS;
- remove the coincidence or intersection of the frequency ranges of the CS and the oscillation frequencies of possible third-party disturbances.
There are many devices and design solutions that allow you to do this. The most successful include:
- insertion of conductors with a smaller cross-section into stranded wires of power lines;
- the use of shock absorbers in transport to reduce vibrations while driving;
- use of damper inserts in pipelines operating under high pressure;
- a ban on walking in step when moving across bridges in a column;
- to prevent buildings from swaying and entering into wind resonance, install “blowers” that supply air against the wind;
- supplying current pulses to a non-rigid part during turning.
One of the universal methods designed to blur resonance suggests using two connected elements. For elements, changes in rigidity occur according to two different laws: linear and nonlinear. A coiled spring and a pressed wire are connected together, which is the damping component of the elastic action.
Electron paramagnetic resonance
Karelian State Pedagogical University
Electron paramagnetic resonance
Completed:
553 gr. (2007)
Electron paramagnetic resonance (EPR), the resonant absorption of electromagnetic energy in the centimeter or millimeter wavelength range by substances containing paramagnetic particles. EPR is one of the radiospectroscopy methods. Paramagnetic particles can be atoms and molecules, usually with an odd number of electrons (for example, nitrogen and hydrogen atoms, NO molecules); free radicals (for example, CH3); ions with partially filled internal electron shells (for example, nones of transition elements); impurity atoms (for example, donors in semiconductors); conduction electrons in metals and semiconductors. In general, paramagnetic substances are substances whose molecules have non-zero magnetic moments.
EPR was discovered by Evgeniy Konstantinovich Zavoisky in 1944. Since 1922, a number of works have expressed ideas about the possibility of the existence of EPR. An attempt to experimentally detect EPR was made in the mid-30s. Dutch physicist K. Gorter and his colleagues. However, ESR could only be observed thanks to radio spectroscopic methods developed by Zavoisky.
The magnetic moment M of a paramagnetic sample consists of the magnetic moments µі of the paramagnetic particles included in it, M = ∑ µі, (from i = 1 to N) where N is the number of paramagnetic particles. In the absence of an external magnetic field H, the chaotic thermal motion of paramagnetic particles leads to the averaging of the total magnetic moment to zero (M = 0). If a sample is placed in a constant magnetic field H, the magnetic moments of the paramagnetic particles are oriented along the direction of the vector H, so a non-zero total magnetic moment arises, that is, the sample is magnetized. The higher the magnetic field strength, the more magnetized the sample is. In relatively weak magnetic fields, the magnitude of the induced magnetic moment M is proportional to the field strength: M = χН, where χ is the magnetic susceptibility (usually χ = 10-3–10-6). In paramagnetic materials, the magnetic moment M is oriented in the direction of the external field. The potential energy E of a paramagnetic sample is determined by the scalar product of the vectors M and H according to the formula E = -(M × H), which means that the energy of the paramagnetic material in a magnetic field decreases, since
E = -(M × H) < 0. Therefore, paramagnetic substances are drawn into the magnetic field.
Let us consider the magnetic properties of a free paramagnetic atom. According to the laws of quantum mechanics, the orbital mechanical momentum of an electron
pl = √ l (l+ 1)ћ,
where l is the orbital quantum number, ћ = h/(2π) = 1.0545 × 10-27 erg × s – Planck’s constant. Orbital magnetic moment of an electron
µі = √ l (l+ 1)β
where β = eћ/(2mc) = 9.274 × 10-21 erg/G – Bohr magneton. Here e is the charge of the electron, m is the mass of the electron, c is the speed of light in vacuum. The ratio of the magnetic moment to the mechanical moment of the electron, usually expressed in units of e/(2mc), is called the magnetomechanical ratio or g-factor. In the case of magnetism caused by the orbital motion of an electron, the value of the g-factor is gorb = 1.
The electron also has its own mechanical moment (spin) and, accordingly, its own magnetic moment. In the case of pure spin magnetism, the mechanical and magnetic moments of the electron
ps = √s(s + 1)ћ and µ s = 2√s(s + 1) β,
where s is the spin quantum number of the electron, equal to s = 1/2. In the system of units e/(2mc), the value of the g-factor of a free electron is gs = 2.
If a free atom contains several electrons, then their orbital and spin moments
fold up. In this case, the magnetic properties of the atom will be determined by the values of quantum numbers L and S, which characterize the total moments due to the orbital and spin motion of electrons in the atom, as well as the total quantum number J. For light atoms L = ∑li,
S =∑si, and the value J can take the values J = |L + S|, …, |L — S|. In this case, the magnetomechanical ratio can be calculated using Lande's formula:
g = 1 + [J(J + 1) + S(S + 1) - L(L + 1)]/2J(J + 1).
In the absence of a total spin momentum (S = 0), we obtain g = 1; when the total orbital momentum is zero (L = 0), the value g = 2; in other cases, intermediate values 1 < g < 2 are possible.
Different values of quantum numbers L, S and J, as a rule, correspond to different energy levels of the atom. The electronic states of atoms are also characterized by magnetic quantum numbers mL, mS and mJ, which determine the projections of the orbital, spin and total moments in a given direction (Fig. 1). Quantum numbers mL, mS and mJ can take the following sets of values: mL = L, L - 1, ..., -(L - 1), - L; mS = S, S - 1, ..., -(S - 1), - S; mJ = J, J - 1, ..., -(J - 1), - J. In a spherically symmetric atom there is no physically distinguished direction of the coordinate axes. Therefore, in the absence of an external magnetic field, the energy levels of the atom, characterized by different values of magnetic quantum numbers, coincide. It is customary to say that such energy levels are degenerate in the magnetic quantum number.
If the atom finds itself in an external magnetic field H0, then in the direction of the vector H0 it is possible to determine the projections of the orbital, spin and total moments of the electrons. In this case, the degeneracy in magnetic quantum numbers is removed - different energy levels correspond to different values of mL, mS and mJ. Experimentally, this manifests itself in the fact that the spectral lines of paramagnetic atoms in a magnetic field are split (Fig. 1). The splitting of energy levels in a magnetic field was discovered in 1896 by the Dutch physicist P. Zeeman. The Zeeman effect underlies the EPR phenomenon.
Rice. 1. a - splitting of the electron energy level depending on the magnetic field H0; b - dependence of the power P of microwave radiation transmitted through the paramagnetic sample on the strength of the external magnetic field. The value ∆Р is the resonant absorption of microwave radiation (EPR signal). The blue curve is the first derivative of the EPR signal.
PHENOMENON OF ELECTRON PARAMAGNETIC RESONANCE
The first EPR signal was obtained which studied certain salts of iron group ions. Using the original radio engineering method he developed for recording electromagnetic radiation in the meter range, Zavoisky discovered that if a weak alternating electromagnetic field is applied to a paramagnetic sample placed in a constant magnetic field, then at a certain ratio between the strength H0 of the constant magnetic field and the frequency v of the alternating field, absorption is observed electromagnetic field energy. The condition for observing this effect is the perpendicular orientation of the magnetic vector of the alternating field H1(t) with respect to the direction of the static field H0. The phenomenon of magnetic resonance can be explained within the framework of classical and quantum physics.
Its description within the framework of classical physics is as follows: in an external constant magnetic field H, the magnetic moment vector µ precesses around the direction of the magnetic field H with a frequency v determined by the relation
2γv = γН.
Here γ is the gyromagnetic ratio (the ratio of the magnetic moment to the mechanical one). The precession angle θ (the angle between the vectors H and µ) remains constant. If the system is placed in a magnetic field H1┴H, rotating around H with frequency v, then the projection of the vector µ onto the direction of the field H will change with frequency v1 = γH1/2γ. This is a change in the projection µ with frequency v1 under the influence of the radio frequency field H1 (Fig. 2)
Rice. 2
has a resonant character and determines the EPR. When studying EPR, a linearly polarized alternating magnetic field is usually used, which can be represented as the sum of two fields rotating in opposite directions with a frequency v. One of the components, coinciding in the direction of rotation with precession, causes a change in the projection of the magnetic moment µ onto H.
In the quantum mechanical interpretation of EPR, to clarify the physical picture of the EPR phenomenon, we consider how a constant magnetic field H0 and an alternating magnetic field H1(t) affect the energy levels of an isolated paramagnetic atom (or ion). As we have already noted, the magnetic properties of an atom are characterized by the value of the quantum number J - the resulting magnetic moment. In most chemical
and biological systems studied by the EPR method, the orbital magnetic moments of paramagnetic centers are, as a rule, either equal to zero or make virtually no contribution to the recorded EPR signals. Therefore, for the sake of simplicity, we will assume that the paramagnetic properties of the sample are determined by the total spin of the S atom.
In the absence of an external magnetic field, the energy of a free atom does not depend on the spin orientation. When the external magnetic field H0 is turned on, the energy level splits into 2S + 1 sublevels, corresponding to different projections of the total spin S in the direction of the H0 vector:
E(mS) = mSgβH0,
where the magnetic spin quantum number ms, which can take the values mS = S, S - 1, ... ..., -(S - 1), - S. In the simplest case of a paramagnetic center with one unpaired electron, spin S = 1/2. This spin value corresponds to two Zeeman energy levels with mS = + 1/2 and -1/2, separated by the interval ∆E = gβH0 (Fig. 1). If the energy of quanta of electromagnetic radiation with frequency v acting on a system of spins in an external magnetic field is equal to the energy difference between neighboring levels, that is, hv = gβH0, then such radiation will cause transitions between energy levels. In this case, an alternating electromagnetic field, which has a magnetic component H1(t) perpendicular to the static field H0, can induce transitions both from bottom to top and from top to bottom with equal probability. Such induced transitions are accompanied by a change in spin orientation. According to quantum mechanical selection rules, only such transitions are possible in which the value of the magnetic quantum number changes by ∆mS = ±1. Such transitions are called allowed. The transition from the lower level to the upper one is accompanied by the absorption of a quantum of electromagnetic radiation. The transition from the upper level to the lower one leads to the emission of a quantum with energy ∆E = gβH0.
In a state of thermodynamic equilibrium, the populations of the lower (N 1) and upper (N2) levels differ. According to the Boltzmann distribution,
N2 (-∆E)
— = exp ——
N2 (kT)
where N 1 and N2 are the number of spins with magnetic quantum numbers mS = –1/2 and +1/2, k is Boltzmann’s constant, T is the absolute temperature. Since the lower energy levels are more populated than the upper levels (N2/N1 < 1), electromagnetic radiation will more often induce bottom-up transitions (energy absorption) than top-down transitions (energy emission).
Therefore, in general, absorption of the energy of the electromagnetic field by the paramagnetic sample will be observed. This is the essence of the EPR phenomenon. The energy difference between neighboring Zeeman levels is small (∆E << kT), so the radiation frequency corresponds to the microwave or radio frequency range (λ = 3 cm at H0 = 3300 Oe).
So far, we have considered an idealized case - a system of isolated paramagnetic atoms that do not interact with each other or with their environment. Such an idealization is an extremely strong simplification, within the framework of which the experimentally observed resonant absorption of electromagnetic radiation cannot be fully explained. Indeed, as the sample absorbs the energy of the electromagnetic field, the difference in the population of energy levels will disappear. This means that the number of induced transitions from bottom to top (energy absorption) will decrease, and the number of transitions from top to bottom (emission) will increase. After the populations of the upper and lower levels become equal (N2 = N1), the number of absorbed quanta becomes equal to the number of emitted quanta. Therefore, in general, absorption of electromagnetic radiation energy should not be observed. In reality, however, the situation is different.
In order to understand why, under resonance conditions, a paramagnetic system absorbs the energy of an electromagnetic field, it is necessary to take into account the phenomenon of magnetic relaxation. The essence of this phenomenon is that paramagnetic particles can exchange energy with each other and interact with the atoms and molecules surrounding them. For example, in crystals, spins can transfer their energy to the crystal lattice, in liquids - to solvent molecules. In all cases, regardless of the state of aggregation of the substance, by analogy with crystals it is customary to say that spins interact with the lattice. In the broadest sense of the word, the term “lattice” refers to all the thermal degrees of freedom of a system to which the spins can quickly release the energy they absorb. Thanks to the fast non-radiative relaxation of spins in the system, the almost equilibrium ratio of the populations of Zeeman sublevels is restored, at which the population of the lower level is higher than the population of the upper level, N2/N1 = exp(-∆E/kT) < 1. Therefore, the number of induced transitions from bottom to top corresponding to absorption energy will always exceed the number of induced transitions from top to bottom, that is, resonant absorption of electromagnetic radiation energy will prevail over radiation.
EPR radio spectrometer design
The design of an EPR radiospectrometer is in many ways similar to that of a spectrophotometer for measuring optical absorption in the visible and ultraviolet parts of the spectrum. The radiation source in the radio spectrometer is a klystron, which is a radio tube that produces monochromatic radiation in the centimeter wavelength range. The spectrophotometer diaphragm in the radio spectrometer corresponds to an attenuator that allows you to dose the power incident on the sample. The sample cell in a radiospectrometer is located in a special block called a resonator. The resonator is a parallelepiped with a cylindrical or rectangular cavity in which the absorbing sample is located. The dimensions of the resonator are such that a standing wave is formed in it. The element missing from the optical spectrometer is an electromagnet, which creates a constant magnetic field necessary for splitting the energy levels of electrons.
Rice. 3
The radiation that passes through the sample being measured, in the radiospectrometer and in the spectrophotometer, hits the detector, then the detector signal is amplified and recorded on a recorder or computer. One more difference of the radio spectrometer should be noted. It lies in the fact that radio frequency radiation is transmitted from a source to a sample and then to a detector using special rectangular tubes called waveguides. The cross-sectional dimensions of the waveguides are determined by the wavelength of the transmitted radiation. This feature of the transmission of radio radiation through waveguides determines the fact that to record the EPR spectrum in a radio spectrometer, a constant radiation frequency is used, and the resonance condition is achieved by changing the magnetic field value.
Another important feature of the radio spectrometer is signal amplification by modulating it with a high-frequency alternating field. As a result of signal modulation, it differentiates and transforms the absorption line into its first derivative, which is an EPR signal.
MAIN CHARACTERISTICS OF EPR SPECTRA
Let us briefly consider some characteristics of ESR signals, which can provide important information about the nature and electronic structure of paramagnetic particles.
g — Factor
The position of the line in the EPR spectrum is characterized by the value of the g-factor. The resonant value of the magnetic field is inversely proportional to the g-factor Hres = hn/(gβ). Measuring the g-factor value provides important information about the source of the EPR signal. As mentioned above, for a free electron g = 2. Taking into account the correction due to the influence of fluctuations of the electron-positron vacuum, this value is g = 2.0023. In many important cases (organic free radicals, paramagnetic defects in crystal lattices, etc.), the values of g-factors differ from the pure spin value by no more than the second decimal place. However, this is not always the case. Paramagnetic particles studied by EPR are, as a rule, not free atoms. The influence of anisotropic electric fields surrounding atoms, splitting of Zeeman levels in a zero external magnetic field (see below) and other effects often lead to significant deviations of the g factor from the pure spin value and to its anisotropy (the dependence of the g factor on the orientation of the sample in the external magnetic field). Significant deviations of g factors from the purely spin value g = 2.0023, as we noted above, are observed in the presence of a sufficiently strong spin-orbit interaction.
Fine structure of EPR spectra
If the spin and orbital moments in an atom are non-zero, then due to the interaction of the spin and orbital moments (spin-orbit interaction), the energy levels can further split. As a result, the appearance of the EPR spectrum will become more complicated and instead of one spectral line, several lines will appear in the EPR spectrum. In this case, they say that the EPR spectrum
has a fine structure. In the presence of strong spin-orbit interaction, splitting of Zeeman levels can be observed even in the absence of an external magnetic field.
We will illustrate the appearance of fine structure using the EPR spectrum of chromium alum as an example. The Cr3+ ion has a total spin of 3/2 (three unpaired electrons), therefore, four values of the magnetic quantum number are possible: mS = 3/2, 1/2, -1/2 and -3/2. In chromium alum, strong spin-orbit coupling and electrical anisotropy of the crystal lattice lead to the fact that the energy level splits in zero field, and the energy level splits into two levels corresponding to the values mS = ±3/2 and ±1/2 (Fig. . 4). In a magnetic field, each of these levels is split into two
Rice. 4. Diagram of energy levels of Cr3+ ions, illustrating the appearance of the fine structure of the EPR spectrum
sublevel. Taking into account the selection rule for transitions between electronic Zeeman levels (∆mS = ± 1), we obtain the diagram of electronic transitions shown in Fig. 4. From that diagram it is clear that the resonance condition (∆E = gbH) will be satisfied at three different values of the magnetic field, due to which three resonance lines will appear in the EPR spectrum, that is, a fine structure of the EPR spectrum will appear
Ultrafine structure of EPR spectra
If, in addition to unpaired electrons, the paramagnetic sample under study contains atomic nuclei with their own magnetic moments (1H, 2D, 14N, 13C, etc.), then due to the interaction of electronic and nuclear magnetic moments, a hyperfine structure (HFS) of the spectrum appears.
Let us consider the emergence of HFS using the example of the interaction of an unpaired electron with a paramagnetic nitrogen nucleus (Fig. 5). This interaction is observed in the NO molecule, as well as in nitroxide radicals, which are widely used to study various biological systems. If the unpaired electron is localized near the nitrogen nucleus, then the magnetic field created by the magnetic moment µN of the nitrogen nucleus is added to the external magnetic field H0 acting on the electron. The nitrogen nucleus has spin I= 1, therefore three projections of the magnetic moment µN are possible: in the direction, perpendicular and against the external magnetic field H0. These orientations of the nuclear spin correspond to the values of the magnetic quantum number Iz = + 1, 0, -1. Therefore, due to the interaction
Rice. 5. Diagram of energy levels illustrating the appearance of the hyperfine structure of the EPR spectrum of the paramagnetic NO molecule
of an unpaired electron with a nitrogen nucleus, each of the Zeeman energy levels of the unpaired electron will split into three sublevels (Fig. 5). Transitions between energy levels induced by microwave radiation must satisfy quantum mechanical selection rules: ∆Sz = ± 1 (the orientation of the electron spin changes) and ∆Iz = 0 (the orientation of the nuclear spin is conserved). Thus, as a result of hyperfine interaction, three lines will appear in the EPR spectrum of the nitroxide radical, corresponding to three possible orientations of the magnetic moment of the nitrogen nucleus (Iz = -1, 0, +1)
Spectral line width
EPR signals are characterized by a certain spectral linewidth. This is due to the fact that
that the Zeeman energy levels between which resonant transitions occur are not infinitely narrow lines. If, due to the interaction of unpaired electrons with other paramagnetic particles and the lattice, these levels turn out to be blurred, then the resonance conditions can be realized not at one value of the field H0, but in a certain range of fields. The stronger the spin-spin and spin-lattice interactions, the wider the spectral line. In the theory of magnetic resonance, it is customary to characterize the interaction of spins with the lattice by the so-called spin-lattice relaxation time T1, and the interaction between spins by the spin-spin relaxation time T2. The width of a single EPR line is inversely proportional to these parameters:
∆H ~ Т1-1 ~ Т2-1
The relaxation times T1 and T2 depend on the nature of the paramagnetic centers, their environment and molecular mobility, and temperature.
The study of the shape of the EPR spectrum depending on various physicochemical factors is an important source of information about the nature and properties of paramagnetic centers. In Fig. Figure 6a shows a typical ESR spectrum of one of the stable nitrous oxide free radicals, which are often used in chemical and biophysical research. The shape of the EPR spectra of such radicals is sensitive to changes in their environment and mobility, so they are often used as molecular probes to study microviscosity and structural changes in various systems: solutions, polymers, biological membranes and macromolecular complexes. For example, from the temperature dependences of the intensity and width of the EPR spectra of spin probes, one can obtain important information about phase transitions in a system containing paramagnetic centers. In Fig. Figure 6b shows the temperature dependence of one of the parameters of the ESR spectrum (~Hmax is the distance between the extreme components of the HFS) for a nitrate radical dissolved in a multilayer liquid crystal film formed from distearophosphatidylcholine molecules. Such structures form the basis of biological membranes. It can be seen that the temperature dependence of the parameter ∆Нmax has a characteristic kink, from which it can be judged that at a temperature of 53°C a phase transition occurs in the system, accompanied by an increase in the mobility of nitrate radical molecules.
Rice. 6. a – ESR spectrum of a nitroxide radical dissolved in a multilayer film of distearophosphatidylcholine molecules. The shape of the EPR spectrum is determined by the molecular mobility and orientation of the nitroxide radical; b – dependence of the EPR spectrum parameter ∆ Нmax on temperature. A sharp decrease in the parameter ∆Hmax at a temperature of 53°C is due to an increase in the molecular mobility of the radical as a result of the phase transition of distearophosphatidylcholine molecules from a gel-like (“solid”) state to a liquid crystalline (“liquid”) state
The characteristics of the EPR spectra listed above - the g-factor, the fine and ultrafine structure of the EPR spectrum, the widths of individual components of the spectrum - are a kind of “passport” of a paramagnetic sample, by which one can identify the source of the EPR signal and determine its physicochemical properties. For example, by observing the ESR signals of biological objects, one can directly monitor the progress of intracellular processes in plant leaves, animal tissues and cells, and bacteria.
Bibliography
L. A Blumfeld, “Electron paramagnetic resonance”
Moscow State University . M. _ IN . Lomonosov
Wikipedia https://ru. wikipedia. org/ “Electron paramagnetic resonance” https://www. / “Electron paramagnetic resonance” XuMuK_ru https://www. – “ELECTRONIC PARAMAGNETIC RESONANCE” https://medx. fbm. / "Faculty of Fundamental Medicine of Moscow State University"
Get text
Resonance: atomic, partial and molecular
Atomic resonance is the absorption of electromagnetic waves by the nuclei of an atom, which occurs when the vector of its angular momentum changes. AR manifests itself especially often in atoms that are placed in a strong magnetic field. In this case, they must be exposed to a small electromagnetic field, characterized by the radio frequency range.
Nuclear magnetic resonance graph
There is also a theory of resonance in this area. According to it, chemical compounds have an electronic structure, and the distribution of electrons in the molecules of a substance is a combination or resonance of a structure with a different structure.
Important! This means that the structure of a molecule is described not only by one possible structural formula, but by a combination (resonance) of other structures. The resonance theory allows us to visualize the construction of mats using chemical terminology and classical formulas
model of the wave function of any complex molecule.
Resonation is used in a frequency meter
At the molecular level
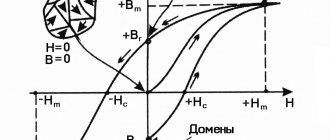
Conventional ferromagnets
that we encounter in everyday life (for example, objects made of transition metals such as iron) exhibit magnetization in the absence of an external magnetic field.
Moreover, their magnetism is realized at the level of domains with minimal sizes of tens of nanometers, that is, ensembles of millions of individual atoms. At the same time, high-density recording of information and devices for processing it require smaller carriers of the magnetic moment. Magnetic resonance is the phenomenon of resonant absorption of the energy of an alternating electromagnetic field by a system that includes fragments that have a non-zero intrinsic magnetic moment - spin.
This absorption causes transitions between energy levels due to different spatial orientations of the spins. In the case of nuclei, this phenomenon is called nuclear magnetic resonance (NMR), for electrons - electron paramagnetic resonance (EPR). Here, promising candidates are “magnetic molecules”, i.e. microscopic objects with a typical size of the order of a nanometer that exhibit useful magnetic properties - molecular magnetism
. Such compounds traditionally include paramagnetic molecules containing one or more unpaired electrons. By combining many such molecules, we can obtain a macro-object with a qualitatively different magnetism, while the magnetic properties of the molecular magnets themselves can be set through directed molecular design.
Molecular magnetism is a rapidly developing area of scientific knowledge at the intersection of chemical and physical sciences, and the EPR Spectroscopy Laboratory conducts research in several areas in this area.
Thus, the EPR method turned out to be extremely informative in the study of magnetic structural anomalies in the case of molecular magnets created on the basis of polymer complexes of copper (II) with stable nitroxyl radicals *, which were discovered more than 15 years ago in the laboratory of multi-spin coordination compounds of the ITC SB RAS, headed by Academician V.I. Ovcharenko.
These complexes are unique in that their magnetic moment changes (smoothly or abruptly) depending on temperature. Such manifestations are typical for the so-called spin crossover
, when under the influence of external influences in a transition metal the spin configuration of the internal electron
d
shell, which is occupied by valence electrons, changes.
However, in the case of copper-nitroxide compounds, classical spin crossover is impossible, since both radicals and copper(II) have only one unpaired electron with spin S = 1/2. Nevertheless, in this case, a change (“switching”) of the total magnetic moment of the entire copper-nitroxide cluster can occur, and the change in magnetic properties is accompanied by a structural transformation.
Using EPR in such molecular magnets, they were studied as magnetic ( exchange
) interactions during “non-classical” spin transitions, as well as weaker exchange interactions between individual copper-nitroxide clusters. In this way, it was possible to establish a one-dimensional topology of magnetic “chains” in crystals grown on the basis of these compounds, when the channels through which magnetic interactions propagate are unidirectional chains. Finally, using laser excitation, it was shown for the first time that this class of molecular magnets can be “switched” by light, which is important for practical applications in spintronics.
In parallel, combined EPR methods are being developed and applied to study another class of molecular magnets - monomolecular
(MMM). The phenomenon of monomolecular magnetism, first experimentally discovered in 1991, is that a substance exhibits the properties of a permanent magnet in the absence of an external magnetic field already at the level of an individual molecule. And in this, MMMs are fundamentally different from classical ferromagnets, in which magnetization, as mentioned above, is a cooperative property.
Since MMMs are bistable, i.e., they can exist in two equivalent states, one molecule is capable of storing one bit of information and serving as a single block of ultra-dense information storage devices, spin transistors and elements of quantum computers.
How does MMM work? When such a molecule is placed in an external magnetic field, the spins of its unpaired electrons are oriented in the direction of the external field, since this orientation is the most energetically favorable. In this case, even a magnetically insulated molecule will lose magnetization extremely slowly, and the main reason for this behavior is magnetic anisotropy
, which in general means the dissimilarity of the magnetic properties of bodies in different directions.
In the case of MMM, we are talking about the splitting of the energy levels of the spin system, which leads to the formation of an energy barrier between states with opposite magnetization. For modern MMMs, this barrier is already quite high, and we can observe MMM behavior even at liquid nitrogen temperatures.
To use MMMs in applied problems, you need to learn how to manipulate their magnetization, i.e., spin state. Thus, to “reversal magnetization” of a molecule, it is necessary to go through several of its spin states in a controlled manner, separated by an energy barrier. This barrier for most known MMMs lies in the terahertz and far-IR regions, and in order to induce the corresponding spin transition, just such an energy quantum is required.
This monochromatic emission corresponds to the range of a free electron laser
(FEL) at the Siberian Center for Synchrotron and Terahertz Radiation Center (Novosibirsk). This is how a scientific project to study MMM was born, jointly with the Institute of Nuclear Physics of the SB RAS. Within its framework, an EPR spectroscopy research station was created, where unique experiments are carried out with the goal of learning how to controllably “reversal magnetization” of MMMs by selectively exciting spin transitions with laser radiation.
Types of resonance phenomena
To calculate the parameters of a mechanical system, you can continue to study the pendulum. The natural movement of the swing is slowed down by friction of functional components and air resistance. To prevent vibration damping, an external force (F) must be applied. Maximum efficiency will be ensured by frequency matching. The calculation algorithm is shown below.
v = ((F* Δt)/m) * N,
- N – number of pulses;
- m is the total mass of the load.
(m*v2)/2 = m*g*h = m*g*L*(1-cos α).
From these combinations, simple transformations yield two formulas for calculations:
- N = (m/(F* Δt)) * √(2*g*L*(1-cos α));
- t (total time to perform N oscillations) = N*T = (2π*m*L)/(F* Δt)) * √(2*(1-cos α)).
Substituting certain initial values, the periodicity of the necessary resonant oscillations is calculated:
- m=100kg;
- F = 10N;
- L = 200 cm;
- Δt = 1 s;
- N = 34;
- t = 96;
- T = 2.8 s.
The phenomenon of resonance can be observed in AC circuits when the frequencies of the power source (signal) and the reactive components of the circuit coincide. In this case, we can consider electrical resistance as an analogue of friction forces in a mechanical system.
Current resonance
To create the necessary conditions, you can use a parallel connection of standard elements (R, L and C). If we ensure equality of the impedances of the reactive components, at a certain frequency the total value of the currents in the corresponding circuits will be greater than the current of the power source. The graphics in the figure demonstrate a vector representation of electrical parameters.
Xc = 1/(2π*f*C),
- Xc – resistance;
- f – frequency;
- C – capacity.
XL = 2π*f*L.
Fresonance = 1/2π * √ (L*C).
Conditions for voltage resonance in a series circuit
If you use a transformer to form a connection between two oscillatory circuits, the calculation becomes more complicated. To create the necessary conditions, the equality of the reactive components is ensured.
Resonance curves of coupled circuits
Nonlinear systems
If there are no symmetrical reactions to external influences, resonance phenomena manifest themselves in a special way. In particular, the presence of a coil with a ferrite core in the circuit significantly complicates accurate calculations. In such materials, the magnetic properties are determined by the nonlinear distribution of elementary components.
The word resono is Latin for response. An oscillating system responds to external oscillatory influences. As the external frequency approaches its own frequency, it responds with a sharp increase in the amplitude of its forced periodic deviations from the equilibrium state.
Resonance phenomenon
Important! Resonance and unison are not the same phenomena. Unison is the coincidence of sounds in tone
In this case, there is no increase in the amplitude of sound vibrations, but a “one-voice” of two or more sound sources occurs.
Two strings can sound in unison if a force is simultaneously applied to them, causing them to vibrate. But one can resonate with the other at the moment their vibration frequencies coincide and increase the volume of its sound.
What are the benefits or harms of the phenomenon?
In order to talk about the positive or negative impact of the coincidence of oscillation frequencies, we need to remember its manifestation in one or another area of human activity.
Positive sides
There are many examples where the phenomenon of resonance is used. A sound wave is a vibration of air. Instruments have the ability to sound beautiful if the size, shape and material create the conditions for resonance. All wind and reed instruments sound due to the coincidence of sound frequencies.
When designing and constructing concert halls, the effect of acoustic resonance is used. The sound of music and artists' voices completely depends on the properties of vibrational movements. The ancient architects of the Middle Ages were excellent at the art of constructing structures with a strong acoustic effect. In St. Paul's Cathedral (London) there is a gallery where any sound or whisper can be heard clearly.
In the mining industry, when destroying or crushing hard rocks, the method of resonant destruction is used. This allows you to complete a large volume in a short time with great efficiency. Drilling holes in concrete structures is easier with a drill with a hammer drill function.
Large bells in temples are difficult to swing without a resonant effect. A child can disperse a massive tongue if he pulls the rope in time with the free movement. An adult will not be able to help him if his efforts do not resonate.
The magnitude of the frequency of alternating current is measured based on the phenomenon of coincidence of oscillation frequencies. The frequency meter device is used where it is necessary to monitor constant frequency values in electrical circuits.
Negative effect
The phenomena of coincidence of oscillation frequencies are diverse. When walking along a board between trenches, there is a possibility that the rhythm of the step and the system will coincide. Its role is played by a wooden base with a person. As a result, the board will begin to bend strongly (up, down).
A similar situation was recorded in 1906 in St. Petersburg on the Egyptian Bridge. As the cavalry squadron passed in formation, the precise rhythm of the trained horses coincided with the vibrations of the structure across the Fontanka River. The resonance led to the sudden destruction of the strong bridge.
To prevent such situations, military units are instructed to cross such structures at a free pace, and not “in step.” There is a speed limit for trains crossing the bridge for safety reasons. Therefore, impacts of wheels with rails at the joints occur less often than swings of the bridge. In some cases, for fast trains, the opposite principle is used: the speed is increased and the trains pass at maximum speed.
The ship has its own rocking period, and when the frequencies of the sea wave coincide with the floating craft, the rocking increases significantly. In this situation, the captain needs to change the speed or slightly veer off course. As a result of the actions, the period of the waves changes, the pitching returns to normal.
When large industrial mechanisms operate, force often arises due to imbalance (poor alignment, curvature of the supporting shaft). Its force is directed towards the support; the period of application may coincide with the vibrations of the foundation itself or the rotation of the shaft. As a result of resonance, huge structures are destroyed and load-bearing rotating parts are broken. To prevent emergency equipment failure, you need to take timely measures to weaken the effect.
Resonance in physics for
We often hear the word resonance: “public resonance”, “event that caused resonance”, “resonant frequency”. Quite familiar and ordinary phrases. But can you say exactly what resonance is?
If the answer jumped out at you, we are truly proud of you! Well, if the topic “resonance in physics” raises questions, then we advise you to read our article, where we will talk in detail, clearly and briefly about such a phenomenon as resonance.
Before talking about resonance, you need to understand what oscillations are and their frequency.
Oscillations and Frequency
Oscillations are a process of changing the states of a system, repeated over time and occurring around an equilibrium point.
https://youtube.com/watch?v=PClMmgvEyH8
The simplest example of oscillation is riding on a swing. We present it for a reason; this example will be useful to us in understanding the essence of the phenomenon of resonance in the future.
Resonance can only occur where there is vibration
And it doesn’t matter what kind of vibrations they are - electrical voltage fluctuations, sound vibrations, or just mechanical vibrations
In the figure below we describe what fluctuations can be.
Types of vibrations
By the way! For our readers there is now a 10% discount on any type of work
Oscillations are characterized by amplitude and frequency. For the swings already mentioned above, the oscillation amplitude is the maximum height to which the swing flies. We can also swing the swing slowly or quickly. Depending on this, the oscillation frequency will change.
Oscillation frequency (measured in Hertz) is the number of oscillations per unit time. 1 Hertz is one oscillation per second.
When we swing a swing, periodically rocking the system with a certain force (in this case, the swing is an oscillatory system), it performs forced oscillations. An increase in the amplitude of oscillations can be achieved if this system is influenced in a certain way.
By pushing the swing at a certain moment and with a certain periodicity, you can swing it quite strongly, using very little effort. This will be a resonance: the frequency of our influences coincides with the frequency of oscillations of the swing and the amplitude of the oscillations increases.
Resonance on a swing
The essence of the phenomenon of resonance
Resonance in physics is a frequency-selective response of an oscillatory system to a periodic external influence, which manifests itself in a sharp increase in the amplitude of stationary oscillations when the frequency of the external influence coincides with certain values characteristic of a given system.
The essence of the phenomenon of resonance in physics is that the amplitude of oscillations increases sharply when the frequency of influence on the system coincides with the natural frequency of the system.
There are known cases when the bridge along which soldiers were marching resonated with the marching step, swayed and collapsed. By the way, this is why now, when crossing the bridge, soldiers are supposed to walk at a free pace, and not in step.
The Egyptian Bridge in St. Petersburg, which collapsed due to resonance.
Examples of resonance
The phenomenon of resonance is observed in a variety of physical processes. For example, sound resonance. Let's take a guitar. The sound of the guitar strings itself will be quiet and almost inaudible.
However, there is a reason that the strings are installed above the body - the resonator.
Once inside the body, the sound from the vibrations of the string intensifies, and the one who holds the guitar can feel how it begins to slightly “shake” and vibrate from the blows on the strings. In other words, resonate.
Another example of observing resonance that we encounter is circles on water. If you throw two stones into the water, the passing waves from them will meet and increase.
Guitar resonator
Resonance can be both beneficial and harmful. And reading the article, as well as the help of our student service in difficult educational situations, will only bring you benefit. If, while completing your coursework, you need to understand the physics of magnetic resonance, you can safely contact our company for quick and qualified help.
Finally, we suggest watching a video on the topic “resonance” and making sure that science can be exciting and interesting. Our service will help with any work: from an essay on “The Internet and Cybercrime” to a coursework on the physics of oscillations or an essay on literature.
Lesson plan on the topic: “Forced vibrations. Resonance" (9th grade). Author Belaga
Class 9
Subject
: Forced vibrations. Resonance.
The purpose of the lesson:
explain why forced vibrations are more important than free ones; how forced oscillations are established; when there is a sharp increase in amplitude and resonance occurs;
Tasks:
· Educational –
provide students with knowledge of the concepts of free and forced vibrations; explain the meaning of forced oscillations; establish the origin of forced oscillations and the occurrence of resonance.
· Developmental –
development of the concept of application and harm caused by resonance in nature; development of imaginative thinking of oscillatory processes in nature; develop the ability to work with a book.
· Educating
– fostering a conscious and serious attitude towards the academic discipline. Formation of views on the development of the nature of oscillatory processes and connections with the outside world. Cultivating interest in the subject
Lesson type:
lesson formation of new knowledge
Methods:
verbal, lecture, demonstration, explanatory and illustrative
Types of student activities:
work with a textbook, independent work.
During the classes:
1. Org. moment (greeting, checking readiness for the lesson, motivation for learning activities, students’ mood).
2. Updating the necessary knowledge.
3. Checking homework using individual questioning.
1. What are mechanical vibrations called? (Mechanical vibrations are body movements that repeat exactly or approximately at equal intervals of time.)
2. Name the main characteristics of mechanical vibrations (The main characteristics of mechanical vibrations are: displacement, amplitude, frequency, period.)
3. What is offset? (Displacement is the deviation of a body from its equilibrium position.)
4. What is the amplitude of oscillations called? (Amplitude is the modulus of the maximum deviation from the equilibrium position.)
5. What is the oscillation frequency? (Frequency is the number of complete oscillations performed per unit time.)
6. What is called the period of oscillation ? (Period is the time of one complete oscillation, i.e. the minimum period of time after which the process repeats.)
7. How are the period and frequency of oscillations related to each other? (Period and frequency are related by the relation: ν = 1/T)
8. Which oscillations are damped?
4. Studying a new topic.
Forced oscillations of a spring pendulum.
Let us consider how forced oscillations arise and are maintained in an oscillatory system with its own frequency. If you rotate the installation handle, a periodic external force will begin to act on the body. The body will sway with increasing amplitude. After some time, the oscillations will have a steady-state character, and the amplitude will stop increasing. The frequency of vibration of the load will be equal to the frequency of rotation of the handle (the frequency of change in the external force).
1. Energy conversion during mechanical vibrations
.
Let's consider the process of energy conversion using the example of oscillations of a load on a thread (Fig. 10).
When the pendulum deviates from the equilibrium position, it rises to a height h relative to the zero level,
therefore, at point A the pendulum has potential energy mgh. When moving to the equilibrium position, to point O, the height decreases to zero, and the speed of the load increases, and at point O all the potential energy mgh will turn into kinetic energy mυ2/2. At equilibrium, kinetic energy is at its maximum and potential energy is at its minimum. After passing the equilibrium position, the kinetic energy is converted into potential energy, the speed of the pendulum decreases and, at the maximum deviation from the equilibrium position, becomes equal to zero. With oscillatory motion, periodic transformations of its kinetic and potential energy always occur.
With free mechanical vibrations, energy loss inevitably occurs to overcome resistance forces. If oscillations occur under the influence of a periodic external force, then such oscillations are called forced
.
The nature of forced oscillations depends on the nature of the action of the external force, on its magnitude, direction, frequency of action and does not depend on the size and properties of the oscillating body
.
Resonance
(from the Latin word resonans - giving an echo)
Using the same setup, let’s check how the amplitude of steady-state oscillations depends on the frequency of the external force. The amplitude begins to increase with a further increase in the frequency of the external force. It reaches its maximum if the free vibrations of the load act in time with the external force. The amplitude tends to zero if the frequency of the external force is very high.
As a result of inertia, the body does not have time to move and “trembles in place.”
The dependence of the amplitude on the external frequency is presented in the figures
.
Resonance is a sharp increase in the amplitude of forced oscillations when the frequency of free oscillations coincides with the frequency of change in the external force.
3. Application of resonance and combating it
.
The phenomenon of resonance plays an important role in a number of natural, scientific and industrial processes. For example, it is necessary to take into account the phenomenon of resonance when designing bridges, buildings and other structures that experience vibration under load, otherwise under certain conditions these structures may be destroyed. The phenomenon of resonance can cause the destruction of cars, buildings, bridges if their natural frequencies coincide with the frequency of a periodically acting force.
Therefore, for example, engines in cars are installed on special shock absorbers, and military units are prohibited from keeping pace when moving across the bridge.
4. Consolidation. Independent work with the textbook.
Questions for consolidation.
1. What oscillations are called forced? (Oscillations occurring under the influence of an external periodic force).
2. How do forced vibrations occur, under the influence of what forces? (An external periodic force, called a driving force, imparts additional energy to the oscillatory system, which goes to replenish the energy losses occurring due to friction.)
3. How do forced oscillations differ from free oscillations? (Unlike free oscillations, when the system receives energy only once (when the system is brought out of equilibrium), in the case of forced oscillations the system absorbs this energy from a source of external periodic force continuously.)
4. What is the total energy of the oscillatory system? (This energy makes up for the losses spent on overcoming friction, and therefore the total energy of the oscillatory system still remains unchanged.)
5. How does the frequency of forced oscillations depend on the frequency of the driving force? (The frequency of forced oscillations is equal to the frequency of the driving force.)
6. What do we call the phenomenon of resonance? (In the case when the frequency of the driving force υ coincides with the natural frequency of the oscillatory system υ0, a sharp increase in the amplitude of forced oscillations occurs - resonance.)
7. What causes the phenomenon of resonance? (Resonance arises due to the fact that at υ = υ0 the external force, acting in time with free oscillations, is always aligned with the speed of the oscillating body and does positive work: the energy of the oscillating body increases, and the amplitude of its oscillations becomes large.)
5. Homework:
§ 13, pp. 34-35
6. Lesson summary
Resonance frequency
The procedure associated with a change in the position of the system near the equilibrium point and repeated over time is called oscillations. A swinging pendulum repeats its movements relative to the normal to the horizontal plane. At the same time, if additional energy is not applied to its movement, its swinging will fade.
The phenomenon of such changes can be classified according to the following parameters:
- according to the mathematical model used in oscillations;
- according to the periodicity structure;
- by the nature of physical properties;
- by type of interaction with environmental conditions.
Attention! All vibrations, regardless of their physical properties, have general laws that can be described by wave phenomena. These patterns are studied by the theory of wave oscillations
Mechanical vibrations are associated with the transformation of one form of energy into another, wave vibrations are associated with the spatial movement and distribution of energy.
The common parameters for all oscillations are:
- frequency;
- period;
- amplitude.
Period (T) is the time of a whole (complete) oscillation, during which it is possible to record the repetition of any of the characteristics of the system state. This means that she has completed a complete swing. The designation of the period is T, the unit of measurement is second (s).
The greatest deviation of a point of a body or any quantity of a system from an equilibrium position is called the amplitude of oscillations and is denoted by the letter A. The unit of measurement is those quantities whose changes are being considered. For mechanical deviations, the amplitude is measured in meters (m), the amplitude of alternating voltage is measured in volts (V), and so on.
Period and frequency of mechanical vibrations
ωres = √(ω02 – 2ß2).
In this formula:
- ωres – resonance frequency;
- ω0 – angular frequency;
- ß – attenuation coefficient.
When the attenuation coefficient increases, the resonance phenomenon decreases.
Resonance of currents through reactive elements
Resonance of currents appears in electrical circuits of alternating current circuits under the condition of parallel connection of branches with different reactances. In the resonant mode of currents, the reactive inductive conductivity of the circuit will be equivalent to its own reactive capacitive conductivity, i.e. $BL = BC$.
The oscillations of the circuit, the frequency of which has a certain value, in this case coincide in frequency with the voltage source.
The simplest electrical circuit in which we observe current resonance is considered to be a circuit with a parallel connection of a capacitor to an inductor.
Since the reactivity resistances are equal in magnitude, the amplitudes of the currents $I_c$ and $I_u$ will be the same and can reach their maximum amplitude. Based on Kirchhoff's first law, $IR$ is equal to the source current. The source current, in other words, flows only through the resistor. When considering a separate parallel circuit $LC$, at the resonant frequency its resistance turns out to be infinitely large: $ZL = ZC$. When a harmonic mode with a resonant frequency is established, the circuit is observed to provide a certain steady-state oscillation amplitude with a source, and the power of the current source is spent exclusively on replenishing losses in the active resistance.
Thus, the impedance of a series $RLC$ circuit turns out to be minimal at the resonant frequency and equal to the active resistance of the circuit. At the same time, the impedance of a parallel $RLC$ circuit is maximum at the resonant frequency and is considered equal to the leakage resistance, which is actually also the active resistance of the circuit. In order to ensure conditions for resonance of current or voltage, it is necessary to check the electrical circuit to predetermine its complex resistance or conductivity. In addition, its imaginary part must be equal to zero.
Examples that are repeated by many
Another example, which is even included in jokes, is the breaking of dishes by sound vibrations, from practicing the violin and even singing. Unlike a company of soldiers, this example was repeatedly observed and even specially tested. Indeed, the resonance that occurs when the frequencies coincide leads to the splitting of plates, glasses, cups and other utensils.
This is an example of process development under conditions of a high-quality system. The materials from which the dishes are made are fairly elastic media in which vibrations propagate with low attenuation. The quality factor of such systems is very high, and although the frequency coincidence band is quite narrow, resonance leads to a strong increase in amplitude, as a result of which the material is destroyed.
Operating principle
As the simple example of a swing shows, the system has a certain frequency (v). Without calculations, it is clear that the lengthening (L) of the rope at the same deviation from the vertical by an angle (α) provokes an increase in the period of oscillation (T).
Resonance is defined in physics in the 11th grade of the school curriculum as an increase in amplitude when the natural frequency of a structure coincides with external influences. Chaotic shocks provoke the opposite effect - braking of the pendulum. This result is explained by the opposite direction of the vectors of the corresponding forces.
Physical definition and binding to objects
Resonance, by definition, can be understood as a fairly simple process:
- there is a body that is at rest or oscillates with a certain frequency and amplitude;
- it is acted upon by an external force with its own frequency;
- in the case when the frequency of the external influence coincides with the natural frequency of the body in question, a gradual or sharp increase in the amplitude of oscillations occurs.
However, in practice the phenomenon is considered as a much more complex system. In particular, the body can be represented not as a single object, but as a complex structure. Resonance occurs when the frequency of the external force coincides with the so-called total effective oscillatory frequency of the system.
Resonance, if we consider it from the standpoint of physical definition, must certainly lead to the destruction of the object. However, in practice there is a concept of the quality factor of an oscillatory system. Depending on its value, resonance can lead to various effects:
- with a low quality factor, the system is not able to retain oscillations coming from outside to a large extent. Therefore, there is a gradual increase in the amplitude of natural vibrations to a level where the resistance of materials or connections does not lead to a stable state;
- high quality factor, close to unity, is the most dangerous environment in which resonance often leads to irreversible consequences. These may include both mechanical destruction of objects and the release of large amounts of heat at levels that can lead to fire.
Also, resonance occurs not only under the action of an external force of an oscillatory nature. The degree and nature of the system's response is, to a large extent, responsible for the consequences of externally directed forces. Therefore, resonance can occur in a variety of cases.
Meaning of the concept
What is the phenomenon in mechanics and physics? Let's explain resonance in simple words in everyday life - this is a coincidence of the rhythm of movement. We need to remember some pleasant fun from childhood. We are talking about swinging on a hanging swing. One participant sits on the bar, the other helps him, pulling the seat more and more. A child could just as easily be in the assistant’s place; he or she can rock an adult. This “works” is mechanical resonance, in which the vibrations of the swing completely coincide with the frequency of the assistant. As a result, we get a jump in amplitude.
When swinging on a swing on your own, it is realistic to use the coincidence of vibrations for maximum range of motion:
- In a sitting position. You need to tuck and straighten your lower limbs to the beat.
- In a standing position. It's easier to swing together. In the beloved Boat ride, each participant must squat at the point of greatest lift, and then straighten up in the lowest possible position.
All efforts can actually lead to the swing making a full revolution around its axis. To prevent accidents, a circuit limiter is installed for the safety of vacationers. You need to understand that in order to get the effect of the coincidence of oscillatory movements, you need to get out of the resting state. Balance will not allow the swaying to increase. The described example relates to parametric excitation and vibration resonance.
The amplitude of the oscillations depends on the speed of movement. As it increases, the magnitude increases until it reaches its maximum. A further increase in speed will have the opposite effect. When constructing a graph of resonance - the dependence of the amplitude on the applied external force, we obtain a curve. The absolute maximum corresponds to a frequency that coincides with the natural oscillation frequency of the system. In physics and mechanics, resonance formulas are used - the dependence of amplitude on frequency and applied force.
Units
The number of movements is usually measured in Hertz (1 Hz). If the frequency value is known, for example 45 Hz, the body vibrates 45 times per second. There is a concept of forced movements, in this case there is a rocking body and a coercive force. The force is applied with a certain frequency. If there is a large difference in characteristics, there will be no jump in oscillatory movements.
The phenomenon was first explained and described from the point of view of mechanics and acoustics in 1602 by Galileo Galilei. His work was devoted to the oscillatory phenomena of pendulums and strings for musical instruments. In the description, the scientist deduced the dependence of a heavy pendulum to collect (accumulate) energy under external influence with a certain frequency value. The term was coined from the Latin word "resonantia", meaning echo. The magnetic form of the concept was theorized by James Clerk Maxwell in 1808.
Nuclear magnetic resonance
Certain types of atoms contain nuclei that can be compared to miniature magnets. Under the influence of a powerful external magnetic field, the nuclei of atoms change their orientation in accordance with the relative position of their own magnetic field in relation to the external one. An external strong electromagnetic pulse is absorbed by the atom, resulting in its reorientation. As soon as the source of the impulse ceases its action, the nuclei return to their original positions.
Nuclei, depending on their belonging to a particular atom, are capable of receiving energy in a certain frequency range. The change in the position of the nucleus occurs in one step with external oscillations of the electromagnetic field, which is the reason for the occurrence of the so-called nuclear magnetic resonance (abbreviated NMR). In the scientific world, this type of resonance is used to study atomic bonds within complex molecules. The magnetic resonance imaging (MRI) method used in medicine allows the results of scanning of internal human organs to be displayed on a display for diagnosis and treatment.
The magnetic field of the OMR scanner, formed using inductance coils, creates high-frequency radiation under the influence of which the nuclei of hydrogen atoms change their orientation, provided that their natural frequencies coincide with the external one. As a result of the data received from the sensors, a graphic image is formed on the monitor.
If we compare the NMR and OMR methods regarding the negative impact of radiation on the human body, then scanning using a nuclear magnetic resonator is less harmful than OMR. Also, in the study of soft tissues, NMR technology has shown greater efficiency in reflecting the detail of the tissue area under study.
What types of resonance exist?
The phenomenon is characterized by features; types are distinguished:
- Mechanical. When designing industrial facilities, safety measures must be taken into account. If the mechanical frequencies of the basis of machines and mechanisms coincide with the vibrations of the engine, a resonant effect may occur.
- Electric. Observed in electrical circuits at a certain frequency. The phenomenon is used in wireless signal transmission - television, cellular communications.
- Optic. With a special arrangement of optical cavities (mirrors), a resonator for light waves is observed. The phenomenon is used in laser systems and parametric generators.
- Nuclear magnetic resonance. Abbreviated as NMR, it is used in medical diagnostics, when performing magnetic resonance imaging.
- Public. Society often uses the concept of a response to an event, phenomenon or random occurrence. The response to the incident is a similar reaction of a large mass of people. A recent example is the increase in the retirement age introduced by Federal Law in 2022. As a result, the response from the majority of citizens was the same - negative and disagreement with the decision.
- Cognitive or psychological. If the subject meets someone and has a positive impression, we can talk about the consequence of resonance. At the same time, interests, judgments, and opinions coincide. In psychology, resonance is the unity of souls, aspirations and emotions.
- Plasmon resonance. In quantum physics, the concept of plasmon is used. These are quasiparticles in current conductors, when excited at a certain frequency that coincides with an external electromagnetic wave. The phenomenon is used in the design of sensors for chemical or biological systems.
Helmholtz resonator
Humanity has known the amazing properties of empty vessels for a long time. Ancient architects used knowledge of sound resonance when building a theater: they placed bronze vessels in the walls to make the voices of the actors sound louder. Helmholtz resonators are widely used in acoustics. Helmholtz is a German scientist who substantiated the theory of hearing from a physical point of view. Using a set of resonators named after him, complex sounds can be analyzed based on the frequency of the wave's oscillations.
How does a resonator work? It is a spherical or bottle-shaped vessel with a narrow neck. The whole secret lies in the sound resonance of the vibrations of the air that is inside. The sound wave is complex. It consists of many vibrations. But each of the resonators responds best to the frequency that is equal to its own, that is, the frequency of vibration of the air enclosed in the cavity. What does it depend on?
If the resonator is shorter than the sound wavelength, then its operating principle is the same as that of a spring pendulum. The air in the narrow neck moves much faster than in the resonator itself. It is the vibrations in the neck of the vessel that play the main role. It turns out that the kinetic energy is concentrated mainly in this bottleneck. Elastic energy is carried by the air mass located inside the resonator.
There is much less air in the neck than inside, so the change in its volume during oscillations is usually neglected. It is conventionally believed that this entire mass moves as a single whole, like an air plug, and the volume of air inside the resonator changes greatly. It turns out that the air inside works like a spring in an oscillatory system. Its influx blocks the path of other air into the vessel, and the outflow lowers the pressure and prevents the release of air from the inside. When the air plug goes down, it compresses the nearby layer of air inside the resonator, i.e., increases its density. As a result, the growing pressure sets in motion the next layer of air, then another, etc. Thus, the compression spreads across the layers, transmits its momentum, and a sound wave arises.
It is now clear that the cause of the creepy voices in the apartment building was sound resonance. The howling of the wind and other noises from the street are disordered harmonic vibrations of different frequencies. They are called pure tones. When passing through the wall, all frequencies except resonant ones weakened. Resonant frequencies are those that coincide with the frequencies of the air in empty vessels. Moreover, they could even intensify. The policemen panicked because they heard sounds unusual for humans and living beings. The fact is that our speech sounds at a frequency much higher than 100 hertz, and the “brownie” made unusually low sounds.
Organized himself
self-organizing systems can also exhibit a number of unusual properties.
, in which ordering occurs on the nano- and microscale.
A special place among them is occupied by ionic liquids
(ILs). In the simplest case we are talking about molten salts, but in general the spectrum of ILs is quite wide and covers numerous combinations of organic and inorganic cations and anions. The range of their amazing physicochemical properties (primarily nano- and microheterogeneity) is also diverse, which can find application in a variety of fields of science and industry: from catalysis to biomedicine.
To date, many different types of nanostructures formed in ILs have been discovered, mainly theoretically: ion pairs, substructures based on hydrogen bonds, ion clusters, micelles-like nanostructures, sponge-like arrays of micrometer-scale nanostructures. However, many of the results of theoretical modeling are quite difficult to confirm experimentally.
In most cases, ILs are diamagnetic and therefore cannot be studied in their “pure form” using the EPR method. To do this, a special paramagnetic spin probe should be dissolved in them (usually in trace amounts).
This approach has its advantages and disadvantages. On the one hand, the probe molecules themselves, dissolved in pure IL, can specifically interact with the solvent. On the other hand, if even trace amounts of water (tens of parts per million!) are present in an IL, then its system of hydrogen bonds can change greatly, which will significantly affect the physicochemical properties. In addition, the question always arises: are the observed inhomogeneities in an IL inherent specifically to it or are they a consequence of the presence of a guest molecule in it?
However, since in any case most of the applications of ILs are somehow related to their role as a solvent, the interactions in the solvent–solute pair are quite natural. In this sense, the use of spectroscopic methods using probe molecules is completely justified, and by selecting the structure of the probe, it is possible to simulate real interactions of ILs with specific substances. For this reason, in the laboratory of EPR spectroscopy, three variants of EPR techniques using spin probes were used to study the heterogeneous structure of ILs.
the stationary EPR method
The spectra of probes played by stable radicals were analyzed. It turned out that for a series of ILs in the temperature range 170–270 K, the experimental spectrum corresponds to a superposition of two different fractions of spin probe molecules with a fundamentally different model of motion. One fraction consists of rotating molecules, the other - of sedentary ones. This suggests that the softening and melting of ILs at the molecular level is a fairly smooth process.
In the EPR method with time resolution
Photoexcited molecules such as porphyrins or fullerenes were used as spin probes.
Their ground state is diamagnetic, but when irradiated with light they transform into long-lived triplet states
*, which can be detected using ESR.
It turned out that such probe molecules are present in ILs in the form of several different fractions with different microenvironments and, accordingly, physical properties. It can be assumed that such heterogeneity is associated with the formation of nanosized cavities, similar to micelles, in ILs. the spin polarization lifetime was noted
(non-equilibrium population of energy levels) in ILs compared to traditional solvents, which may be useful for some applications.
Pulse EPR
involves the use of a pulsed microwave field and is used in different versions, using specific pulse sequences, often in combination with additional radio frequency excitation of the sample.
In the laboratory, this method was used to analyze random molecular librations
(low-angle “jitters”) of stable radicals used as spin probes - such movements effectively shorten the spin relaxation time.
In particular, using this approach, a density anomaly was discovered for the first time for glassy ILs. It turned out that in the temperature range of 150–200 K the mobility of probe molecules progressively decreases with increasing temperature, which contradicts all known trends.
This behavior can be described by nanoscale structural rearrangements, during which the ensemble of radicals in the IL matrix breaks down into two subgroups. Radicals located in the region of low density of the IL matrix begin to experience diffusion rotation, which is detected using stationary ESR, and those localized in other regions with increased density are detected using pulsed EPR. And although the average density of the IL remains constant, thanks to the selectivity of the techniques, it is possible to detect local density inhomogeneities that change with temperature up to the phase transition at the glass transition point.
It is interesting that such anomalies are observed not only in pure ILs, but also in their mixtures with water, which may be important, for example, in the development of new types of cryoprotectors.
Types and examples of resonance
Only in physics itself do we distinguish such types of resonance as:
- Mechanical resonance is the same aforementioned swing, the resonance of a bridge from a passing company of soldiers, the resonance of a bell ringing, etc. In a word, resonance caused by mechanical influences.
- Acoustic resonance is the resonance that makes all stringed musical instruments work: guitar, violin, lute, balalaika, banjo, etc. By the way, the body of musical instruments has its own shape for a reason. The sound produced by the string when plucked enters the body and there enters into resonance with the walls, which as a result leads to its amplification. For this reason, the sound quality of the same guitar greatly depends on the material from which it is made and even on the varnish with which it is coated.
- Electrical resonance is the coincidence of the oscillation frequency of the external voltage with the oscillation frequency of the electrical circuit through which the current flows.
In addition to these purely physical resonances, there is also the public resonance that we have already mentioned - a strong public response to some event (usually political or economic), for example, Brexit, Britain, its withdrawal from the European Union caused a wide public resonance in many European countries and especially, of course, in Britain itself.
There is also cognitive resonance - this is a complete coincidence in views and opinions. For example, you met a new person, and he thinks the same way as you, you have absolutely similar views, tastes, preferences, then cognitive resonance takes place. And the opposite phenomenon is cognitive dissonance, when you absolutely disagree with someone or something and absolutely do not accept what is happening. (For example, the author of this article, finding himself in some Ukrainian bureaucratic institution, be it Zheka, BTI or tax office, experiences real cognitive dissonance)).
The benefits and harms of resonance
In order to draw some conclusion about the pros and cons of resonance, it is necessary to consider in which cases it can manifest itself most actively and noticeably for human activity.
Positive effect
The response phenomenon is widely used in science and technology . For example, the operation of many radio circuits and devices is based on this phenomenon.
- Two-stroke engine. The muffler of a two-stroke engine has a special shape designed to create a resonant phenomenon. It improves engine performance by reducing consumption and pollution. This resonance partially reduces the unburned gases and increases compression in the cylinder.
- Musical instruments. In the case of string and wind instruments, sound production occurs mainly when the oscillatory system (strings, columns of air) is excited until the phenomenon of resonance occurs.
- Radios. Each radio station emits an electromagnetic wave with a clearly defined frequency. To capture it, the RLC circuit is forcibly vibrated by an antenna, which captures all electromagnetic waves that reach it. To listen to one station, the natural frequency of the RLC circuit must be tuned to the frequency of the desired transmitter by changing the capacitance of the variable capacitor (the operation is performed by pressing the station search button). All radio communication systems, whether transmitters or receivers, use resonators to "filter" the frequencies of the signals they process.
- Magnetic resonance imaging (MRI). In 1946, two Americans, Felix Bloch and Edward Mills Purcell, independently discovered the phenomenon of nuclear magnetic resonance, also called NMR, which earned them the Nobel Prize in Physics.
Negative impact
However, the phenomenon is not always useful . You can often find references to cases where suspension bridges broke when soldiers walked across them “in step.” At the same time, they refer to the manifestation of the resonant effect of resonance, and the fight against it becomes large-scale.
- Motor transport. Motorists are often annoyed by noise that occurs at certain vehicle speeds or as a result of engine operation. Some slightly rounded parts of the body resonate and emit sound vibrations. The car itself, with its suspension system, is an oscillator, equipped with effective shock absorbers that prevent sharp resonance from occurring.
- Bridges. The bridge can perform vertical and transverse vibrations. Each of these types of oscillations has its own period. If the lines are suspended, the system has a very different resonant frequency.
- Building. Tall buildings are susceptible to earthquakes. Some passive devices help protect them: they are oscillators whose natural frequency is close to the frequency of the building itself. Thus, the energy is completely absorbed by the pendulum, preventing the destruction of the building.
How to use
Resonant currents are used today in some filter systems, radio engineering, electricity, radio stations, asynchronous motors, high-precision electrical welding installations, oscillating generator electrical circuits and high-frequency devices. Often they are used to reduce the generator load.
Note! The simplest circuit where they are observed is a parallel oscillatory circuit. Such circuits are used in modern industrial induction boiler equipment and improve efficiency indicators
Scope of application
Mysterious house
In “Stories about Old Moscow” by A. Vyurkov, a house is described that sounds like a scary voice. The main character of the work, Ivan Pavlovich, decided to get rich by fraud. He hired a team of masons to build him an apartment building and did not pay them the full amount promised. Soon the tenants began to leave the hotel one after another, because they were frightened by the evil spirits that howled in an inhuman voice.
Ivan Pavlovich was left without money and without tenants. He had nothing to pay the interest on the loan, so his property and himself were seized. As time passed, one of the contractors revealed to Ivan Pavlovich the secret of the mystical house. It turns out that the deceived workers decided to take revenge: they walled up empty bottles in the wall, which sounded with every gust of wind, scaring the guests.
Experience with tuning forks
An acoustic wave is like a swing: if you push it haphazardly, losing the rhythm, then it will not fly high
The importance of matching frequency (rhythm) can be easily seen in the experiment with two tuning forks. Let's take those that have the same frequency and place them quite close to each other
Let's hit the legs of the first one with a hammer - it will sound, and very soon it will make the other one sound. Why will this happen? The second instrument will be set in motion (swinged) by the sound wave. When the first one is silent, the second one will make a sound for some time. This is how sound resonance occurs. If we perform an experiment on tuning forks of different frequencies, we will see that they do not resonate.