The definition of the concept of resonance (response) in physics is entrusted to special technicians who have statistics graphs who often encounter this phenomenon. Today, resonance is a frequency-selective response, where a vibration system or a sudden increase in external force causes another system to oscillate with greater amplitude at certain frequencies.
Operating principle
This phenomenon is observed when a system is able to store and easily transfer energy between two or more different storage modes, such as kinetic and potential energy. However, there is some loss from cycle to cycle, called attenuation. When the damping is negligible, the resonant frequency is approximately equal to the natural frequency of the system, which is the frequency of unforced oscillation.
These phenomena occur with all types of oscillations or waves: mechanical, acoustic, electromagnetic, nuclear magnetic (NMR), electron spin (ESR), and quantum wave function resonance. Such systems can be used to generate vibrations of a certain frequency (for example, musical instruments).
The term "resonance" (from the Latin resonantia, "echo") comes from the field of acoustics, especially seen in musical instruments, such as when strings begin to vibrate and produce sound without direct input from the player.
Examples of resonance in life
Pushing a person on a swing is a common example of this phenomenon. A loaded swing, a pendulum, has a natural vibration frequency and a resonant frequency that resists being pushed faster or slower.
An example is the oscillation of projectiles on a playground, which acts like a pendulum. A person's push while swinging at a natural swing interval causes the swing to go higher and higher (maximum amplitude), while attempting to swing at a faster or slower pace creates smaller arcs. This is because the energy absorbed by vibrations increases when the shocks correspond to natural vibrations.
The response is widely found in nature and is used in many artificial devices. This is the mechanism by which virtually all sine waves and vibrations are generated. Many of the sounds we hear, such as when hard objects made of metal, glass or wood hit, are caused by short vibrations in the object. Light and other short-wave electromagnetic radiation is created by resonance on the atomic scale, such as electrons in atoms. Other conditions in which the beneficial properties of this phenomenon may apply:
- Timekeeping mechanisms of modern watches, a balance wheel in a mechanical watch and a quartz crystal in a watch.
- Tidal response of the Bay of Fundy.
- Acoustic resonances of musical instruments and the human vocal tract.
- Destruction of a crystal glass under the influence of a musical right tone.
- Frictional idiophones, such as making a glass object (glass, bottle, vase), vibrate when rubbed around its edge with a fingertip.
- The electrical response of tuned circuits in radios and televisions that allow selective reception of radio frequencies.
- Creation of coherent light by optical resonance in a laser cavity.
- Orbital response, exemplified by some of the gas giant moons of the Solar System.
Atomic-scale material resonances are the basis of several spectroscopic techniques that are used in condensed matter physics, for example:
- Electronic spin.
- Mossbauer effect.
- Nuclear magnetic.
The destructive power of sound
Many people have probably heard that a wine glass can be broken with the voice of an opera singer. If you lightly hit a glass with a spoon, it will “ring” like a bell at its resonant frequency. If sound pressure is applied to glass at a certain frequency, it begins to vibrate. As the stimulus continues, vibration builds up in the glass until it collapses when the mechanical limits are exceeded.
Examples of beneficial and harmful resonance are everywhere. Microwaves are all around us, from the microwave oven that heats food without the use of external heat, to the vibrations in the earth's crust that cause devastating earthquakes.
2) When we tune a radio receiver, we change the natural frequency of the oscillating circuit, ensuring that it matches the frequency of the transmitting radio station.
3) When the violinist moves the bow along the string, energy is transferred to the string (tiny hooks on the horsehair stretched on the bow tug the string). A so-called parametric resonance occurs. The string begins to vibrate at its own frequency, depending on its length, tension and mass. The amplitude of vibration does not increase to infinity, since the energy of the vibrating string is immediately spent on creating sound waves that fly into space. The result is a balance: how much energy the violinist spends, the same amount is dissipated in the string itself and spent on emitting sound.
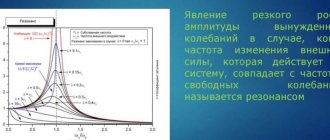
The phenomenon of resonance is that the amplitude of steady-state forced oscillations reaches its greatest value when the frequency of the driving force is equal to the natural frequency of the oscillatory system.
A distinctive feature of forced oscillations is the dependence of their amplitude on the frequency of changes in the external force. To study this dependence, you can use the setup shown in the figure:
A spring pendulum is mounted on a crank with a handle. When the handle rotates uniformly, a periodically changing force is transmitted to the load through a spring. Changing with a frequency equal to the frequency of rotation of the handle, this force will cause the load to undergo forced vibrations. If you rotate the crank handle very slowly, the load together with the spring will move up and down in the same way as the suspension point O. The amplitude of the forced oscillations will be small. With faster rotation, the load will begin to oscillate more strongly, and at a rotation frequency equal to the natural frequency of the spring pendulum (ω = ωob), the amplitude of its oscillations will reach a maximum. With a further increase in the rotation speed of the handle, the amplitude of forced oscillations of the load will again become smaller. A very fast rotation of the handle will leave the load almost motionless: due to its inertia, the spring pendulum, not having time to follow changes in the external force, will simply tremble in place.
The phenomenon of resonance can also be demonstrated with string pendulums. We hang a massive ball 1 and several pendulums with threads of different lengths on a rail. Each of these pendulums has its own oscillation frequency, which can be determined by knowing the length of the string and the acceleration of gravity.
Types of phenomenon
In describing resonance, G. Galileo drew attention to the most essential thing - the ability of a mechanical oscillatory system (heavy pendulum) to accumulate energy, which is supplied from an external source with a certain frequency. Manifestations of resonance have certain characteristics in different systems and therefore different types are distinguished.
Mechanical and acoustic
Mechanical resonance is the tendency of a mechanical system to absorb more energy when its vibration frequency matches the system's natural vibration frequency. This can lead to severe motion fluctuations and even catastrophic failure in unfinished structures, including bridges, buildings, trains and airplanes. When designing facilities, engineers must ensure that the mechanical resonant frequencies of component parts do not match the oscillatory frequencies of motors or other oscillating parts to avoid a phenomenon known as resonant disaster.
Electrical resonance
Occurs in an electrical circuit at a certain resonant frequency when the circuit impedance is minimum in a series circuit or maximum in a parallel circuit. Resonance in circuits is used to transmit and receive wireless communications such as television, cellular, or radio.
Optical resonance
An optical cavity, also called an optical resonator, is a special arrangement of mirrors that forms a standing wave resonator for light waves . Optical cavities are the main component of lasers, surrounding the amplification medium and providing feedback to the laser radiation. They are also used in optical parametric oscillators and some interferometers.
Light confined within the cavity produces standing waves repeatedly for specific resonant frequencies. The resulting standing wave patterns are called "modes". Longitudinal modes differ only in frequency, while transverse modes differ for different frequencies and have different intensity patterns across the beam cross section. Ring resonators and whispering galleries are examples of optical resonators that do not produce standing waves.
Orbital wobble
In space mechanics, an orbital response occurs when two orbiting bodies exert a regular, periodic gravitational influence on each other. This is usually because their orbital periods are related by the ratio of two small integers. Orbital resonances significantly enhance the mutual gravitational influence of bodies. In most cases, this results in an unstable interaction in which the bodies exchange momentum and displacement until resonance no longer exists.
Under some circumstances, a resonant system can be stable and self-correcting to keep bodies in resonance. Examples are the 1:2:4 resonance of Jupiter's moons Ganymede, Europa and Io and the 2:3 resonance between Pluto and Neptune. Unstable resonances with Saturn's inner moons create gaps in Saturn's rings. A special case of 1:1 resonance (between bodies with similar orbital radii) causes large Solar System bodies to clear out the neighborhoods around their orbits, pushing out almost everything else around them.
Atomic, partial and molecular
Nuclear Magnetic Resonance (NMR) is the name given to the physical resonance phenomenon associated with the observation of specific quantum mechanical magnetic properties of an atomic nucleus when an external magnetic field is present. Many scientific methods use NMR phenomena to study molecular physics, crystals, and non-crystalline materials. NMR is also commonly used in modern medical imaging techniques such as magnetic resonance imaging (MRI).
Series oscillating circuit
In an oscillatory circuit you can obtain undamped oscillations if you connect it to an alternating current source. If the source is connected in series with a coil L and a capacitor C, then such a circuit is called a series oscillatory circuit (Fig. 3).
When an external source is connected to the circuit, it is not the circuit’s own (free) oscillations that arise, which are determined by the values of L and C, but with the frequency of the source voltage U=Um∙sinω∙t. Such circuit oscillations are called forced. During forced oscillations, the circuit elements L, C will have, depending on the source frequency, certain inductive XL and capacitive Xc resistances and corresponding voltage drops UL, Uc across them. But the circuit has not only reactance, but also an active loss resistance R, which is basically equal to the resistance of the coil wire.
Since the voltages in the coil and capacitor are shifted relative to the current by different phase angles, they can be shown more clearly in vector diagrams (Fig. 4)
It will be interesting➡ The gimlet rule
The voltage across the inductive reactance UL leads the current by 90°, and the voltage across the capacitive reactance Uc lags the current at the same angle of 90°. And it turns out that the vectors UL and Uc are shifted by 180°, i.e. are in antiphase. The voltage vector at the source U will be equal to the geometric sum of the voltage of the vector UR and the vector of the voltage difference of the reactances UL-Uc.
As can be seen from the diagram in Fig. 4a, at UL > Uc, the external source voltage leads the current in the oscillatory circuit by an angle φ<90° and is located above the abscissa axis in the inductance voltage zone. This means that in this case the circuit has an inductive resistance. At UL < Uc (Fig. 4b), the source vector will already lag behind the current vector by an angle φ<90° and the circuit will have capacitive reactance.
The total resistance of the circuit Z will be equal to:
The amplitude value of the current Im is determined by the formula:
where Um is the amplitude voltage of the source, and ω is its angular frequency.
When equality is satisfied:
The benefits and harms of resonance
In order to draw some conclusion about the pros and cons of resonance, it is necessary to consider in which cases it can manifest itself most actively and noticeably for human activity.
Positive effect
The response phenomenon is widely used in science and technology . For example, the operation of many radio circuits and devices is based on this phenomenon.
- Two-stroke engine. The muffler of a two-stroke engine has a special shape designed to create a resonant phenomenon. It improves engine performance by reducing consumption and pollution. This resonance partially reduces the unburned gases and increases compression in the cylinder.
- Musical instruments. In the case of string and wind instruments, sound production occurs mainly when the oscillatory system (strings, columns of air) is excited until the phenomenon of resonance occurs.
- Radios. Each radio station emits an electromagnetic wave with a clearly defined frequency. To capture it, the RLC circuit is forcibly vibrated by an antenna, which captures all electromagnetic waves that reach it. To listen to one station, the natural frequency of the RLC circuit must be tuned to the frequency of the desired transmitter by changing the capacitance of the variable capacitor (the operation is performed by pressing the station search button). All radio communication systems, whether transmitters or receivers, use resonators to "filter" the frequencies of the signals they process.
- Magnetic resonance imaging (MRI). In 1946, two Americans, Felix Bloch and Edward Mills Purcell, independently discovered the phenomenon of nuclear magnetic resonance, also called NMR, which earned them the Nobel Prize in Physics.
Negative impact
However, the phenomenon is not always useful . You can often find references to cases where suspension bridges broke when soldiers walked across them “in step.” At the same time, they refer to the manifestation of the resonant effect of resonance, and the fight against it becomes large-scale.
- Motor transport. Motorists are often annoyed by noise that occurs at certain vehicle speeds or as a result of engine operation. Some slightly rounded parts of the body resonate and emit sound vibrations. The car itself, with its suspension system, is an oscillator, equipped with effective shock absorbers that prevent sharp resonance from occurring.
- Bridges. The bridge can perform vertical and transverse vibrations. Each of these types of oscillations has its own period. If the lines are suspended, the system has a very different resonant frequency.
- Building. Tall buildings are susceptible to earthquakes. Some passive devices help protect them: they are oscillators whose natural frequency is close to the frequency of the building itself. Thus, the energy is completely absorbed by the pendulum, preventing the destruction of the building.
Parallel oscillatory circuit
In a parallel oscillating circuit, the signal source is connected to an inductor and a capacitor in parallel (Fig. 11). When an alternating voltage is applied to the circuit, energy is exchanged between the capacitor and the coil, but only in the circuit inside the circuit.
For resonance to occur in it, as in a series circuit, the necessary conditions are the equality of the capacitive Xc and inductive XL resistances, as well as the equality of the natural oscillation frequency of the circuit and the oscillation frequency of the current source. Only resonance in a parallel oscillatory circuit, in contrast to resonance in a series circuit, is called current resonance.
In an ideal parallel circuit (without losses), the vectors of inductive Ic and capacitive current IL (at XL=Xc) at resonance will be directed in opposite directions and the total current will go to zero (Fig. 14a). This means that the circuit resistance will tend to infinity. But in a real parallel circuit there is a loss resistance R which is concentrated mainly in the inductance (Figure 14b) and therefore, even at resonance, the current in the circuit is no longer zero, but is equal to the active component of the current in the coil circuit - Iк=IL+IR. This means that the total resistance of the circuit Z will no longer be infinite, but equal to:
Z=L/CR.
Figure 15 shows a graph of the characteristics of the dependence of the current Ik and the impedance Z of the parallel circuit on frequency.
We can conclude: there are two currents in the parallel circuit circuit - the current from the source I flowing through the active loss resistance of the coil and the reactive circuit current Ik. A reactive current of quite large magnitude flows inside the circuit:
Iк=IQ,
but it consumes a small current from the source, which is only necessary to compensate for losses in the circuit:
I=U/Z.
The quality factor Q of a parallel circuit, in contrast to a series circuit, shows how many times the current in the circuit elements is greater than the source current consumption:
Q ≈ Iк/I.
Figure 16 gives a specific example of a parallel oscillating circuit, where it is clear that the circuit current is Q times greater than the source current.
Radio receivers also use direct connection of the oscillating circuit with the antenna, i.e. the circuit is connected in parallel to the signal source (Fig. 17). Using a variable capacitor, we tune the circuit to the signal frequency of the desired radio station. At resonance, the loop current caused by the desired radio station becomes relatively large, and the loop resistance is also large. Therefore, a significant voltage is obtained between points a and b. For other stations, the circuit represents low resistance and the radio station signal goes to ground.
Fighting resonance
But despite the sometimes disastrous consequences of the response effect, it is quite possible and necessary to fight it. To avoid the unwanted occurrence of this phenomenon, two methods of simultaneously applying resonance and combating it are usually used:
- “Dissociation” of frequencies is carried out, which, if they coincide, will lead to undesirable consequences. To do this, they increase the friction of various mechanisms or change the natural frequency of vibration of the system.
- They increase the damping of vibrations, for example, by placing the engine on a rubber lining or springs.
Examples that are repeated by many
Another example, which is even included in jokes, is the breaking of dishes by sound vibrations, from practicing the violin and even singing. Unlike a company of soldiers, this example was repeatedly observed and even specially tested. Indeed, the resonance that occurs when the frequencies coincide leads to the splitting of plates, glasses, cups and other utensils.
This is an example of process development under conditions of a high-quality system. The materials from which the dishes are made are fairly elastic media in which vibrations propagate with low attenuation. The quality factor of such systems is very high, and although the frequency coincidence band is quite narrow, resonance leads to a strong increase in amplitude, as a result of which the material is destroyed.