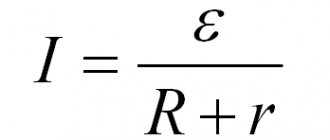OGE 2022 in physics ›
1. The change in internal energy by doing work is characterized by the amount of work, i.e. work is a measure of the change in internal energy in a given process. The change in the internal energy of a body during heat transfer is characterized by a quantity called the amount of heat .
The amount of heat is the change in the internal energy of a body during the process of heat transfer without doing work.
The amount of heat is denoted by the letter \( Q \). Since the amount of heat is a measure of the change in internal energy, its unit is the joule (1 J).
When a body transfers a certain amount of heat without doing work, its internal energy increases; if the body gives off a certain amount of heat, then its internal energy decreases.
2. If you pour 100 g of water into two identical vessels, one and 400 g into the other at the same temperature and place them on identical burners, then the water in the first vessel will boil earlier. Thus, the greater the mass of a body, the greater the amount of heat it requires to heat up. The same is true with cooling: when a body of greater mass is cooled, it gives off a greater amount of heat. These bodies are made of the same substance and they heat up or cool down by the same number of degrees.
\[Q\sim m\]
3. If we now heat 100 g of water from 30 to 60 °C, i.e. at 30 °C, and then up to 100 °C, i.e. by 70 °C, then in the first case it will take less time to heat up than in the second, and, accordingly, heating water by 30 °C will require less heat than heating water by 70 °C. Thus, the amount of heat is directly proportional to the difference between the final \( (t_2\,^\circ C) \) and initial \( (t_1\,^\circ C) \) temperatures: \( Q\sim(t_2- t_1)\).
4. If you now pour 100 g of water into one vessel, and pour a little water into another identical vessel and put in it a metal body such that its mass and the mass of water are 100 g, and heat the vessels on identical tiles, then you will notice that in a vessel containing only water will have a lower temperature than one containing water and a metal body. Therefore, in order for the temperature of the contents in both vessels to be the same, it is necessary to transfer more heat to the water than to the water and the metal body. Thus, the amount of heat required to heat a body depends on the type of substance from which the body is made.
5. The dependence of the amount of heat required to heat a body on the type of substance is characterized by a physical quantity called the specific heat capacity of the substance .
A physical quantity equal to the amount of heat that must be imparted to 1 kg of a substance to heat it by 1 ° C (or 1 K) is called the specific heat capacity of the substance.
1 kg of substance releases the same amount of heat when cooled by 1 °C.
Specific heat capacity is denoted by the letter \( c \). The unit of specific heat capacity is 1 J/kg °C or 1 J/kg K.
The specific heat capacity of substances is determined experimentally. Liquids have a higher specific heat capacity than metals; Water has the highest specific heat, gold has a very small specific heat.
The specific heat of lead is 140 J/kg °C. This means that to heat 1 kg of lead by 1 °C it is necessary to expend an amount of heat of 140 J. The same amount of heat will be released when 1 kg of water cools by 1 °C.
Since the amount of heat is equal to the change in the internal energy of the body, we can say that specific heat capacity shows how much the internal energy of 1 kg of a substance changes when its temperature changes by 1 °C. In particular, the internal energy of 1 kg of lead increases by 140 J when heated by 1 °C, and decreases by 140 J when cooled.
The amount of heat \( Q \) required to heat a body of mass \( m \) from temperature \( (t_1\,^\circ C) \) to temperature \( (t_2\,^\circ C) \), is equal to the product of the specific heat capacity of the substance, body mass and the difference between the final and initial temperatures, i.e.
\[ Q=cm(t_2{}^\circ-t_1{}^\circ) \]
The same formula is used to calculate the amount of heat that a body gives off when cooling. Only in this case should the final temperature be subtracted from the initial temperature, i.e. Subtract the smaller temperature from the larger temperature.
6. An example of solving a problem. 100 g of water at a temperature of 20 °C is poured into a glass containing 200 g of water at a temperature of 80 °C. After which the temperature in the vessel reached 60 °C. How much heat did the cold water receive and how much heat did the hot water give out?
When solving a problem, you must perform the following sequence of actions:
- write down briefly the conditions of the problem;
- convert the values of quantities to SI;
- analyze the problem, establish which bodies are involved in heat exchange, which bodies give off energy and which receive;
- solve the problem in general form;
- perform calculations;
- analyze the received answer.
1. The task.
Given: \( m_1 \) = 200 g \( m_2 \) = 100 g \( t_1 \) = 80 °C \( t_2 \) = 20 °C \( t \) = 60 °C ______________
\( Q_1 \) — ? \( Q_2 \) — ? \( c_1 \) = 4200 J/kg °C
2. SI: \( m_1 \) = 0.2 kg; \( m_2 \) = 0.1 kg.
3. Task analysis. The problem describes the process of heat exchange between hot and cold water. Hot water gives off an amount of heat \( Q_1 \) and cools from temperature \( t_1 \) to temperature \( t \). Cold water receives an amount of heat \( Q_2 \) and is heated from temperature \( t_2 \) to temperature \( t \).
4. Solution of the problem in general form. The amount of heat given off by hot water is calculated by the formula: \( Q_1=c_1m_1(t_1-t) \).
The amount of heat received by cold water is calculated by the formula: \(Q_2=c_2m_2(t-t_2)\).
5. Computing. \( Q_1 \) = 4200 J/kg · °С · 0.2 kg · 20 °С = 16800 J \( Q_2 \) = 4200 J/kg · °С · 0.1 kg · 40 °С = 16800 J
6. The answer is that the amount of heat given off by hot water is equal to the amount of heat received by cold water. In this case, an idealized situation was considered and it was not taken into account that a certain amount of heat was used to heat the glass in which the water was located and the surrounding air. In reality, the amount of heat given off by hot water is greater than the amount of heat received by cold water.
The concept of the amount of heat.
We know that the internal energy of the body can be changed in two ways.
By doing work and by heat exchange. When implementing method 2, the change in internal energy is equal to the amount of heat transferred. The amount of heat can be either positive or negative. If the internal energy of a body increases during the process of heat exchange, then the amount of heat is greater than zero. If the internal energy of a body decreases during heat exchange, then the amount of heat is less than zero. That is, the body gives off an amount of heat. From this we can conclude:
The amount of heat is that part of the internal energy that a body loses or receives during heat transfer.
EXAMPLES OF TASKS
Part 1
1. The specific heat capacity of silver is 250 J/(kg °C). What does this mean?
1) when 1 kg of silver cools by 250 °C, an amount of heat of 1 J is released 2) when 250 kg of silver cools by 1 °C, an amount of heat of 1 J is released 3) when 250 kg of silver cools by 1 °C, an amount of heat of 1 J is absorbed 4) When 1 kg of silver cools by 1 °C, an amount of heat of 250 J is released
2. The specific heat capacity of zinc is 400 J/(kg °C). It means that
1) when 1 kg of zinc is heated by 400 °C, its internal energy increases by 1 J 2) when 400 kg of zinc is heated by 1 °C, its internal energy increases by 1 J 3) to heat 400 kg of zinc by 1 °C it must be spent 1 J of energy 4) when 1 kg of zinc is heated by 1 °C, its internal energy increases by 400 J
3. When transferring an amount of heat \( Q \) to a solid body with mass \( m \), the body temperature increased by \( \Delta t^\circ \). Which of the following expressions determines the specific heat capacity of the substance of this body?
1) \( \frac{m\Delta t^\circ}{Q} \) 2) \( \frac{Q}{m\Delta t^\circ} \) 3) \( \frac{ Q}{\Delta t^\circ} \) 4) \( Qm\Delta t^\circ \)
4. The figure shows a graph of the dependence of the amount of heat required to heat two bodies (1 and 2) of the same mass on temperature. Compare the specific heat capacity values (\( c_1 \) and \( c_2 \)) of the substances from which these bodies are made.
1) \( c_1=c_2 \) 2) \( c_1>c_2 \) 3) \( c_1 4) the answer depends on the value of the mass of the bodies
5. The diagram shows the amount of heat transferred to two bodies of equal mass when their temperature changes by the same number of degrees. Which relationship is correct for the specific heat capacities of the substances from which bodies are made?
1) \( c_1=c_2 \) 2) \( c_1=3c_2 \) 3) \( c_2=3c_1 \) 4) \( c_2=2c_1 \)
6. The figure shows a graph of the temperature of a solid body depending on the amount of heat it gives off. Body weight 4 kg. What is the specific heat capacity of the substance of this body?
1) 500 J/(kg °C) 2) 250 J/(kg °C) 3) 125 J/(kg °C) 4) 100 J/(kg °C)
7. When heating a crystalline substance weighing 100 g, the temperature of the substance and the amount of heat imparted to the substance were measured. The measurement data was presented in table form. Assuming that energy losses can be neglected, determine the specific heat capacity of the substance in the solid state.
1) 192 J/(kg °C) 2) 240 J/(kg °C) 3) 576 J/(kg °C) 4) 480 J/(kg °C)
8. To heat 192 g of molybdenum by 1 K, you need to transfer an amount of heat of 48 J to it. What is the specific heat of this substance?
1) 250 J/(kg K) 2) 24 J/(kg K) 3) 4 10-3 J/(kg K) 4) 0.92 J/(kg K)
9. What amount of heat is needed to heat 100 g of lead from 27 to 47 °C?
1) 390 J 2) 26 kJ 3) 260 J 4) 390 kJ
10. Heating a brick from 20 to 85 °C requires the same amount of heat as heating water of the same mass by 13 °C. The specific heat capacity of the brick is
1) 840 J/(kg K) 2) 21000 J/(kg K) 3) 2100 J/(kg K) 4) 1680 J/(kg K)
11. From the list of statements below, select two correct ones and write their numbers in the table.
1) The amount of heat that a body receives when its temperature increases by a certain number of degrees is equal to the amount of heat that this body gives off when its temperature decreases by the same number of degrees. 2) When a substance cools, its internal energy increases. 3) The amount of heat that a substance receives when heated is used mainly to increase the kinetic energy of its molecules. 4) The amount of heat that a substance receives when heated is mainly used to increase the potential energy of interaction of its molecules 5) The internal energy of a body can only be changed by giving it a certain amount of heat
12. The table presents the results of measurements of mass \( m \), temperature changes \( \Delta t \) and the amount of heat \( Q \) released when cooling cylinders made of copper or aluminum.
Which statements correspond to the results of the experiment? Select two correct ones from the list provided. Indicate their numbers. Based on the measurements taken, it can be argued that the amount of heat released during cooling
1) depends on the substance from which the cylinder is made. 2) does not depend on the substance from which the cylinder is made. 3) increases with increasing cylinder mass. 4) increases with increasing temperature difference. 5) the specific heat capacity of aluminum is 4 times greater than the specific heat capacity of tin.
Part 2
C1. A solid body weighing 2 kg is placed in a 2 kW furnace and begins to heat up. The figure shows the dependence of the temperature \( t \) of this body on the heating time \( \tau \). What is the specific heat capacity of the substance?
1) 400 J/(kg °C) 2) 200 J/(kg °C) 3) 40 J/(kg °C) 4) 20 J/(kg °C)
What does Q depend on?
Test No. 1 : Let's take two identical vessels. Let's pour water weighing 400 g into one of them, and vegetable oil weighing 400 g into the other. Let's start heating them using identical burners. Watching thermometer readings. We see that the oil heats up faster. This means that the amount of heat depends on the temperature. Experiment 2 : Take 1 kg of water and 1 kg of sunflower oil. Let's heat both vessels by 1 degree. 4,200 J were spent on heating water. And 1,700 J were spent on heating oil. From this we can conclude: The amount of heat depends on the type of substance.
A physical quantity that shows how much heat is required to heat 1 kg of a substance by 1 degree is called the specific heat capacity of the substance.
Denoted by the letter C. Measured in J, divided by kg, multiplied by degrees Celsius.
Experiment 3: Take 400 g of water in one vessel, and 200 g of water in another vessel. Let's heat both vessels by the same number of degrees. To heat 1 vessel, more heat was required. Conclusion: the amount of heat depends on mass.
Formulation of the Joule-Lenz law
The rule was generalized and formulated based on the works of two physicists - British and Russian. Joule and Lenz derived their law almost simultaneously, but independently of each other, which is why it was named after both scientists.
The wording of the law illustrates well the following: if electricity is applied to a section of the circuit, the wire will begin to heat up. In everyday life, the thermal effect of current is observed in incandescent lamps and all electrical appliances. If you plug a device with a coil at the end of a section of circuit into an outlet, it will heat up and generate heat. For example, a welding machine connected to electricity will begin to melt the electrode, an electric kettle or boiler will heat the water, and a table lamp will fill the room with light.
Briefly, the law of James Joule and Emil Lenz can be formulated as follows: the amount of heat released when heating a semiconductor or conductor is directly proportional to a certain amount of time during which the current is applied, plus the resistance and the square of the working force of the electric current.
Q required
When heated
Formula: Q = cm (t2 -t1)
When a substance melts
Melting is the transition of a substance from a solid state to a liquid state.
Formula: Q = m * λ
.
The physical meaning of the specific heat of fusion: lambda shows how much heat is needed to melt 1 kg of a substance at the melting point.
Unit of measurement: J/kg.
The essence of the heat law
Law of Electromagnetic Induction - Formula
The scientists mentioned above (Joule Lenz) almost simultaneously (1841-1842) established the dependence of heating on current strength. For a visual experiment, you can use the following kit:
- the conductor is placed in a container of water;
- the thermometer will measure the change in liquid temperature when the circuit is connected to a power source;
- Using a voltmeter and an ammeter, the voltage and current at the control points are clarified.
A similar experiment can be reproduced in a container with a salt solution, which has a certain conductivity
According to Ohm's law, current ( I ) can be determined in terms of voltage ( U ) and electrical resistance ( R ):
I=U/R.
the work performed ( A ) as follows:
A = I * U * t = I * (I*R) * t = (U/R) * U * t = I2*R*t = (U2/R) * t.
Here t denotes the corresponding time interval.
At this stage, we should recall the first law of thermodynamics, which determines the conservation of energy in a closed system. This postulate allows us to describe the phenomenon under consideration using the created formula. This assumes that the amount of heat (Q) is equal to the work done (A). Final expression (Lenz's law):
Q = I2*R*t = (U2/R) * t = I * U * t.
The essence of the phenomenon is explained by the collision of charged particles with conductor molecules. If the sample is a solid material, we are talking about electrons and lattice components, respectively.
The amount of heat released
- When burning fuel.
- When a substance is cooled.
- When a liquid crystallizes.
- When steam condenses.
To heat bodies, the energy released during the combustion of fuel is often used.
There are different types of fuel: coal, oil, gasoline, kerosene, peat, alcohol, natural gas and so on.
The energy released during complete combustion of fuel is called the heat of combustion of the fuel.
Formula: Q = m * q
where q is called the specific heat of combustion of the fuel.
Physical meaning : q shows how much heat will be released during the combustion of 1 kg of fuel.
Units: J/kg.
When cooling substances: (The same as when heating a substance).
Formula Q = cm (t2 - t1)
When crystallizing substances: (Same as when melting a substance).
Formula Q = m * λ
What quantities are called specific
Physicists often use specific quantities because they are quite convenient for calculations.
Specific value is a value per unit of mass, length, area, or volume.
Rice. 3. Specific value is a value per unit of something (for example, mass, length, etc.)
In ordinary life, we also use specific values. For example, the price of a product is a specific value, since it is per unit of product. Knowing the quantity of goods, it is easy to calculate the total purchase price.
For example, a bun costs 20 rubles. You need to buy 3 buns. We will find the total amount of money by multiplying the price of one bun (specific value) by the number of pieces.
It is known that when fuel burns, energy is released. The specific heat of combustion and the number of kilograms of fuel burned will help calculate the released thermal energy.
Integral and differential formulas
Ohm's law in differential form
The dependencies established in the previous section are valid when the conductor is stationary. In this case, we can assume that the work of the applied external forces is spent directly on increasing the temperature. Given the given topic, the movement of charges (q) provides a potential difference that is equivalent to voltage (U = ϕ1 – ϕ2). Accordingly, A = q * (ϕ1 – ϕ2) = q * U. The charge can be expressed in terms of current:
q = I*t.
After elementary mathematical transformations, we get A = Q = I * U * t. If we take the change in heat (dQ) over a time interval (dt), we can express John Lenz's law in integral form:
dQ = I2 * R * dt.
For further discussion, it is necessary to introduce the concept of thermal power density (W). This term denotes the amount of energy that is released per unit time in a unit volume ( V ) of the control conductor:
W = Q/(V*t).
Electrical resistance can be expressed in terms of specific index ( p ):
R = p* (dL/dS),
Where:
- L – length;
- S – cross section.
By adding current density ( j = I / S = G * E ) and the concept of conductivity ( G = 1/ R ), we can write Lenz's law in differential form as follows:
W = G * E2.