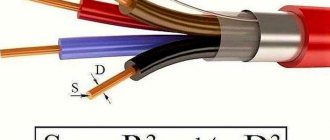
When calculating any electrical networks, such a concept as the cross-sectional area of the conductor is used. This property directly affects the safety and durability of the entire system, so it is important that the calculated cross-sectional area of the electrical conductor matches the actual one. This article will discuss methods for measuring the diameter of a conductor and its cross-section, as well as other options for determining the characteristics of a wire.
Methods for measuring wire diameter
In order to calculate the cross-sectional area of a conductor, you need to know its exact diameter. There are several ways to measure wire diameter. These include measurements:
- Using a caliper: to do this, you need to understand the principle of operation of a caliper and be able to take readings from its scales. In this case, the use of an electronic measuring device allows you to simplify measurements - it will show the exact diameter value on its screen.
- Using a micrometer: This device gives a slightly more accurate reading than a mechanical caliper, but it also requires some skill to take correct and accurate readings.
- Using a regular ruler: this method is suitable for those who do not have measuring instruments such as a caliper or micrometer in their arsenal. Measuring the conductor diameter using a ruler will not be very accurate, but it will be possible to roughly estimate the diameter.
To measure the diameter of the conductor, first of all, it is stripped of insulation with a knife or stripper. Next, if a micrometer or caliper is used, the wire core is tightly clamped between the jaws of the device and the size of the conductor is determined using the scales of the device. When using a ruler, the insulation is removed to a distance of 5-10 cm and the core is wound around a screwdriver. The turns of the conductor should be pressed tightly against each other (approximately 8-20 turns). Next, the length of the wound section is measured and the resulting value is divided by the number of turns - you get a more or less accurate diameter value.
Stereometry. Sectional area through the projected area of the section.
If the cross-section has a complex shape, then you should not try to find its area “head-on”. A smart person will go around the mountain... And we will go around: we will determine the projection area of the section (usually this is very simple) and the angle of inclination of the section plane to the plane of the base. Then we will use the well-known formula. But more on this later.
Problem 1. In a rectangular parallelepiped with edges ‚ and the points and - are the midpoints of the edges and, respectively. The plane intersects the edge at point .
a) Prove that ;
b) Find the cross-sectional area of this parallelepiped by the plane.
Figure 1 – for task 1
Let's construct a section. Let's construct a straight line - after all, the points belong to the same face. Let's construct a straight line and find the point of intersection of the straight line and the straight line - point .
Figure 2 – for task 1
This point belongs to both the face plane and the face plane. Let's draw a straight line and determine the point of intersection of this line with the edge - point.
Figure 3 – for task 1
Let's construct lines along which the section “cuts” the edges of the parallelepiped: .
Figure 4 – for task 1
Now let's construct a line and determine the point of its intersection with the line - the point of intersection lies in the plane of the upper face, and this allows us to connect it with the point. Now let's find the intersection of the segment with the edge - point, and you can outline and hatch the section:
Figure 5 – for task 1
Let us prove point a). Consider triangles and . They are similar because they are formed by parallel lines: . Since , then the coefficient of similarity of these triangles is . Then . Since the triangles and are also similar with a coefficient, then. But triangles are equal according to the 2nd criterion, therefore, , or , that is, .
b) Determine the cross-sectional area. To do this, you can determine the projection area of this section, and the cosine of the angle between the plane and the base of the parallelepiped. First, let's find the projection area. Let's cut two triangles from the base of the parallelepiped, then the remainder is the projection area (the polygon is highlighted in blue).
Figure 6 – for task 1
The area of the base of the parallelepiped is 12, cut off the triangle: according to the condition, according to the proven relation from point a). Hence,
We cut off the triangle: according to the condition, according to the proven relation from point a). Hence,
Then the projection area is equal to
Now let’s find the angle of inclination of the section plane to the plane of the base, or rather, its cosine. You need to find the cosine of an angle - or any other trigonometric function - of an angle. Consider a triangle. It is rectangular, leg (equal to the height of the parallelepiped). The length of the segment can be found from the triangle:
According to previously proven,.
Then
The cross-sectional area is
Answer: .
Problem 2. In a regular quadrangular prism, the side of the base is equal to 6, and the side edge is equal to . On the edges and the points and are marked, respectively, and .
a) Let be the point of intersection of the plane with the edge. Prove that is a square;
b) Find the cross-sectional area of the prism by plane .
Figure 1 – for task 2
Let's draw a straight line and through a point - a straight line parallel to it, since the plane cuts the opposite faces of the parallelepiped (straight prism) along parallel straight lines:
Figure 2 – for task 2
Let's find the point of intersection of the line and - point. This point belongs to the plane of the face. Therefore, it can be connected to a point by a segment that intersects the edge at point . Let's find the point of intersection of the line and - point. This point belongs to the plane of the face. Therefore, it can be connected to a point by a segment that intersects the edge at point .
Figure 3 – for task 2
Figure 4 – for task 2
By connecting the points , , , , , , we obtain the desired section.
Let us prove that it is a square.
Figure 5 – for task 2
Since the segments and belong to the same plane (sectional plane) and at the same time parallel to the planes of the upper and lower bases of the prism, they are parallel. Also .
and – diagonals of straight regular prisms with base side 1 and height . Then
It turns out - at least a rhombus. And according to the principle of a parallelogram, since the opposite sides are equal in pairs, then it is a square.
b) Determine the cross-sectional area. To do this, you can determine the projection area of this section, and the cosine of the angle between the plane and the base of the parallelepiped. First, let's find the projection area. Let's cut two triangles from the base of the parallelepiped, then the remainder is the projection area (the polygon is highlighted in purple).
Figure 6 – for task 2
The area of the base of the prism is 36, cut off the triangle: according to the condition, according to the proven relation from point a). Hence,
We cut off the triangle: according to the condition, according to the proven relation from point a). Hence,
Then the projection area is equal to
Now let’s find the angle of inclination of the section plane to the plane of the base, or rather, its cosine. You need to find the cosine of an angle - or any other trigonometric function - of an angle. Consider a triangle. It is rectangular, leg (equal to the height of the parallelepiped). The length of the segment can be found from the triangle:
According to previously proven,.
Then
The cross-sectional area is
Answer: .
How to find out the cross-section of a wire by its diameter for a stranded or segmented cable
If determining the diameter for a single-core conductor does not cause any problems, then measuring a stranded or segmented conductor can cause certain difficulties.
Measuring the cross-section of a stranded wire
When determining the core diameter of a given cable, you cannot measure this size for all wires of the core at once: the value will be inaccurate, since there is space between the wires. Therefore, this cable must first be stripped of insulation, then the stranded conductor must be fluffed up and the number of wires in the core must be counted. Next, using any method (calipers, ruler, micrometer), measure the diameter of one core and determine the cross-sectional area of the wire. After this, the resulting value is multiplied by the number of wires in the bundle and the exact size of the existing conductor is obtained.
Segment conductor measurement
Determining the dimensions of a segmented conductor is somewhat more difficult than measuring a round solid or stranded cable. In order to correctly estimate the cross-sectional area of such a conductor, it is necessary to use special tables. For example, to calculate the cross-sectional area of an aluminum conductor segment, determine the height and width of the segment and use the following table:
| Cable | Sectional area of the segment, mm2 | ||||||||
| 35 | 50 | 70 | 95 | 120 | 150 | 185 | 240 | ||
| Three-core sector single-wire, 6(10) kV | high | 5,5 | 6,4 | 7,6 | 9 | 10,1 | 11,3 | 12,5 | 14,4 |
| shire | 9,2 | 10,5 | 12,5 | 15 | 16,6 | 18,4 | 20,7 | 23,8 | |
| Three-core sector stranded, 6(10) kV | high | 6 | 7 | 9 | 10 | 11 | 12 | 13,2 | 15,2 |
| shire | 10 | 12 | 14 | 16 | 18 | 20 | 22 | 25 | |
| Four-core sector single-wire, up to 1 kV | high | – | 7 | 8,2 | 9,6 | 10,8 | 12 | 13,2 | – |
| shire | – | 10 | 12 | 14,1 | 16 | 18 | 18 | – | |
Table with formulas for the area of a circle
| Circle radius r | |
| Diameter - this is double the radius, therefore, substituting it into the formula instead of the latter, you need to | |
| source data (active link to go to the calculator) | sketch | formula |
| 1 | radius | |
| 2 | diameter | |
| 3 | circumference | |
| 4 | side of a square inscribed in a circle | |
| 5 | side of a square containing a circle | |
| 6 | sides of the triangle | Where |
| 7 | side of an equilateral triangle | |
| 8 | height of an equilateral triangle | |
| 9 | side and base of an isosceles triangle | |
| 10 | sides of a right angle of a triangle | |
| 11 | side and base of an isosceles triangle | |
| 12 | lateral sides of an isosceles triangle and the angle between them | |
| 13 | sides of a right triangle | |
| 14 | side and angle at the base of a triangle | |
| 15 | side of an equilateral triangle | |
| 16 | side and angle at the base of the trapezoid | |
| 17 | sides and diagonal of trapezoid | Where |
| 18 | sides of the rectangle | |
| 19 | side and number of sides of the polygon | |
| 20 | hexagon side |
Calculation by formula
The main geometric indicator of a conductor is its cross-sectional area. The throughput of the electrical conductor, and, consequently, its performance characteristics, which affect safety and durability, depend on this size. As mentioned above, this parameter is easily determined after measuring the diameter of the conductor. To do this, use the formula to determine the area of a circle:
Ready-made tables are a great way to quickly determine the cross-sectional area of a wire, but to be one hundred percent sure of the obtained value, it is better to check and calculate it yourself.
Application area
The circle is one of the fundamental figures that surround a person everywhere. Pipes, wheels, lamps, stove burners - all of this has the shape of a circle or a cross section in the form of a circle. Calculating the area of such a section may be necessary in the following situations:
- Determination of container volumes.
- Solving problems in strength of materials and electrical engineering.
- Calculation of the amount of materials during design, construction and repair.
- Conducting irrigated agriculture.
It is worth paying attention to the difference between a circle and a circle. A circle is a closed curve, all points of which are equally distant from the center, while a circle is a part of a plane (geometric figure) bounded by a circle.
The circle has a number of characteristics:
- radius (r/R) - a segment connecting the center of the figure with its border;
- diameter (d/D ) - a segment that connects two points on the border of a circle and passes through its center;
- circumference (C/c/L/l).
The theorem states: the area of a circle (S) is equal to the product of half the circumference and its radius. The circumference C is directly dependent on the radius R with a coefficient π (“pi” = 3.14).
Calculator for calculating wire cross-section by diameter
To quickly calculate the cross-sectional area of a round conductor, you can use a special calculator, which is designed for this purpose and is able to quickly and accurately calculate the size of the conductor using the formula indicated above.
When using this online calculator, you must accurately measure the conductor diameter for a solid conductor or one of the wires of a stranded wire using a caliper, micrometer or ruler. For a stranded conductor, you will need to additionally calculate the number of wires.
Calculation procedure
Since the main task is to find the cross-sectional area of the pipe, the basic formula will be slightly modified.
As a result, the calculations are performed as follows:
S=π×(D/2-N)2, where
D – value of the external section of the pipe;
N – wall thickness.
Please note that the more digits of π you plug into your calculations, the more accurate they will be.
Let us give a numerical example of finding the cross section of a pipe with an outer diameter of 1 meter (N). In this case, the walls have a thickness of 10 mm (D). Without going into details, let's take the number π equal to 3.14.
So the calculations look like this:
S=π×(D/2-N)2=3.14×(1/2-0.01)2=0.754 m2.
What does cross section mean?
Before revealing the basic concept, you need to decipher the meaning of the term and understand how a wire differs from a cable. A wire is a conductor that is used to connect several sections of an electrical circuit. May have one or many current-conducting conductor elements. They, in turn, can be bare, insulated, single-core or multi-core.
Conductor cut area
The former are used in overhead electrical transmission lines. The latter are used in electrical devices, panels or cabinets. In everyday life, they are located inside electrical wiring.
For your information! Insulated and single-core conductors are used everywhere, and stranded conductors are used where bends with a small radius are needed.
What is a cross section
A cross section is a figure that is formed from a conductive section by a directional plane. The area obtained from a perpendicular cut of any type of wire is indicated in square millimeters. This is an important parameter for calculating the electrical network.
Features of electrical wires
With all the variety of cable products and a huge selection of wires for laying electrical networks, there are selection rules. It is not necessary to memorize all brands of cables and wires; you need to be able to read and decipher their markings. First, it’s worth figuring out the difference between a wire and a cable.
Wire – A conductor used to connect two sections of a circuit. May have one or more conductive wires. The veins can be:
- naked;
- isolated;
- single-core;
- stranded.
Bare lines are used where touching live wires is impossible. In most cases they are used for overhead power lines.
The insulating coating is used in one or two layers. Wires that have two or three conductors in double insulation are confused with a cable. The confusion occurs due to the fact that insulation covers each core, and on the outside there is a general polymer or other coating. Such conductors are used inside electrical devices, panels or cabinets. In everyday life, they are hidden in the wall or laid in special channels.
Insulated products are used everywhere. Depending on the degree of electrical safety of the room and the installation location, the insulation class is selected.
Stranded conductors are used where small radius bends are required when laying complex routes where single-core analogues cannot pass. This type of current conductors is convenient to install in cable ducts. Single-core wires are more difficult to bend in such conditions, force must be applied, and there is a risk of damage to the wire.
For your information. Marking APPV 3*2.5 means a wire with aluminum conductors, polyvinyl chloride insulation, flat, having a dividing base. The meaning of the marking is clarified in the reference literature.
In terms of structure, a cable consists of several individually insulated strands placed in a protective outer layer of dielectric material. The space between the cores and the shell is filled with paper tapes, plastic threads or cable yarn to prevent sticking. Additionally, the product can be reinforced with armor made of tapes or steel braiding to protect against mechanical damage.
Table of correspondence of wire diameters and their cross-sectional area
You don’t always want or have the opportunity to make payments in a store or on the market. In order not to waste time on calculations or to avoid making mistakes, you can use the table for the correspondence of diameters and cross-sections of wires, which contains the most common (normative) sizes. You can rewrite it, print it and take it with you.
| Conductor diameter | Conductor cross-section |
| 0.8 mm | 0.5 mm2 |
| 0.98 mm | 0.75 mm2 |
| 1.13 mm | 1 mm2 |
| 1.38 mm | 1.5 mm2 |
| 1.6 mm | 2.0 mm2 |
| 1.78 mm | 2.5 mm2 |
| 2.26 mm | 4.0 mm2 |
| 2.76 mm | 6.0 mm2 |
| 3.57 mm | 10.0 mm2 |
| 4.51 mm | 16.0 mm2 |
| 5.64 mm | 25.0 mm2 |
How to work with this table? As a rule, the cables have a marking or tag indicating its parameters. The cable marking, number of cores and their cross-section are indicated there. For example, VVNG 2x4. We are interested in the core parameters and these are the numbers that appear after the “x” sign. In this case, it is stated that there are two conductors having a cross section of 4 mm2. So we will check whether this information corresponds to reality.
How to work with a table
To check, measure the diameter using any of the described methods, then check the table. It states that with such a cross-section of four square millimeters, the wire size should be 2.26 mm. If your measurements are the same or very close (there is a measurement error, since the devices are not ideal), everything is fine, you can buy this cable.
Stated sizes do not always correspond to real ones
But much more often, the actual diameter of the conductors is much smaller than the declared one. Then you have two options: look for a wire from another manufacturer or take a larger cross-section. Of course, you will have to overpay for it, but the first option will require a fairly long period of time, and it is not a fact that you will be able to find a cable that complies with GOST.
The second option will require more money, since the price significantly depends on the declared cross-section. Although, it’s not a fact - a good cable made according to all standards may cost even more. This is understandable - the cost of copper, and often also for insulation, subject to technology and standards, is much higher. That’s why manufacturers cheat by reducing the diameter of the wires in order to reduce the price. But such savings can turn into disaster. So be sure to take measurements before purchasing. Even trusted suppliers.
And one more thing: inspect and feel the insulation. It should be thick, continuous, and have the same thickness. If, in addition to changing the diameter, there are also problems with insulation, look for a cable from another manufacturer. In general, it is advisable to find products that meet the requirements of GOST, and not made according to specifications. In this case, there is hope that the cable or wire will serve for a long time and without problems. It's not easy to do these days, but whether you're wiring your home or running electricity from a utility pole, quality is very important. Because it’s probably worth looking for.
Determining the wire cross-section of outlet lines
When determining the wire diameter for room wiring, consider the maximum load of consumers that can be turned on at the same time. Based on this power, the cross-section of the main lines that go from the meter and input circuit breakers to the distribution boxes is selected. These are the areas that will bear the total load of all connected consumers. Choose a wire with copper conductors of at least 6 mm2.
Branch conductors from distribution boxes to sockets are selected individually for each room. This takes into account household electrical appliances that can be connected to an outlet. The cross-section of the cores is selected with a margin of one order of magnitude. This is in case there is a need to power some kind of construction tool from an outlet: a hammer drill, a welding inverter.
If the total power of consumers in the room is 4 kW, then the conductor with a copper core feeding the outlet should have a cross-section of 2.5 mm².
Attention! The cross-section of the conductor must allow it to withstand the current load and not overheat during operation of household appliances. In practice, the device with the highest power is determined and the appropriate wire diameter is selected relative to the characteristics of the device.
As a result, it turns out that the outlet conductor with copper conductors for each socket will have a cross-section of 2.5 mm2. The main wire for wiring is taken with a cross-section of 6 mm². It should be taken into account that the entire electrical wiring circuit is carried out with wires having cores of the same material. Copper and aluminum strands cannot be twisted together.