EMI filters and anti-aliasing filters for switching converters

Input EMI filters

Input and output filters are a necessary component of almost any electronic system that includes switching converters or high-speed components.
Although input EMI filters and output anti-aliasing filters serve different purposes, their configuration can be similar in some cases. The main purpose of input filters is to protect against electromagnetic interference (EMI) generated by the converter, as well as protection against possible interference from the network. In many cases, the best choice is a commercial EMI filter that provides differential and common mode filtering. These filters are installed between the mains supply and the AC/DC converter. The filters include magnetically coupled chokes and tanks. In the general case, the circuit of such a filter is shown in Fig. 1.

Rice. 1. Common mode and differential EMI filter circuit

In this EMI filter, two filters are connected in series. The near-line EMI differential noise filter consists of capacitors CY5, CY6, CX2 and a magnetically coupled two-winding LDM inductor. An EMI differential noise filter is installed in series with it, which includes capacitors CY3, CY4, CX1 and a magnetically coupled two-winding inductor LCM.

As can be seen from the figure, EMI filters for common-mode and differential noise have a similar configuration, with the exception of the location of the beginning and end of the windings of the LDM and LCM chokes. The difference is explained as follows. Differential noise currents in phase and neutral flow in different directions, and common mode noise currents in phase and neutral flow in one direction and are closed through the frame or ground. Thus, in both chokes the magnetic fluxes created by the two windings add up. Consequently, the inductance of the inductor increases and the EMI filter works like a classic LC filter.

The EMI filters described above are usually installed in 220 V mains voltage lines at the input of the AC/DC converter. EMF filters are produced by many well-known companies in the Russian electronics market, including Murata, Epcos, Würth Elektronik and many others.

The author strongly recommends using purchased filters and not trying to make them yourself from discrete components. EMI filters should not be used on AC circuits in DC circuits. Direct current will create bias in the filter chokes, and surges of interference currents lead to saturation of the inductor core, which entails a decrease in their inductance and, consequently, filtering properties.

However, it is not always possible to use ready-made EMI filters. For example, in distributed power systems in DC circuits after or before the bus converter, it may be necessary to install an EMI filter before the PoL converter. In this case, you will most likely have to create such a filter using discrete components, especially if the power of the converter in front of which the filter is installed is low.

An example is the LC filter shown in Fig. 2. Since the filter is described by a 2nd order equation and is a well-known oscillatory element, we will only present the final relations.

Rice. 2. LC-type EMI filter

The natural frequency of the oscillatory link is determined from relation (1):

ω0 = 1/√LC. (1)

The amount of damping is determined from relation (2):

β = (R/2) × (√C/L). (2)

In the diagram in Fig. 2 there is no resistor R in explicit form, therefore such a filter is called undamped, but this does not mean that R = 0 and any input influence generates undamped oscillations in the filter. The value of R is the sum of the ohmic resistance of the inductor RDC, the equivalent series resistance of the capacitor (ESR) and the resistance of the conductors. The transfer characteristic of this filter is shown in Fig. 3.

Rice. 3. Transfer characteristic of LC filter

As can be seen from this figure, the lower the damping degree β, the more pronounced the resonant peak in the frequency domain. Also, under the condition β<1, the smaller β, the more pronounced the oscillatory transient process in the time domain. In the latter case, the aphorism “the best is the enemy of the good” is quite appropriate. There are known cases where, due to the use of high-quality capacitors with very low ESR in DC buses, voltage fluctuations on the bus occurred precisely because of the low ESR value, since as ESR decreased, the degree of damping decreased and the oscillation of the transient process increased.

One more circumstance should be taken into account: the EMI filter will work as designed only if its output impedance is significantly less than the input impedance of the converter. Otherwise, connecting the converter to the filter output will noticeably distort the filter characteristics. And the filter can distort the operation of the converter.

In general, the input circuit of the converter can be represented as a sequential RLC chain. Thus, the frequency characteristic of the impedance will have an extremum in the form of a minimum. To eliminate the mutual influence of the EMI filter and the converter, it is desirable that the output impedance of the converter be an order of magnitude less than the input impedance of the EMI filter.

In Fig. Figure 4 shows an example of the frequency dependence of the impedances of the EMI filter and converter. From this figure, as well as from the above considerations, it is clear that the damping amount should not be too small. On the other hand, an excessive increase in β will eliminate oscillations, the transition process will become aperiodic and drag on over time. As a rule, the optimal β value should be selected between 0.5 and 1.0.

Rice. 4. Frequency dependences of the impedances of the EMI filter and converter

With an undamped filter (Fig. 2), the value of R in relation (2) is mainly determined by the sum of RDC + ESR, but this value is not enough to increase β to 0.7–1.0. Therefore, additional resistance must be introduced. The best option for introducing a damping chain is shown in Fig. 5. Chain Z3 consists of a resistor Rd and a capacitor Cd connected in series. The latter blocks the DC voltage and prevents additional power loss across the resistor Rd. The damping coefficient of an EMI filter with a damping chain is described by expression (3):

βd = (n/n + 1) [(Rd/2) × (√C/L)], (3)

where n = Cd/C.

Rice. 5. Damped LC filter

For practical reasons, the value of n should be in the range of 4–7 units. In Fig. Figure 6 shows the frequency dependence of the impedances of the damped and undamped filters. The resonant peak of a damped EMI filter is significantly smaller than that of an undamped one. The damping circuit can be composed of a series RL circuit and connected in parallel to the filter inductance, but this is not the best solution, in our opinion, since the power dissipation on the resistor increases.

Rice. 6. Frequency dependences of the impedances of the damped and undamped LC filter

If you turn on several LC filters in series, the slope of the frequency response of the EMI filter in the region ω>ω0 will increase, and noise filtering will improve, but, as it seems to the author, such a solution does not make sense. The dimensions of the solution will increase noticeably, and increasing the slope of the filter's frequency response will not bring practical benefits. The operating frequency of PoL converters is currently in the range of approximately 1–2 MHz.

Taking into account the requirements of the standards, the cutoff frequency of the EMI filter must be within a few kHz. If you choose a value β ≈ 1, then the attenuation at a frequency of 1 MHz will be 50–60 dB, which is quite sufficient to suppress interference. If more significant attenuation is still required, you may not want to consider using a two-stage EMI filter, but rather analyze the power supply and take other measures to reduce interference.

Improving a computer power supply

Our smaller friends (the Chinese) have flooded the electronics market, but they are not always conscientious, but many expensive models of computer power supplies are decent in their class. But still, most power supplies, as I call them, are castrated, that is, when the printed circuit board was designed for some elements, and others are soldered into it, and not all of them, especially for input filters, they are almost never found in cheap models.

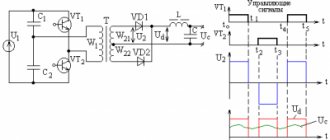
ATX block diagram

The main disadvantage of all cheap power supplies

+5V voltage oscillogram of a cheap power supply.

Output smoothing filters

The same LC filters that were discussed above are used as smoothing output filters. However, in this case, such filters cannot be replaced with purchased ones, and the developer has to calculate them each time. The output smoothing filter allows you to reduce output voltage ripple to units of mV or even several hundred μV. Reducing the ripple amplitude to tens of µV is hardly possible, even if the number of output filter stages is increased.

The parasitic components of the filter components and the conductors of the printed circuit board will interfere with the reduction of ripple. In addition, due to switching frequency jitter, low-frequency components down to several Hz may appear in the output voltage spectrum. They, of course, cannot be suppressed by an anti-aliasing filter. Thus, if it is necessary to limit the output voltage ripple down to µV, an LDO regulator is installed in the power circuit after the smoothing filter.

Rice. 7. Anti-aliasing filter at the output of the boost converter

Let's consider the most common anti-aliasing filter configuration - the π-filter (or P-filter). The diagram of its connection to the boost converter circuit is shown in Fig. 7 [1]. The resonant frequency of this filter is determined from expression (4).

Unlike the EMI filter, the anti-aliasing filter is part of the feedback loop, so the cutoff frequency of the filter should not be less than 10–20% of the switching frequency. Otherwise, the stability of the system decreases due to a delay in the feedback loop, which leads to prolongation of transient processes, as well as to deterioration of stability due to a decrease in the phase margin. As with EMI filters, a damping circuit must be included in the anti-aliasing filter. In Fig. Figure 7 shows three possible options for damping chains.

Damping option 1 with the introduction of the RFILT resistor seems to be the simplest and most economical, but the introduction of this resistor weakens the effectiveness of the filter. In addition, the impedance of the parallel RL filter circuit is reduced. Damping option 2 is the most effective because this circuit improves the filter performance, but increases the cost due to the use of a ceramic capacitor. At first glance, it may seem that damping option 3 is the most effective. However, in this case the largest capacitor capacity is required. Consequently, the cost of the solution increases. In addition, since introducing this chain will reduce the feedback loop bandwidth, this option should be excluded from consideration.

For high-frequency converters with low output current, there is another non-trivial version of the smoothing filter - instead of a choke, a resistor is used in the filter. Let's consider a simple example where an RC filter is used as the output filter of a PoL converter with a switching frequency of 2 MHz and an output current of 20 mA. Let the resistor be 10 ohms and the capacitor be 1 µF. The cutoff frequency of this filter will be about 16 kHz; Taking into account the attenuation of 20 dB/decade, we find that ripples with a frequency of 2 MHz are attenuated by more than 100 times. However, you will have to put up with a voltage drop of 200 mV across the resistor.

Note that the calculation of filters is approximate and the calculated parameters must be checked by prototyping the filter together with the converter. The value of the filter capacitance is affected by the charge voltage, current pulsation frequency, and capacitance temperature. The inductance of the filter choke depends nonlinearly on the current. In addition, the converter will also affect the filter characteristics. These changes cannot be taken into account in practical calculations. Assistance in filter development is provided by proprietary CAD systems for calculating filters. For example, a scheme for calculating an anti-aliasing filter can be found in [1]. To calculate the EMI filter, you can use the tools of [2].

Accepted notations

When analytically analyzing processes, the following quantities are used:

Un

,
In
—effective values ​​of load voltage and current;

Udmin

,
Udnom
,
Udmax
- minimum, rated and maximum voltage of the power source;

Zn

, φ - resistance and angle of active-inductive load;

f

= 1/
T
,
fн
= 1/
h
- output and carrier frequencies (
T
,
h
- their periods);

Ω = f

/
fн
- relative output frequency;

x̄L

= 2π
fL
/
ZН
,
ȳC
= 2π
fCZН
- relative resistance of the inductor and conductivity of the filter capacitor at the fundamental frequency;

α, β, χ = α + β - angles between the main harmonics of voltages and filter currents (according to the vector diagram in Fig. 2b);

n

— transformation ratio;

M

— maximum depth of modulation of PWM pulses;

f

(
t
),
f̂
(
t
),
f̃
(
t
) — function, its main and pulsation components;

F

(
t
) is the switching function.

Rice. 2. a) Equivalent circuit LC filtering b) vector diagrams

Relative values ​​of currents and voltages are indicated by a dash above, amplitude values ​​- by the index “ m

", maximum - with the index "max", and ripples - with the index "P".
Effective values ​​of load voltage and current were chosen as basic values ​​for relative voltages and currents. The analysis partially takes into account the direct voltage drops on the valves of the inverter circuit (transistor - Δ UVT
, diode - Δ
UVD
). The remaining elements of the circuits are assumed to be ideal.

"Local averaging" approach

The approach used to analyze processes in PWM inverters is based on two widely used calculation methods. It can be interpreted as a specification of the fundamental harmonic method for this type of converters [7] and as a kind of “modernization” of the method of switching functions [11].

The time functions in this case are distorted by ripple, but have either a clearly sinusoidal base of the output frequency (filter currents) or almost sinusoidal (output voltage). The filter analysis is performed according to the following general scheme: the voltages and currents of the fundamental frequency elements are determined; current ripples are integrally calculated, and from them - the ripple and non-sinusoidal coefficient of the output voltage; The “superposition” of individual components determines the total currents and voltages of the components, and from them the currents of the inductor, capacitor, etc.

In Fig. Figure 2a shows a circuit diagram of a filter excited by an equivalent PWM voltage source v

(
t
), and the harmonics of the fundamental (output) frequency dominate in the currents and voltages of the elements.
This allows us to represent the time functions fi
(
t
) as the sum of the fundamental and pulsation components:

The essence of the “local averaging” approach is as follows. If the load voltage is considered constant within the period of the carrier frequency, then it is possible to determine the parameters of the filter current ripple, which has a triangular shape. These parameters depend on the duty cycle of the PWM pulses and change from cycle to cycle. For relatively large ratios of carrier and output frequencies, which is usually the case in practice, the average value of the PWM pulse is simplified as a sinusoidal function of the output frequency and an amplitude proportional to the modulation depth M

.
Thus, the modulation envelope is “attributed” to the ripple current of the inductor (capacitor) of the filter, and it is possible to write a final and fairly accurate expression as a function
of M. As a result, closed analytical expressions are obtained for the resulting currents of the filter elements. Next, the calculation is carried out by operating with them.

Mains voltage rectifier: the most popular design

Rule No. 3: after the output from the filter, the voltage is supplied to the rectifier circuit , which in the basic version consists of a diode bridge and an electrolytic capacitor.

During the electrical conversion, the shape of a sine wave, consisting of half-waves of opposite signs, first changes to a signal in a positive direction after the diode assembly, and then these pulsations are smoothed out to an almost constant amplitude value of 311 volts.

Such a network voltage rectifier is included in the operation of all power supplies.

Calculation of the resulting quantities with PWM-2

For the effective value of the total inductor current it is easy to obtain

The inductor current ripple flows entirely through the capacitor, so

For the effective value of the total capacitor current, we obtain

As follows from Fig. 5, the capacitor voltage ripple changes parabolically, with the amplitudes of both parabolas in k

th cycle are defined as follows:

where USPm

is the maximum pulsation amplitude that occurs at γ
k
= 0.5.

Rice. 5. Diagrams of current and voltage ripple of the filter capacitor

If we determine the effective value of the parabolic function (19) for k

th cycle and geometrically subtract the pseudo-constant component from the last one (determined by integrating the pulsation), then for the effective value of the entire pulsation we obtain:

In relative units, this is the non-sinusoidal coefficient ξ:

Theoretically M

varies within the range 1≥
M
≥0, and, as follows from (20), ξ varies in the range ξ = (0.4346–0.7303)
USPm
.
Practically M
= 0.85–0.95 and ξ = (0.4499–0.4866)
ŪSPm
.
If we calculate ξ from the maximum pulsation, as is usually done in practice, then we would get 0.7071 ŪSPm
, i.e. the error would be 31.2–36.4%, which is significant. Considering the importance of ξ for calculating filter parameters, formula (20) can be considered a good support in favor of the analysis approach used here.

Transformation ratio n

and the maximum modulation depth
M
depend on the inverter switch circuit. In particular, for the switch bridge circuit we have:

where t̄kmax

=
tkmax
/
h
- maximum relative switching time.

Calculation of fundamental harmonics

When calculating the fundamental harmonics, the load voltage and current are assumed to be sinusoidal. From the vector diagrams (Fig. 2b) you can find the voltages and currents of the filter elements in relative units:

The time functions of the fundamental harmonics are expressed as:

where V1m

is the amplitude of the fundamental harmonic of PWM pulses at the filter input, and the amplitudes
UНm
,
UL1m
,
IL1m
and
IC1m
are determined by multiplying the corresponding effective values ​​by a factor of √2.

Radiated Interference Measurement

The method for measuring radiated interference above 30 MHz is described in the IEC reference standard CISPR16–2-3. For these types of measurements, an anechoic room with a conductive floor or an anechoic chamber is typically used. Again, the device under test is placed on a non-conductive table (for portable or tabletop devices) or on the floor. In order to be able to rotate the device under test around its own axis in its original state during measurements, it is placed on a turntable (Fig. 10). In relatively large anechoic rooms, the receiving antenna is installed at a distance of 10 m from the device under test at a height that corresponds to the maximum magnitude of the electric field at each measurement frequency. Additionally, the orientation of this antenna changes to provide horizontal and vertical polarization. In small anechoic chambers, the distance between the antenna and the device under test is 3 m. The height of the antenna should be fixed, and the floor between the device under test and the antenna should be covered with absorbent material.

Rice. 10. Installation for measuring radiated interference in anechoic rooms or chambers

Step response

The output voltage must remain stable when the voltage at the input of the pulse converter changes.
Likewise, if the output current suddenly decreases or increases, the output voltage must be quickly adjusted. Figure 10 shows the transient response of a variable stability switching controller (yellow curve) with an output voltage of 5 V when the load current suddenly changes from 0 to 1 A (green curve). Rice. 10. Transient response of a stable pulse controller

When the load current suddenly changes, the control circuit must quickly adjust the output voltage to the specified level. The output voltage should not change significantly as a result of the surge response, otherwise electronic circuits may be damaged due to overvoltage. Ideally, after a load surge, the output voltage is quickly adjusted to the set value; there are no emissions or even ringing. Ringing at the compensation stage is due to unstable operation of the pulse converter. The operation of a pulsed power supply controller is considered stable if it responds quickly enough to abrupt changes in the load and promptly compensates for this change.

Measurements according to IEC CISPR 22 standard

The measurements discussed above were performed according to IEC CISPR16–2-1 standard. Thanks to the line impedance stabilization circuit, the asymmetric voltage was isolated and equalized to the common mode voltage, which was then compared with the limit value of the IEC CISPR 22 standard for residential and commercial devices (Class B). There are no directly applicable EMC standards for power supply components (including switching controllers of all types).

The entire system in which the switching converter is used must be rated and tested in accordance with the standards that apply to the product or family of products. In the example considered, the IEC CISPR 22 standard for IT applications was taken as a basis. The limit values ​​from CISPR 22 are also specified in the general standard IEC 61000-6-3. In cases where there are no device-specific standards, general standards apply.

conclusions

1. Consideration of the parameters of the output smoothing LC filter in conjunction with other main characteristics of the inverter and taking into account the specific features of PWM allows you to optimize the choice of filter.
2. The use of the “local averaging” apparatus in combination with the methods of the fundamental harmonic and switching functions allows for a comprehensive analysis of the processes in the output filter of PWM inverters, obtaining compact and at the same time quite accurate analytical expressions for calculating and selecting parameters.
3. The parameters can be calculated directly using the analytical relationships given here, the accuracy of which for most values ​​is not lower than 1%, and in some cases - not lower than 2–3%. In more critical cases, you can use the results presented.
4. All other things being equal, the non-sinusoidal coefficient of the inverter output voltage in the practical range of modulation depth ( M
   = 0.85–0.95) with PWM-2 is approximately 1.8 times greater than with PWM-3.

Literature

1. Bareghamyan G.V. Research of a transistor converter with a sinusoidal output voltage // Abstracts of the report at the All-Union Scientific and Technical. conf. “Application in technological processes of mechanical engineering production of semiconductors. convert. frequencies". Ufa. 1980.
2. Bareghamyan G.V. Selection of parameters of the LC filter of a transistor inverter with PWM and sinusoidal output voltage // Abstracts of the report at the Interdepartmental Scientific and Technical. meeting "Problems of electromagnetic compatibility of power semiconductor converters." Tallinn. 1982.
3. Bareghamyan G.V., Margaryan V.V., Simonyan G.R. Calculation of the output LC filter of an inverter with PWM // Dep. in ArmNIINTI 06/08/98. No. 85-Ar98. Yerevan. 1998.
4. Bareghamyan G.V. Automation of calculation of an inverter with a three-level PWM // Modeling, optimization, control: Collection of scientific papers / SEUA. Yerevan. 2001. Issue. 4.
5. Bareghamyan G.V. Automation of calculation of inverters with sinusoidal output voltage and two-level pulse-width modulation // Izv. NAS RA and SEUA. TN series. 2001. T. 55. No. 3.
6. Bareghamyan G.V. Fundamentals of the synthesis of stabilized transistor converters with pulse-width modulation: Dis. for the competition for Doctor of Technical Sciences. Sci. Yerevan, 2006.
7. Kovalev F.I., Mustafa G.M. Using the first harmonic method in the design of stabilized inverters // Electrical engineering. 1982. No. 3.
8. Moin V.S. Stabilized transistor converters. M.: Energoatomizdat. 1986.
9. Mostkova G. P., Tolkachev A. I. Calculation of output filters of autonomous inverters // Elektrotekhn. industry. Ser. Convert technique. 1971. No. 22.
10. Mustafa G. M. Calculation of pulse-modulated inverters with sinusoidal output voltage // Electrical engineering. 1988. No. 2.
11. Rudenko V. S., Senko V. I., Chizhenko I. M. Fundamentals of converter technology. M.: Higher school. 1980.
12. Adamiya G. G., Berkovich E. I., Kartavykh A. S. et al. Static uninterruptible power supply units. M.: Energoatomizdat. 1992.

Reducing pulsations

In practice, an LC low-pass filter is typically used to reduce residual ripple to a level of a few mV and suppress high-frequency components.
Figure 5 shows the circuit of such a filter, which can be implemented using an unshielded WE-PD2 inductor and a standard electrolytic capacitor. Rice. 5. Simple Low Pass Filter Circuit

Rice. 6. Two-stage output filter

If interference should be completely absent at the output, a low-pass filter is used in the second stage along with an LC low-pass filter (see Fig. 6). An inexpensive two-stage filter can be implemented using the WE-PD2 choke and WE-MPSB series SMD ferrite.

Components LFILTER and CFILTER1 work as a low-pass filter, which filters the clock signal of the switching power supply controller and suppresses harmonic oscillations. Next, the RF components of the controller's output voltage are converted into heat using an SMD ferrite bead, and CFILTER2 attenuates their amplitude. This type of output filter reduces residual ripple to a few mV and provides power to even sensitive analog circuits.

Calculation of ripple components with PWM-3

With three-level PWM (PWM-3), a voltage with levels ± Vm

, 0. Having performed similar steps to derive formulas (4)–(12), we obtain the following expressions:

In this case, the coefficient γ is the module of the switching function F

(ϑ), i.e.

Then, taking into account (22), for the main component of the inductor current ripple we have

For the effective value of this pulsation one can obtain

It turns out that for the same M

= 0.85–0.95 we have
ĪLП
≈ (0.3928–0.4365)
ĪLPm
, i.e., with the same current ripple amplitudes, its effective value with PWM-3 is approximately 3–5% greater than with PWM-3 2.