Potential difference
Voltage on a non-uniform section of the circuit
(where there are external forces) is equal to the sum of the source emf and the potential difference in this area:
For a homogeneous chain section
, where outside forces do not act,
Those. the voltage coincides with the potential difference at the ends of the circuit section
.
- Ohm's law for a homogeneous section
of a circuit in integral
and differential
form
. Resistance and its dependence on temperature. Superconductivity.
Ohm's law for a homogeneous section
of a chain in integral
and differential
form
Ohm's law for a homogeneous section of a chain: n
German physicist Georg Ohm experimentally established that the current strength in a circuit is directly proportional to the applied voltage and inversely proportional to the resistance of the conductor:.
Ohm's law in differential form (Ohm's law for current density).
Ohm's Law form applies to the entire conductor.
Let us present Ohm's law in differential (i.e., relating to a current element of length dl
) form.
A certain point inside the conductor is characterized by the current density vector, the electric field strength, and the properties of the conductor material, i.e. specific resistance. Let us mentally select a small volume near the point in question and substitute it into Ohm’s law, we obtain: ,
here is the potential difference between cross sections
dS
separated at a distance
dl
. Hence,.
Let's take into account that - electrostatic field strength; - electric field density; - electrical conductivity.
Then formula (20) implies Ohm’s law in differential form
: .
Example of outside forces
The simplest diagram of a source of external force (current source), which is of mechanical origin, is shown in Fig. 1.
Figure 1. Diagram of a third-party force source. Author24 - online exchange of student work
Finished works on a similar topic
Course work Third-party forces and EMF 400 ₽ Abstract Third-party forces and EMF 240 ₽ Test work Third-party forces and EMF 250 ₽
Receive completed work or specialist advice on your educational project Find out the cost
Let there be an electrically neutral medium with equal charges of opposite sign between electrodes A and B (Fig. 1). An external force of non-electrical origin moves positive charges to electrode B (this electrode is positively charged), and negative charges to electrode A (negatively charged electrode). An electric current flows in an external circuit. Current produces work. The energy that is necessary to produce such work is supplied by external forces, which spend it on separating charges between the electrodes. The current inside the external power source short-circuits the current in the external circuit. The direction of the electric current in the external circuit is from the positive electrode to the negative, and vice versa inside the current source. A practical implementation of such a scheme is an electrostatic machine.
EMF and Ohm's law[ | ]
The electromotive force of the source is related to the electric current flowing in the circuit, the relationships of Ohm's law. Ohm's law for a non-uniform section of a circuit
has the form[1]:
φ 1 − φ 2 + E = IR , {\displaystyle \varphi _{1}-\varphi _{2}+{\mathcal {E}}=IR,}
where φ 1 − φ 2 {\displaystyle \varphi _{1}-\varphi _{2}} is the difference between the potential values at the beginning and end of the circuit section, I {\displaystyle I} is the strength of the current flowing through the section, and R {\displaystyle R } is the resistance of the section.
If points 1 and 2 coincide (the circuit is closed), then φ 1 − φ 2 = 0 {\displaystyle \varphi _{1}-\varphi _{2}=0} and the previous formula turns into the Ohm’s law formula for a closed circuit
[1]:
E = IR , {\displaystyle {\mathcal {E}}=IR,}
where now R {\displaystyle R} is the total
resistance of the entire circuit.
In general, the total resistance of a circuit consists of the resistance of the section of the circuit external to the current source ( R e {\displaystyle R_{e}} ) and the internal resistance of the current source itself ( r {\displaystyle r} ). Taking this into account, you should:
E = IR e + I r . {\displaystyle {\mathcal {E}}=IR_{e}+Ir.}
How is Ohm's law formulated for a closed circuit?
Ohm's law for a complete closed circuit is formulated as follows: the current in a closed circuit is directly proportional to the emf in the circuit and inversely proportional to the total resistance of the circuit. Total resistance means the sum of external and internal resistance.
Interesting materials:
How to write length in mathematics? How to record a telephone conversation on an iPhone? How to record a telephone conversation on iPhone 2021? How to record internal sound on Android? How are vector coordinates written? How to write a date in Excel? How to schedule a message in a telegram? How to fill out the place of birth in the application form? How to fill out the first part of the application for participation in the auction? How to refuel at a Tatneft self-service gas station?
Zero level
Students in grades 7-9 sometimes encounter the concept of emf in problems. And immediately the question: “What is this?”
If you pick up any current source: a battery (voltaic cell), power supply, etc., you will see, for example, the inscription “4.5 V” on it. You call this source voltage. But in fact, this is EMF - electromotive force. Symbolized as ℰ, measured in volts (V).
If the electrical resistance of the source can be neglected (i.e., the problem statement does not say anything about this resistance or it is written that the source is ideal), then the emf and voltage of the source are equal.
Thus,
EMF is one of the characteristics of a current source
.
Usually this is enough to solve problems in grades 7-9.
Definition and physical meaning
Applying some potential difference between the two ends of a conductor will create a flow of electrons from one end to the other. But this is not enough to maintain the flow of charges in the conductor. The drift of electrons leads to a decrease in the potential until it balances (the current stops). Thus, to create a direct current, mechanisms are needed that continuously return the described system to its original configuration, that is, preventing the aggregation of charges as a result of their movement. For this purpose, special devices called power supplies are used.
To illustrate their operation, it is convenient to consider a closed loop of resistance and a galvanic power source (battery). If we assume that there is no current inside the battery, then the described problem of combining charges remains unresolved. But in a circuit with a real power source, electrons are constantly moving. This occurs due to the fact that the flow of ions also flows inside the battery from the negative electrode to the positive one. The energy source that moves these charges in the battery is chemical reactions. This energy is called electromotive force.
EMF is a characteristic of any energy source capable of controlling the movement of electrical charges in a circuit. In analogy with a closed hydraulic circuit, the work of the energy source. d.s. corresponds to the operation of the pump to create water pressure. Therefore, the symbol representing these devices is indistinguishable on hydraulic and electrical diagrams.
Despite the name, electromotive force is not actually a force and is measured in volts. Its numerical value is equal to the work done to move a charge along a closed circuit. The source emf is expressed by the formula E=A/q , in which:
- E—electromotive force in volts;
- A is the work of external forces to move the charge in joules;
- q is the displaced charge in coulombs.
From this EMF formula it follows that the electromotive force is not a property of the circuit or load, but is the ability of the electricity generator to separate charges.
Non-electrostatic nature of EMF[ | ]
Inside the EMF source, current flows in the opposite direction to normal.
This is impossible without an additional force of a non-electrostatic nature that overcomes the force of electric repulsion. As shown in the figure, an electric current, the normal direction of which is from “plus” to “minus”, that is, from the positive pole of the current source to the negative pole, inside the EMF source (for example, inside galvanic cell) flows in the opposite direction. The direction from “plus” to “minus” coincides with the direction of the electrostatic force acting on positive charges. Therefore, in order to force the current to flow in the opposite direction, an additional force of a non-electrostatic nature is needed (centrifugal force, Lorentz force, forces of a chemical nature, force from the vortex electric field) that would overcome the force from the electrostatic field. Dissipative forces, although they oppose the electrostatic field, cannot force the current to flow in the opposite direction, therefore they are not part of the external forces whose work is used in determining the emf.
Induction EMF[ | ]
The cause of the occurrence of an electromotive force in a closed loop can be a change in the magnetic field flow penetrating the surface limited by this loop. This phenomenon is called electromagnetic induction. The magnitude of the induced emf in the circuit is determined by the expression
E = − d Φ dt , {\displaystyle {\mathcal {E}}=-{\frac {d\Phi }{dt}},}
where Φ {\displaystyle \Phi } is the magnetic field flux through the specified surface. The “−” sign before the expression shows that the induced current created by the induced emf prevents a change in the magnetic flux in the circuit (see Lenz’s rule). In turn, the cause of a change in magnetic flux can be either a change in the magnetic field or the movement of the circuit as a whole or its individual parts.
Electromotive force. Ohm's law for a complete circuit
As you know, for an electric current to exist, an electric field must be present. Moreover, this field must be constantly maintained by some current source. Today we will talk about the main characteristic of a current source, which is called electromotive force (or, in short, EMF). First, let's consider a simple experiment: take two oppositely charged balls and connect them with a conductor. In this case, an electric current will arise in the conductor, but it will be very short-lived. The fact is that very soon a redistribution of charge will occur, and the potentials of the balls will be equalized. This means that the electric field will cease to exist.
From this we can conclude that in order to maintain a constant current, the presence of certain forces of non-electric origin is necessary so that these forces can move charges against the field. Such forces are called external forces. That is, third-party forces are any forces that act on electric charges, but are not forces of electrical origin
. For example, these could be forces acting on charges from a magnetic field - this is used in generators.
In batteries or accumulators, the work of separating electrical charges is done by chemical reactions.
Another argument that we can give is that the work done by Coulomb forces when moving a charge along a closed loop is zero
. This means that some other forces must provide non-zero work to maintain the potential difference.
A device for maintaining electric current is called a current source.
In any current source, external forces act on the charges, doing work against the Coulomb forces.
Therefore, the characteristic of the source must be a value independent of the magnitude of the charge. This quantity is called electromotive force. The electromotive force is equal to the ratio of the work of external forces when moving a charge along a closed circuit to the magnitude of this charge:
From the formula it is clear that the electromotive force, like voltage, is measured in volts:
Now that we are familiar with EMF, we can move on to studying Ohm's law for a complete circuit. A complete circuit is a closed circuit that includes a current source.
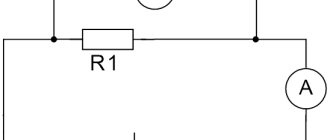
For convenience, we will consider the simplest electrical circuit, consisting only of a current source, a resistor and connecting wires:
As we have already said, a current source is characterized by an emf. However, any current source has a certain resistance, which is called internal resistance
. Ohm's law for a complete circuit represents the relationship between emf, internal and external resistance, and current in the circuit. In order to establish this connection, we use the law of conservation of energy. Let us write that the work of external forces is equal to the product of the source emf and the magnitude of the charge:
As you know, each section of the circuit emits a certain amount of heat. According to the Joule-Lenz law, this amount of heat is calculated by the formula:
Based on the law of conservation of energy, we can equate this amount of heat to the work of external forces:
Ohm's law for a complete circuit sounds like this: the current strength in a closed circuit is equal to the ratio of the source emf to the total resistance of the circuit:
Ohm's law can be derived for a complete circuit using slightly different reasoning. As we know, with a series connection, the total voltage of the circuit is equal to the sum of the voltage drops in all sections of the circuit:
We see that the product of current and resistance of a resistor is nothing more than the voltage across this resistor. And the product of current and internal resistance is the voltage drop across the source itself:
It must be said that the internal resistance of the source in many cases is negligible compared to the resistance of the external part of the circuit. In this case, we can assume that the voltage at the source terminals is approximately equal to the EMF (that is, the voltage drop across the source is considered approximately zero):
However, it is the internal resistance that determines the current strength in the circuit during a short circuit. Let us recall that during a short circuit, the external resistance becomes almost zero, so the current in the circuit increases sharply:
Let us now consider a circuit containing several current sources connected in series.
In this case, the EMF of the entire circuit is equal to the algebraic sum of the EMF of individual sources.
In such cases, it is necessary to select the so-called “current bypass direction”. This direction is chosen conditionally (in our case, counterclockwise). Then, because they tend to induce current in the bypass direction.
A, because they tend to cause a current in the direction opposite to the direction of bypass. Negative EMF means that external forces inside the source do negative work. Thus, the EMF of our circuit will be equal to:
In accordance with the rules of series connection, the total resistance of the circuit is equal to the sum of the external resistance and the internal resistances of all current sources:
An example of solving a problem.
Task.
A resistor with a resistance of 15 Ohms is connected to a current source with an internal resistance of 1 Ohm. After this, an ammeter was connected to the circuit, which showed that the current strength was 5 A. Find the work done by external forces inside the source in 2 minutes.
Mechanical analogy of an electrical circuit
To better understand the significance of a current source in a closed electrical circuit, consider the following mechanical analogy. Figure 2.48 shows a closed loop consisting of pipes and a pump. To exclude the effect of gravity, we assume that the contour is horizontal. The entire circuit is filled with liquid, such as water. In any section of a horizontal pipe, liquid flows due to the pressure difference at the ends of the section. The liquid moves in the direction of decreasing pressure. But the pressure force that appears due to the compression of a liquid is a type of elastic force that is potential. Therefore, the work of these forces on a closed path, like the work of Coulomb forces, is equal to zero. Consequently, these forces alone cannot cause long-term circulation of liquid in a closed circuit, since the flow of liquid is accompanied by energy losses due to the action of friction forces.
To circulate water, a pump is needed - an analogue of a power source. The impeller of this pump acts on liquid particles and creates a constant pressure difference (pressure) at the inlet and outlet of the pump, due to which the liquid flows through the pipes. The role of external forces in the pump is played by the force acting on the water from the rotating impeller. Inside the pump, water flows from areas of lower pressure to areas of higher pressure.