Electricity
— directed movement of charged particles in an electric field.
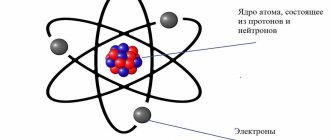
Charged particles can be electrons or ions (charged atoms).
An atom that has lost one or more electrons acquires a positive charge. — Anion (positive ion). An atom that has gained one or more electrons acquires a negative charge. — Cation (negative ion). Ions are considered as mobile charged particles in liquids and gases.
In metals, charge carriers are free electrons, like negatively charged particles.
In semiconductors, the movement (movement) of negatively charged electrons from one atom to another and, as a result, the movement between the atoms of the resulting positively charged vacancies - holes - are considered.
For the direction of electric current
the direction of movement of positive charges is conventionally accepted. This rule was established long before the study of the electron and remains true to this day. The electric field strength is also determined for a positive test charge.
For any unit charge q
in an electric field of intensity
E,
there is a force
F = qE
, which moves the charge in the direction of the vector of this force.
The figure shows that the force vector F— = -qE
, acting on the negative charge
-q
, is directed in the direction opposite to the field strength vector, as the product of the vector
E
by a negative value. Consequently, negatively charged electrons, which are charge carriers in metal conductors, actually have a direction of movement opposite to the field strength vector and the generally accepted direction of electric current.
Charge quantity Q
= 1 The pendant moved through the cross section of the conductor in time
t
= 1 second is determined by the current value
I
= 1 Ampere from the relation:
I = Q/t
.
Current ratio I
= 1 Ampere in the conductor to its cross-sectional area
S
= 1 m 2 will determine the current density
j
= 1 A/m2:
j = I/S
Job A
= 1 Joule spent on transporting the charge
Q
= 1 Coulomb from point 1 to point 2 will determine the value of the electrical voltage
U
= 1 Volt, as the potential difference
φ
1 and
φ
2 between these points from the calculation:
U
=
A/Q
=
φ
1 -
φ
2
Electric current can be direct or alternating.
Direct current is an electric current whose direction and magnitude do not change over time.
Alternating current is an electrical current whose magnitude and direction changes over time.
Back in 1826, the German physicist Georg Ohm discovered an important law of electricity, which determines the quantitative relationship between electric current and the properties of a conductor, characterizing their ability to withstand electric current. These properties subsequently began to be called electrical resistance, denoted by the letter R
and measured in Ohms in honor of the discoverer.
Ohm's law in its modern interpretation by the classical U/R ratio determines the amount of electric current in a conductor based on the voltage U
at the ends of this conductor and its resistance
R
:
I = U/R
Content
- 1 Ohm's Law
- 2 How electrons move in a conductor
- 3 When is Ohm's law valid?
- 4 Semiconductors in a strong electric field
- 5 Gann effect
- 6 Violation of Ohm's law at high currents
“Be alert!”—use this advice from Kozma Prutkov more often when it comes to laws. In particular, about the laws of physics. Let us recall, for example, Ohm's law: current is proportional to voltage. It turns out that this is not the case. And good! If this law were always observed, we would be left without many electrical and radio devices. Fortunately, Ohm's law, like most laws of physics, has a limited range of applicability. It is outside the scope of this law that interesting physical phenomena arise that ensure the operation of these devices. These phenomena in themselves are very interesting, but today we will discuss another question: why is Ohm’s law violated?
Popular misconceptions about the speed of light
Another example of such a superficial perception is the concept of the nature of lightning. How many people think about what physical processes occur during a thunderstorm? For example, what is the speed of lightning? Is it possible to find out at what height lightning strikes rage without instruments? Let's deal with all this in order.
Someone might say that lightning strikes at the speed of light, and they would be wrong. A flash caused by a giant electrical discharge in the atmosphere spreads so quickly, but lightning itself is much slower. A lightning bolt is not a laser-like beam of light, although it is visually similar. This is a complex structure in an atmosphere saturated with electricity.
The stepped leader or main lightning channel is formed in several stages. Each step, tens of meters long, is formed at a speed of about 100 km/sec along the discharge threads of ionized particles. The direction changes at each stage, so the lightning looks like a sinuous line. 100 kilometers per second is fast, but it is very far from the speed of an electromagnetic wave. Three thousand times.
Ohm's law
Let's connect the conductor to the electrical circuit and measure the current I
, flowing through the conductor, at different values of the applied
voltage
U. Thus, we obtain the dependence I
=
I
(
U
) - the current-voltage characteristic of the conductor.
According to Ohm's law, the current strength is directly proportional to the applied voltage, i.e. the current-voltage characteristic is a linear function \(~I(U) = \frac UR,\)
and resistance R
not depend on
U. If this is not the case (Ohm’s law is not satisfied), then the current-voltage characteristic is nonlinear.
The simplest example of a conductor in which Ohm's law is violated is the filament of an incandescent lamp. For a 40 W lamp, the current-voltage characteristic is shown in Figure 1. There is a linear section only at U
< 5 V, and at large values of
U
the current
I
grows more slowly than in the case of a linear dependence of
I
(
U
).
It's not hard to guess why this happens. As the voltage increases, the coil heats up and its resistance increases. This example illustrates the general rule: Ohm's law is valid only for sufficiently small I
and
U
, and at high currents and voltages it is violated.
Rice. 1
Let's write Ohm's law in a different form. To do this, we introduce the current density value \(~j = \frac IS\), where S
- cross-sectional area of the conductor.
Then \(~j = \frac IS = \frac{U}{RS} = \frac{U}{\frac{\rho L}{S} S} = \frac{1}{\rho} \frac UL = \sigma E.\)
Here ρ
is the resistivity of the conductor, the value \(~\sigma = \frac{1}{\rho}\) is called specific conductivity,
L
is the length of the conductor, \(~E = \frac UL\) is the electric field strength.
Ohm's law assumes a linear relationship between current density j
field
strength
E. If conductivity a for some reason depends on the magnitude of the electric field, then the dependence of j
on
E
becomes nonlinear, and Ohm’s law is violated.
To find out the reasons for the violation of Ohm's law, consider the movement of electrons in conductors in the absence and presence of an electric field.
How do electrons move in a conductor?
Many substances that conduct electricity are crystalline. The atoms of which they are composed do not occupy random positions, but form a structure that periodically repeats in space—a crystal lattice.
In conductors, some of the atoms are ionized, and the electrons detached from them can move along the conductor. The concentration of n such electrons (they are called conduction electrons) depends on the type of conductor. In metals, the concentration of conduction electrons does not depend on temperature. In copper n
= 8.4·1028 m-3.
In semiconductors n depends on temperature. At T
= 300 K in germanium
n
= 2.4·1019 m-3.
It may seem that the electron is “squeezing” through the crystal with great difficulty, every now and then bumping into atoms. But this is not true at all. From quantum theory it follows that due to the strictly periodic arrangement of atoms, electrons will move straight through an ideal lattice. In this way, conduction electrons resemble free electrons in a vacuum. And just as in the case of electrons in a vacuum, the movement of electrons in a crystal can be described using Newton’s II law - F
=
m
*
a
, only the mass
m
* in this notation (it is called the effective mass) differs from the mass
m
e of an electron in vacuum.
This difference reflects the interaction of the conduction electron with the crystal lattice. Since the lattice structures are different in different conductors, the effective electron masses in them will also be different. In this case, m
* can be either greater or less than
m
e.
Real conductors are never ideal crystals. They always contain violations of the periodic arrangement of atoms. For example, atoms of a foreign substance - an impurity - accidentally fall into some places in the lattice. Having collided with such an impurity, electrons are scattered, i.e., they change the direction of their movement. Thermal vibrations of lattice atoms (their deviations from equilibrium positions) disrupt periodicity, and this also leads to electron scattering. The average time between collisions during which the electron moves rectilinearly is called the free path time τ
.
The time τ
depends on the speed of the electron.
In the absence of an electric field, conduction electrons move in different directions, performing chaotic thermal motion. In semiconductors, the movement of electrons is similar to the thermal movement of molecules of an ideal gas. Average speed υ
0 of such motion is found from the condition \(~\frac{m^* \upsilon^2_0}{2} = kT_e\), where
k
is Boltzmann’s constant,
T
e is the electron temperature.
At T
e ≈ 300 K in gallium arsenide (
GaAs
)
υ
0 ≈ 4.5·105 m/s.
In metals, where the electron concentration is much higher than in semiconductors, the conclusions of the molecular kinetic theory of gases cannot be used. As follows from quantum theory, the average speed of chaotic motion of electrons in metals is υ
0 ≈ 106 m/s and practically does not depend on temperature.
Now let's see what the inclusion of the electric field \(~\vec E\) will lead to. The force \(~-e \vec E\) acting on the electron gives it acceleration \(~\vec a = -\frac{e \vec E}{m^*}\). Let's denote the speed i
th electron immediately after scattering \(~\vec \upsilon_i\).
At an arbitrary moment of time, the speed of the ith
electron will be equal to \(~\vec \upsilon_i - \frac{e \vec E}{m^*} t_i\), where
t
i is the time elapsed since the last collision.
Average speed of N
electrons -
\(~\vec u = \frac 1N \sum^N_{i=1} \left( \vec \upsilon_i - \frac{e \vec E}{m^*} t_i \right) = \frac 1N \sum^N_{i=1} \vec \upsilon_i — \frac{e \vec E}{m^*} \left( \frac 1N \sum^N_{i=1} t_i \right) . \)
The quantity \(~\frac 1N \sum^N_{i=1} \vec \upsilon_i\) is the average speed of electrons immediately after scattering. Since the electron velocities immediately after scattering can be directed in any direction, \(~\frac 1N \sum^N_{i=1} \vec \upsilon_i = 0\). The quantity \(~ \frac 1N \sum^N_{i=1} t_i = \tau\) has the meaning of the mean free travel time that is already familiar to us. So, under the influence of an electric field, all electrons acquire an additional speed (it is called drift), the average value of which is equal to \(~u = \frac{eE \tau}{m^*}\), and this speed is directed parallel to the field \(~ \vec E\).
Thus, in the presence of an electric field, a drift motion is superimposed on the chaotic motion of electrons, and a preferential direction of electron motion appears—an electric current arises. If the electron concentration in a conductor is n
, then the density of this current is
\(~j = enu = \frac{ne^2 \tau}{m^*} E.\)
On the other hand, we know that \(~j = \sigma E\). Means,
\(~\sigma = \frac{ne^2 \tau}{m^*}.\)
This formula is called Drude's formula. Ohm's law is valid if none of the quantities included in the Drude formula depends on E
.
If the electron concentration n
or the free path time
τ
, or the effective mass
m
* changes under the influence of an electric field, then Ohm’s law is violated.
Conductors and dielectrics
In other types of materials, such as glass, the electrons of the atoms have very little freedom to move. Although external forces such as physical friction can cause some of these electrons to leave their atoms and go to atoms in another material, they do not move as easily between atoms within the material itself.
This relative mobility of electrons in a material is known as electrical conductivity (electrical conductivity). Electrical conductivity is determined by the types of atoms in a material (the number of protons in each atom's nucleus determines its chemical identity) and how the atoms are bonded to each other. Materials with high electron mobility (many free electrons) are called conductors, and materials with low electron mobility (few or no free electrons) are called insulators. Below are some common examples of conductors and dielectrics:
| Conductors | Dielectrics |
|
|
It should be understood that not all conductive materials have the same level of conductivity, and not all dielectrics are equally resistant to electron movement. Electrical conductivity is analogous to the transparency of some materials to light: materials that easily "conduct" light are called "transparent", and those that do not are called "opaque". However, not all transparent materials transmit light equally. Window glass is better than most plastics, and certainly better than "clear" fiberglass. It's the same with electrical conductors, some are better than others.
For example, silver is the best conductor on the list of "conductors", offering an easier passage for electrons than any other material mentioned. Dirty water and concrete are also considered conductors, but these materials are significantly less conductive than any metal.
It should also be understood that some materials change their electrical properties depending on conditions. Glass, for example, is a very good dielectric at room temperature, but becomes a conductor when heated to very high temperatures. Gases such as air, which are typically insulating materials, also become conductive when heated to very high temperatures. Most metals become poor conductors when heated and become better conductors when cooled. Many conductive materials become perfectly conductive (called superconductivity) at extremely low temperatures.
When is Ohm's law true?
First of all, let us consider under what conditions the value τ
does not change under the influence of
field E.
Time τ
depends on electron speeds.
\
), which appears when the electric field is turned on, increases with increasing E. While the electric field is small, so that the drift speed is much less than the average speed of chaotic motion υ
0, the value
u
can be neglected and time
τ
can
independent of the field
E. If E
is so large that the value
of u
is comparable to
υ
0, then the drift velocity must be taken into account.
In this case, the electron velocities and, consequently, the free travel time τ
turn out to depend on the electric field.
Thus, for Ohm's law to be fulfilled, it is necessary that the condition be satisfied
\(~u \ll \upsilon_0, \qquad (1)\)
that is, the electric field strength in the conductor should be much less than \(~E = \frac{m^* \upsilon_0}{e \tau}\).
In semiconductors, as we have already said, υ
0 ~ 106 m/s.
To achieve a value of u
comparable to
υ
0, a field
E
~ 106 V/m must be applied to the semiconductor. This is a huge value, comparable to the field strength in lightning. Nevertheless, such a field can be created in semiconductors.
There is another, stronger limitation on the speed u
.
It must be less than the speed of sound in the conductor (and υ
zv ~ 103 m/s):
\(~u < \upsilon_{zv}. \qquad (2)\)
As soon as the speed u
reaches the value
υ
zv, sound vibrations are excited in the crystal.
In this case, the free path time τ
and conductivity
σ
, proportional to
τ
, may decrease. This situation is similar to a sharp increase in aerodynamic drag after an airplane breaks the sound barrier.
So, in the field \(~E \ge \frac{m^* \upsilon_{zv}}{e \tau}\) conductivity begins to depend on the value of E
, and Ohm's law is violated.
The effect of the electric field is not limited to the appearance of drift motion. It is known that when current flows in a conductor, Joule heat is released and it heats up. Let's take a closer look at this process.
Any conductor can be considered to consist of two subsystems: a crystal lattice formed by atoms of the substance, and a gas of conduction electrons filling the lattice. Electrons and the lattice can be characterized by their temperatures T
e and
T
p.
In the absence of an electric field, the electron gas is in thermal equilibrium with the lattice and the environment: T
e =
T
p =
T
c.
E
field acts on conduction electrons and primarily heats them up.
Only then is heat transferred from the electrons to the lattice, and then to the environment. Therefore, in the presence of a field, thermal equilibrium is disrupted so that T
e >
T
p >
T
c.
If the heat transfer from the conductor to the environment is worse than the heat transfer from electrons to atoms and, therefore, \(~T_e - T_p \ll T_p - T_c\), then the lattice together with the electrons heats up as a whole. (This situation is typical for the filament of an incandescent lamp.) The opposite case is also possible, when the electron temperature is much higher than the lattice temperature and \(~T_e - T_p \gg T_p - T_c\).
In metals, as we have already said, the average speed of chaotic thermal motion of electrons is practically independent of temperature. But in semiconductors, an increase in T
e under the influence of an electric field means an increase in the speed
υ
0 of the thermal motion of electrons, and therefore a decrease in the free travel time.
If the change Δ υ
0 of the speed
υ
0 is small, i.e. \(~\Delta \upsilon_0 \ll \upsilon_0\), then the dependence of
υ
0 on
E
and, therefore, m on
E
can be neglected.
The condition \(~\Delta \upsilon_0 \ll \upsilon_0\) is equivalent to the condition of small overheating Δ T
e of electrons relative to the equilibrium state:
\(~\Delta T_e \ll T_e . \qquad (3)\)
Thus, the condition for the independence of free time The range of the electric field required to satisfy Ohm’s law sets the following restrictions on the scope of applicability of this law:
\(~u \ll \upsilon_0, \qquad (1)\) \(~u < \upsilon_{zv}, \qquad (2)\) \(~\Delta T_e \ll T_e = T_c . \qquad (3 )\)
Violation of any of these inequalities can lead to deviation from Ohm's law. Below we will see that if the inequalities \(~u \ll \upsilon_0\) and \(~\Delta T_e \ll T_e\) are violated, the electric field E
can also influence other quantities included in the Drude formula—the effective mass
m
* and the electron concentration
n
.
The dependences of m
* and
n
on
E
can significantly change the type of current-voltage characteristics of semiconductors.
Which is faster: lightning or thunder?
This children's question has a simple answer - lightning. From the same school physics course it is known that the speed of sound in air is approximately 331 m/sec. Almost a million times slower than an electromagnetic wave. Knowing this, it is easy to understand how to calculate the distance to lightning.
The flash light reaches us at the moment of discharge, but the sound travels longer. It is enough to detect the time interval between the flash and thunder. Now we simply calculate how far from us the lightning struck, using a simple formula:
L =T × 331
Where T is the time from the flash to the thunder, and L is the distance from us to the lightning in meters.
For example, thunder struck 7.2 seconds after the flash. 331 × 7.2 = 2383. It turns out that lightning struck at an altitude of 2 kilometers 383 meters.
Semiconductors in a strong electric field
In a sample through which current I
, the power
\(~P = I^2R = \sigma E^2LS\)
(we took into account that \(~I = jS = \sigma ES\), \(~R = \frac{\rho L}{ S} = \frac{L}{\sigma S}\)). The power released per unit volume is \(~Q = \sigma E^2\). For the same value of Q
the electric field \(~E = \sqrt{\frac{Q}{\sigma}}\) in semiconductors is much greater than in metals, since the concentration of electrons in semiconductors and, therefore, the conductivity
σ
is much lower. Consequently, it is easier to violate the conditions \(~u < \upsilon_{zv}\), \(~u \ll \upsilon_0\) in them. In addition, for each electron in a semiconductor there is more power than in a metal. The electron gas heats up more strongly, so the inequality \(~\Delta T_e \ll T_e\) is also violated more easily.
Violation of which of the conditions - (1), (2) or (3) - with an increase in the electric field will lead to the most significant deviation from Ohm's law depends on the type of semiconductor. For example, in CdS
first, the condition \(~u < \upsilon_{zv}\) is violated.
In this case, in a field E
zv = 1.4·105 V/m, a kink appears in the current-voltage characteristic
j
(
E
) (Fig. 2).
If E
>
E
zv, this semiconductor emits sound intensively and can be used as a generator of sound vibrations.
Rice. 2
In other semiconductors such as Ge
,
Si
,
GaAs
,
InP
,
CdTe
, sound is excited much weaker, and no noticeable break is observed in the field
E
zv.
In these semiconductors, deviations from Ohm's law are associated with violation of the condition \(~\Delta T_e \ll T_e\). In this case, the free travel time turns out to be inversely proportional to the field E
, i.e. \(~\tau (E) \sim \frac 1E\), and the dependence of the current density on the field is associated only with changes in
m
* and
n
.
In Ge
and
Si
at
E
> 106 V/m, the current-voltage characteristic (Fig. 3) exhibits saturation
j
(
m
*,
n
do not depend on
E
).
In GaAs
,
InP
,
CdTe
, as the electron energy increases with increasing
E
, not only does the free path time
τ
m
also increases .
The increase in m
* is caused by a change in the interaction of electrons with the crystal lattice.
As a result, in these semiconductors, starting from a certain value of the electric field E
a, the current density
j
decreases with increasing
E
(section
E
a <
E
<
E
b in Figure 3).
In GaAs,
the drop in
j
starts from
E
a = 3.2 105 V/m and continues until
E
b ≈ 10
E
a.
In the field E
a, the drift velocity of electrons is \(~u = \frac{j}{en}\) = 1.5·105 m/s.
Rice. 3
In an even stronger field E
~ 107 V/m, along with the violation of the condition \(~\Delta T_e \ll T_e\), the condition \(~u \ll \upsilon_0\) is also violated.
In such a field, electrons receive during their free path energy sufficient to ionize atoms. Fast electrons, when colliding with atoms, knock out additional electrons, which in turn are also accelerated by the field and generate new charge carriers. This process is called impact ionization. The total concentration n
of electrons increases and, consequently, the conductivity increases.
With an even greater increase in the electric field ( E
> 107 V/m), the concentration and conductivity increase like an avalanche, and breakdown of the semiconductor occurs.
Thus, in semiconductors in very strong fields E
The current density \(~j = \sigma E\) increases faster than according to the linear law.
In particular, in Ge
and
Si
the current saturation is replaced by its nonlinear growth, and in
GaAs
,
InP
,
CdTe
the current-voltage characteristic takes on
an N
-shaped form (Fig. 3);
at 0 < E
<
E
a Ohm's law is satisfied, in the interval
E
a <
E
<
E
b there is a falling section caused by a decrease
in τ
and an increase in
m
* in a strong electric field, and, finally, in the region
E
>
E
b there is a rapid increase
in j
due to the increase
in n
.
Gunn effect
The presence of a falling section on the current-voltage characteristic leads to an interesting phenomenon discovered by the American engineer John Gunn.
Let us apply to a GaAs
of length
L,
the voltage
U
0 is such as to be on the falling portion of
the j
(
E
) dependence.
Let us assume that at first the electric field in the sample is uniform and equal to \(~\frac{U_0}{L}\). Suppose that for some reason in the thin layer AB
of the sample the field
E
turned out to be slightly larger than in the rest of the sample volume (Fig. 4).
Then the electron drift speed \(~u = \frac{j}{en}\) inside the AB
will be less than outside.
Therefore, more electrons will approach boundary A
than fly away from it, and
vice versa
B. Near A
there will be an excess of negative charge, and near
B
- a positive one.
Consequently, an additional electric field will appear in the AB
, directed in the same direction as the original one. An increase in the field will lead to the fact that the drift velocity of electrons inside the layer will further decrease, and the field there will increase even more.
Rice. 4
Thus, the uniform distribution of the electric field in the incident section j
(
E
) is impossible: any heterogeneity
E
, no matter how weak, that accidentally arises in the sample does not resolve, but grows.
As a result, a narrow region (size δ
) of a strong field is formed, which is called an electric domain.
In this case, since the voltage U
0 on the sample is given, i.e.
\(~E_2 \delta + E_1 (L - \delta) = U_0 = \operatorname{const},\)
the increase in the field E
2 in the domain is accompanied by a decrease in the field
E
1 outside it.
There will come a moment when E
1 <
E
a and
E
2 >
E
b (see Fig. 3).
The speed of electron drift outside the domain will begin to decrease, and inside it will increase. The growth of the field E
2 in the domain will stop when these rates become equal, and the current densities in the domain and in the sample become the same:
\(~j(E_1) = j(E_2) = j_0.\)
From the last two equalities it follows that the steady-state sample current density j
0 depends on the domain thickness
δ
.
Typically, a domain appears near the cathode (due to the melting of contacts there are more inhomogeneities here) and, carried away by the flow of electrons, begins to move towards the anode at a speed \(~u_0 = \frac{j_0}{en}\). While it moves along the sample, its size does not change, which means that the current j
0. Near the anode, the domain begins to disappear, its thickness decreases, and the current in the sample increases.
E
field outside the domain increases .
As soon as E
1 reaches the value of
E
a, a new domain is generated at the cathode, the current begins to decrease, and this process is repeated periodically (Fig. 5). The period of current oscillations in the sample is \(~T_0 = \frac{L}{u_0}\).
Rice. 5
So, applying a constant voltage U
0, we get an alternating current of frequency \(~f = \frac{1}{T_0} = \frac{u_0}{L}\).
This is completely different from Ohm's law. In gallium arsenide ( GaAs
)
u
0 ≈ 105 m/s.
Using small samples from one to one hundred microns in length, it is possible to change the frequency of alternating current in the range f
~ 109 - 1011 Hz. Most modern ultrahigh frequency (microwave) generators operate based on the Gunn effect. These devices are used, for example, to determine the speed of vehicles by traffic police posts and in television broadcasting via artificial Earth satellites.
AC and DC current - what's the difference?
The difference is that electric charges do not move in the same way. A constant can only move in one direction. In solids, electrons move; in others, ions move. Therefore, in solids, current always flows from minus to plus. In liquid and gaseous substances, it can move in 2 directions: electrons - to the plus, and ions - to the minus and the source of energy.
With the variable movement of particles the situation is different: instead of its movement only in one direction, the latter can periodically change to the opposite. For example, in city electrical networks the standard voltage is 220 V and the frequency is 50 Hz. Frequency means that in 1 second the current passes through a sinusoidal cycle 50 times.
This means that it changes direction 100 times per second, since the cycle changes twice.