| 0.22 Ohm |
| 0.47 Ohm |
| 1 ohm |
| 1.5 ohm |
| 2 ohm |
| 3 ohm |
| 3.3 ohm |
| 4.7 ohm |
| 5.6 ohm |
| 10 ohm |
| 11 ohm |
| 12 ohm |
| 13 ohm |
| 15 ohm |
| 16 ohm |
| 18 ohm |
| 20 ohm |
| 22 Ohm |
| 24 ohm |
| 27 Ohm |
| 30 ohm |
| 33 Ohm |
| 36 Ohm |
| 39 Ohm |
| 43 Ohm |
| 47 Ohm |
| 49.9 ohms |
| 51 Ohm |
| 56 Ohm |
| 62 Ohm |
| 68 Ohm |
| 75 Ohm |
| 82 Ohm |
| 91 Ohm |
| 100 Ohm |
| 110 Ohm |
| 120 Ohm |
| 130 Ohm |
| 150 Ohm |
| 160 Ohm |
| 200 Ohm |
| 220 Ohm |
| 240 Ohm |
| 270 Ohm |
| 300 Ohm |
| 330 Ohm |
| 360 Ohm |
| 390 Ohm |
| 430 Ohm |
| 470 Ohm |
| 499 Ohm |
| 510 Ohm |
| 560 Ohm |
| 620 Ohm |
| 680 Ohm |
| 750 Ohm |
| 820 Ohm |
| 910 Ohm |
| 1 kOhm |
| 1.1 kOhm |
| 1.2 kOhm |
| 1.3 kOhm |
| 1.5 kOhm |
| 1.6 kOhm |
| 1.8 kOhm |
| 2.0 kOhm |
| 2.2 kOhm |
| 2.4 kOhm |
| 2.7 kOhm |
| 3.0 kOhm |
| 3.01 kOhm |
| 3.3 kOhm |
| 3.4 kOhm |
| 3.6 kOhm |
| 3.9 kOhm |
| 3.92 kOhm |
| 4.3 kOhm |
| 4.7 kOhm |
| 4.99 kOhm |
| 5.1 kOhm |
| 5.6 kOhm |
| 6.2 kOhm |
| 6.8 kOhm |
| 7.15 kOhm |
| 7.5 kOhm |
| 8.2 kOhm |
| 9.09 kOhm |
| 9.1 kOhm |
| 9.76 kOhm |
| 10 kOhm |
| 11 kOhm |
| 12 kOhm |
| 13 kOhm |
| 15 kOhm |
| 16 kOhm |
| 18 kOhm |
| 20 kOhm |
| 22 kOhm |
| 24 kOhm |
| 27 kOhm |
| 28 kOhm |
| 30 kOhm |
| 33 kOhm |
| 36 kOhm |
| 39 kOhm |
| 40.2 kOhm |
| 43 kOhm |
| 44.2 kOhm |
| 47 kOhm |
| 49.9 kOhm |
| 51 kOhm |
| 51.1 kOhm |
| 56 kOhm |
| 56.2 kOhm |
| 62 kOhm |
| 68 kOhm |
| 75 kOhm |
| 82 kOhm |
| 90.9 kOhm |
| 100 kOhm |
| 110 kOhm |
| 120 kOhm |
| 130 kOhm |
| 150 kOhm |
| 160 kOhm |
| 174 kOhm |
| 180 kOhm |
| 200 kOhm |
| 220 kOhm |
| 237 kOhm |
| 240 kOhm |
| 270 kOhm |
| 300 kOhm |
| 316 kOhm |
| 330 kOhm |
| 348 kOhm |
| 360 kOhm |
| 390 kOhm |
| 430 kOhm |
| 470 kOhm |
| 499 kOhm |
| 510 kOhm |
| 560 kOhm |
| 604 kOhm |
| 620 kOhm |
| 680 kOhm |
| 750 kOhm |
| 820 kOhm |
| 910 kOhm |
| 1 MOhm |
| 1.1 MOhm |
| 1.2 MOhm |
| 1.5 MOhm |
| 2 MOhm |
| 2.2 MOhm |
| 3 MOhm |
| 4.99 MOhm |
| 5.1 MOhm |
| 10 MOm |
What is the marking of smd resistors
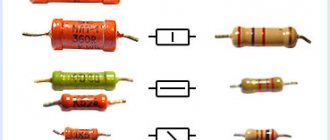
SMD resistors are permanent parts that are required for surface mounting on a board. If we compare smd resistors and metal film resistors, the former will be several times smaller, but there are also those that are larger, which is why there is a marking of smd resistors. They also differ in shape; there are square, rectangular and round and even oval. Carefully studying the SMD resistor markings, you can note that the markings can be digital or alphabetic.
The main difference between SMD resistors is the presence of small contacts that are inserted into the printed circuit board. Let's look at why resistor markings are needed.
Why do we need resistor markings?
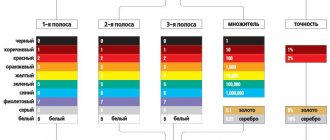
Considering the fact that SMD resistors are small in size, they cannot be color coded, so manufacturers have developed a different marking method. As a rule, the designation of smd resistors contains three or four numbers; letters may be present.
- Digital marking of resistors is necessary in order to indicate the numerical value of the resistance of the resistor, the last digit is a multiplier. It can also indicate the power that must be raised to 10 to get the final result. For example, resistance can be determined this way: 450 = 45 x 10 equals 45 Ohms.
- If the marking is EIA-96, then this means that the resistors are high precision. This standard is intended for resistors that have a small resistance of 1%. This marking system has three elements: 2 numbers that indicate the denomination code, and the letters are a multiplier. The numbers are a code that gives the resistance number. For example, code 04 may indicate 107 ohms.
For convenient calculation, a calculator is used that will help you quickly find the resistance value. To calculate, you need to enter the code that is on the component and the resistance will immediately appear below. This calculator is suitable not only for the standard. To more accurately check the resistance, it is best to use a multimeter for calculations. Read here which is the best multimeter to choose.
What characteristics does it show?
The most important characteristics of the parts are the value of the nominal resistance, the tolerance on the value and the temperature coefficient. Any of these characteristics is related to the power of SMD resistors and the resistance between it and the ambient temperature. In some areas, even noise characteristics are taken into account.
Important! Component characteristics include stability, voltage, resistance dependence and frequency parameters.
To understand this issue in detail, you need to carefully study all the characteristics:
- The value of the nominal resistance. The tolerance on the nominal resistance value is specified as a percentage. This value indicates the resistance of the resistor under external influences on it.
- Temperature. As a rule, the natural temperature is +20°C and there should be normal atmospheric pressure. SMD resistors are produced with a tolerance for nominal resistance ranging from ±0.05% to ±5%.
- Accuracy. The most accurate resistors can be considered those that are calculated using the formula TKS=DR/(R*DT). DR means the change in resistance when the temperature changes by the amount DT, R is the nominal value of the resistance.
If the components can be calculated using this formula, then this means that they have the highest accuracy.
Standard sizes
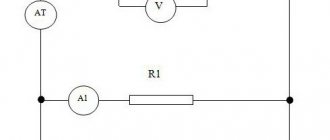
In communication equipment circuits, it is often necessary to supply the consumer with less voltage than the source provides. In this case, an additional resistance is connected in series with the main consumer, at which the excess voltage of the source is extinguished. The video shows a simple calculation of a resistor for an LED.
This resistance is called damping resistance. The voltage of the current source is distributed over sections of the series circuit in direct proportion to the resistance of these sections. Let's consider the circuit for switching on the damping resistance:
- The payload in this circuit is an incandescent light bulb, designed for normal operation at voltage Ul = 80 V and current I = 20 mA.
- The voltage at the terminals of the current source U=120 V is greater than Ul, so if you connect a light bulb directly to the source, then a current exceeding normal will pass through it and it will burn out.
- To prevent this from happening, a damping resistance R extinguished is connected in series with the light bulb.
Calculation of the value of the damping resistance at given values of the consumer current and voltage comes down to the following:
– the amount of voltage that must be extinguished is determined:
Next, you need to calculate the power released at the damping resistance using the formula
Knowing the resistance value and power consumption, choose the type of damping resistance
Expert opinion
Viktor Pavlovich Strebizh, lighting and electrical expert
Any questions ask me, I will help!
Also, such resistors are distinguished by their low fit on the printed circuit board, which allows them to be placed on the circuit more compactly and significantly save useful space. If there is something you don’t understand, write to me!
Types of SMD resistor markings
An important characteristic of resistors is the size. In simple words, this is the size, length and width of the body. It is by taking these elements into account that it is possible to select the appropriate board layout.
Reference! All sizes of SMD resistors in the documentation are indicated using special numbers and letters. The first numbers can indicate the dimensions, which are given in millimeters, the second pair of characters can indicate the width, also in millimeters.
Let's look at some typical resistor sizes and their decoding by numbers:
- SMD resistors 0201: length = 0.6 mm, width = 0.3 mm, height = 0.23 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.05 W, voltage maximum 50 V.
- SMD resistors 0402: length = 1.0 mm, width = 0.5 mm, height = 0.35 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.05 W, voltage maximum 100 V.
- SMD resistors 0603: length = 1.6 mm, width = 0.8 mm, height = 0.45 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.01 W, voltage maximum 100 V.
- SMD resistors 0805: length = 2.0 mm, width = 1.2 mm, height = 0.4 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.125 W, voltage maximum 200 V.
- SMD resistors 1206: length = 3.2 mm, width = 1.6 mm, height = 0.5 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.25 W, voltage maximum 400 V.
- SMD resistors 2010: length = 5.0 mm, width = 2.5 mm, height = 0.55 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 0.75 W, voltage maximum 200 V.
- SMD resistors 2512: length = 6.35 mm, width = 3.2 mm, height = 0.55 mm. The nominal values are 0 Ohm, 1 Ohm - 30 MOhm. Power is only 1 W, voltage maximum 400V.
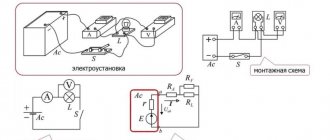
Power in series connection
When resistors are connected in series, electric current passes through each resistance in turn. The current value at any point in the circuit will be the same. This fact is determined using Ohm's law. If you add up all the resistances shown in the diagram, you get the following result: R = 200+100+51+39 = 390 Ohms.
Considering the voltage in the circuit is 100 V, according to Ohm’s law, the current will be I = U/R = 100/390 = 0.256 A. Based on the data obtained, the power of the resistors in a series connection can be calculated using the following formula: P = I2 x R = 0.2562 x 390 = 25.55 W.
In the same way, you can calculate the power of each individual resistor:
If we add up the received power, then the total P will be: P = 13.11 + 6.55 + 3.34 + 2.55 = 25.55 W.
Examples of decoding digital markings of SMD resistors
To quickly understand the decoding of SMD resistor markings, you need to consider several options.
Resistor 103
To calculate resistance, it is worth understanding the numbers from the very beginning. If we take resistor 103, then the first two digits will indicate the numbers, and the third digit will be the number of zeros, so it turns out that 10 and 3 will be 10,000 ohms. Such components may have a small error, which ranges from 2 to 10%.
Resistor 1206
In this case, the resistance is calculated in a different way. The first three digits are the number, and the last number of zeros or, as they say, the power to which the factor 10 must be raised. So, let’s calculate the value of the element with characteristic 1206:
120 * 10 to the 6th power = 2.985984 × 10^12 kOhm with an error of 1%
Resistor 2r2
If the component has a fractional value, then the letter R is placed in the code instead of a dot. In this case, the calculation for the resistor is 2R2 = 2.2 Ohm.
The most difficult thing to calculate is alphabetic and numeric codes, since numbers contain one information, and letters act as a multiplier. For quick calculations, there are special online calculators that help determine the resistance of an SMD resistor. There is also a marking table that is useful in calculations.
conclusions
The only thing that may confuse you is the rather high price; in other places you can get much more LEDs for the same money.
Another criticism, of course, would be that the seller did not indicate the characteristics, although on the other hand, the one who buys it usually knows how to determine them. pros
— Working — Bright
Minuses
— Installation of SMD requires care — High price
The product was provided for writing a review by the store. The review was published in accordance with clause 18 of the Site Rules.
SMD resistor marking table (code/nominal/size/power) table
SMD resistors marking table:
| Code | Nominal, W | Size | Power V |
| 0402 | 0.062 | Length 1.0 ±0.1, width 0.5 ±0.05, height 0.35 ±0.05 | 100 |
| 0603 | 0.1 | Length 1.6 ±0.1 width 0.85 ±0.1 height 0.45 ±0.05 | 100 |
| 0805 | 0.125 | Length 2.1±0.1 width 1.3 ±0.1 height0.5 ±0.05 | 200 |
| 1206 | 0.25 | Length 3.1 ±0.1 width1.6 ±0.1 height0.55 ±0.05 | 400 |
| 1210 | 0.33 | Length 3.1 ±0.1 width 2.6 ±0.1 height0.55 ±0.05 | 400 |
| 2010 | 0.75 | Length 5.0 ±0.1 width 2.5 ±0.1 height 0.55 ±0.05 | 400 |
| 2512 | 1 | Length 6.35 ±0.1 width 3.2 ±0.1 height 0.55 ±0.05 | 400 |
| 0075 | 0,02 | Length 0.3 Width 0.15 | 100 |
| 01005 | 0,03 | Length 0.4 Width 0.2 | 100 |
| 0201 | 0,05 | Length 0.6 Width 0.3 | 100 |
| 1218 | 1 ; 1,5 | Length 3.2 Width 4.8 | 150 |
| 1812 | 0,5; 0,75 | Length 4.5 Width 3.2 | 200 |
Today there are a huge number of highly specialized parts that differ in their advantages and disadvantages. For example, there are capacitors that can operate at high temperatures, almost 230 °C, there are those that are designed to work in aggressive environments, and milliohm chip resistors have also appeared. There are capacitors that can only be used in certain circuits. The table above indicates standard options, but actual power dissipation may vary.