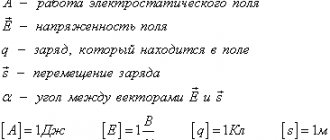We are familiar with the concept of “work” in the everyday sense. When we work, we perform some action, most often useful. In physics (more precisely, in mechanics), the term “work” shows what force was applied as a result of an action, and how far the body moved as a result of this force.
For example, we need to lift a bicycle up the stairs into an apartment. Then the work will be determined by how much the bike weighs and on what floor (at what height) the apartment is located.
Mechanical work is a physical quantity directly proportional to the force applied to the body and the path traveled by the body.
To calculate the work, we need to multiply the numerical value of the force F by the path traveled by the body in the direction of the force S. is designated by the Latin letter A.
| Mechanical work A = FS A - mechanical work [J] F - applied force [N] S – path [m] |
If under the influence of a force of 1 newton a body moves 1 meter, then this force does 1 joule of work.
Since force and path are vector quantities, if there is an angle between them, the formula takes the form.
| Mechanical work A = FScosα A - mechanical work [J] F - applied force [N] S – path [m] α - angle between force and displacement vectors [°] |
The numerical value of work can become negative if the force vector is opposite to the velocity vector. In other words, force can not only give the body speed to make a movement, but also impede the movement already being made. In this case, the force is called counteracting.
To complete the work, two conditions are necessary:
- so that a force acts on the body,
- for the body to move.
The force acting on the body may not do work. For example, if someone unsuccessfully tries to move a heavy cabinet. The force with which a person acts on the cabinet does not do any work, since the movement of the cabinet is zero.
Remember!
Work is zero if:
- when force is applied there is no movement;
- no force is applied and the body moves by inertia;
- the angle between the force and displacement vectors is 90°.
Useful and expended work
The ancient Greeks had such a mythological character - Sisyphus. Because he deceived the gods, they sentenced him after death to forever carry a huge boulder up the mountain from where this boulder rolled down - and so on endlessly. In general, Sisyphus was doing a completely useless job with zero efficiency. That’s why useless work is called “Sisyphean labor.”
To understand the concepts of useful and expended work, let's fantasize and imagine that Sisyphus was pardoned and the stone no longer rolls down the mountain, and the efficiency is no longer zero.
The useful work in this case is equal to the potential energy acquired by the cobblestone. Potential energy, in turn, is directly proportional to height: the higher the body is located, the greater its potential energy. It turns out that the higher Sisyphus rolled the stone, the greater the useful work.
| Potential energy Ep = mgh m —body weight [kg] g —gravitational acceleration [m/s2] h - height [m] On planet Earth g ≈ 9.8 m/s2 |
The work expended in our example is the mechanical work of Sisyphus. Mechanical work depends on the force applied and the path along which that force was applied.
| Mechanical work A = FS A - mechanical work [J] F - applied force [N] S – path [m] |
And how can you reliably determine which work is useful and which is wasted?
Everything is very simple! We ask two questions:
- What makes the process happen?
- For what result?
In the example above, the process occurs in order for the body to rise to a certain height, which means it acquires potential energy (for physics these are synonyms).
The process occurs due to the energy expended by Sisyphus - this is the work expended.
Power
In factories around the world, most tasks are performed by machines. For example, if we need to cap a thousand cola cans, the machine will do it in a matter of minutes. For a human, this task would take much longer. It turns out that the machine and the person perform the same work over different periods of time. In order to describe the speed at which work is performed, we need the concept of power.
Power is a physical quantity equal to the ratio of work to the time it is performed.
| Power N = A/t N - power [W] A - mechanical work [J] t — time [s] |
One watt is the power at which one joule of work is done in one second.
Another formula is also valid for power:
| Power N = Fv N - power [W] F - applied force [N] v —speed [m/s] |
As with work, the rule of signs is valid for power: if the vectors are directed in opposite directions, the power value will be negative.
Since force and speed are vector quantities, if there is an angle between them, the formula takes the following form:
| Power N = Fvcosα N - power [W] F - applied force [N] v —speed [m/s] α is the angle between the force and velocity vectors [°] |
Power unit
A unit of power is taken to be the power at which 1 J of work is done in 1 s.
This unit is called the watt (W) in honor of the scientist Watt (Figure 1).
Figure 1. James Watt (1736-1819) - Scottish engineer and inventor.
From the power formula $N = \frac{A}{t}$:
$$1 \space watt = \frac{1 \space joule}{1 \space second} = 1 \frac{J}{s}$$
Other power units often used are kilowatt (kW), megawatt (MW) and milliwatt (mW):
$1 \space MW = 1,000,000 \space W$
$1 \space W = 0.000001 MW$
$1 \space kW = 1000 \space W$
$1 \space W = 0.001 kW$
$1 \space mW = 0.001 \space W$
$1 \space W = 1000 \space mW$
Power is also sometimes measured in horsepower (hp):
$1 \space l. With. = 735.5 \space W$
$1 \space W = 0.00013596 \space hp$
This unit of measurement is not as popular as the watt, but is still used, for example, in the automotive industry.
Examples of problem solving
Problem 1
The spoon is slowly sinking into a large jar of honey. It is acted upon by gravity, viscous friction and buoyancy. Which of these forces does positive work when the body moves? Choose the correct answer:
- Buoyancy force.
- Viscous friction force.
- Gravity.
- None of the above forces.
Solution
As the spoon falls down, the movement is downward. Only the force of gravity is directed in the same direction as the movement. This means that it does positive work.
Answer: 3.
Problem 2
The box is pulled along the ground by a rope along a horizontal circle of length L = 40 m with a speed constant in absolute value. The modulus of the friction force acting on the box from the ground is 80 N. What is the work done by the traction force per revolution?
Solution
Since the box is pulled at a constant absolute speed, its kinetic energy does not change. All the energy that is spent on the work of the friction force must enter the system due to the work of the traction force. From here we find the work done by the traction force per revolution:
Answer: 3200 J.
Problem 3
A body with a mass of 2 kg under the influence of a force F moves up an inclined plane at a distance l = 5 m. The distance of the body from the surface of the Earth increases by 3 meters. The force vector F is directed parallel to the inclined plane, the modulus of the force F F during this movement in the reference frame associated with the inclined plane ?
Solution
In this case, we are asked to find the work done by a force F when moving a body along an inclined plane. This means that we are interested in the force F and the distance traveled. If we were asked about the work of gravity, we would count through gravity and height.
The work of a force is defined as the scalar product of the force vector and the displacement vector of the body. Hence:
A = Fl = 30 * 5 = 150 J
Answer: 150 J.
Exercise 31
1. Express the power in kilowatts and megawatts: 2500 W; 100 W. Express the power in watts: 5 kW; 2.3 kW; 0.3 kW; 0.05 MW; 0.001 MW.
2. From a dam 22 m high, 500 tons of water falls in 10 minutes. What power is developed in this case?
3. What is the power of a person when walking if he takes 10,000 steps in 2 hours and does 40 J of work for each step?
4. What work does a 100 kW engine do in 20 minutes?
5. In 1 hour, a conveyor lifts 30 m3 of sand to a height of 6 m. Calculate the engine power required for this work. Sand density is 1500 kg/m3.
6. A weightlifter lifted a barbell weighing 125 kg to a height of 70 cm in 0.3 s. What average power did the athlete develop in this case?
Efficiency
When performing necessary (useful) work, for example, mechanical work, it is necessary to perform a larger amount of work, since in reality there are resistance forces and part of the energy is subject to dissipation (dissipation). The efficiency of work is determined using the efficiency factor ($\eta $), while:
\[\eta =\frac{P_p}{P}\left(5\right),\]
where $P_p$ is useful power; $P$ is the consumed power. From expression (5) it follows that the useful power can be found as:
\[P_p=\eta P\ \left(6\right).\]
What does the efficiency depend on?
This value depends on how much total perfect work can turn into useful work. First of all, it depends on the design of the mechanism or machine itself. Engineers all over the world are struggling to increase the efficiency of machines. For example, for electric vehicles the coefficient is very high - more than 90%.
But an internal combustion engine, due to its design, cannot have η close to 100 percent. After all, fuel energy does not act directly on the rotating wheels. Energy is dissipated at each transmission link. Too many transmission links, and some of the exhaust gases still exit into the exhaust pipe.
Formula for useful power of current source
Let the electrical circuit consist of a current source having resistance $r$ and a load (resistance $R$). We find the power of the source as:
\[P=?I\ \left(7\right),\]
where $?$ is the EMF of the current source; $I$ is the current strength. In this case, $P$ is the total power of the circuit.
Let us denote $U$ as the voltage on the external section of the circuit, then we present formula (7) in the form:
\[P=?I=UI+I^2r=P_p+P_0\left(8\right),\]
where $P_p=UI=I^2R=\frac{U^2}{R}(9)$ is useful power; $P_0=I^2r$ — loss power. In this case, the source efficiency is determined as:
\[\eta =\frac{P_p}{P_p+P_0}\left(9\right).\]
The maximum useful power (power at the load) is produced by the electric current if the external resistance of the circuit is equal to the internal resistance of the current source. Under this condition, the useful power is equal to 50\% of the total power.
During a short circuit (when $R\to 0;;U\to 0$) or in idle mode $(R\to \infty ;;I\to 0$) the useful power is zero.
Examples of efficiency calculations
Example 1. It is necessary to calculate the coefficient for a classic fireplace. Given: the specific heat of combustion of birch firewood is 107 J/kg, the amount of firewood is 8 kg. After the wood burned, the temperature in the room increased by 20 degrees. The specific heat capacity of a cubic meter of air is 1.3 kJ/kg*deg. The total cubic capacity of the room is 75 cubic meters.
To solve the problem, you need to find the quotient or ratio of two quantities. The numerator will be the amount of heat that the air in the room received (1300J*75*20=1950 kJ). The denominator is the amount of heat released by wood during combustion (10000000J*8 =8*107 kJ). After calculations, we find that the energy efficiency of a wood-burning fireplace is about 2.5%. Indeed, modern theory about the design of stoves and fireplaces says that the classic design is not energy efficient. This is due to the fact that the pipe directly discharges hot air into the atmosphere. To increase efficiency, a chimney with channels is installed, where the air first gives off heat to the masonry of the channels, and only then comes out. But in fairness, it should be noted that during the combustion of a fireplace, not only the air, but also the objects in the room are heated, and part of the heat goes out through elements that are poorly insulated - windows, doors, etc.
What kind of lighting do you prefer?
Built-in Chandelier
Example 2. The car traveled 100 km. The weight of the car with passengers and luggage is 1400 kg. In this case, 14 liters of gasoline were consumed. Find: Engine efficiency.
To solve the problem, it is necessary to relate the work of moving the load to the amount of heat released during fuel combustion. The amount of heat is also measured in Joules, so there is no need to convert to other units. A will be equal to the product of force and path (A=F*S=m*g*S). The force is equal to the product of mass and the acceleration of gravity. Useful work = 1400 kg x 9.8 m/s2 x 100000 m = 1.37 * 108 J
The specific heat of combustion of gasoline is 46 MJ/kg=46000 kJ/kg. We will consider eight liters of gasoline to be approximately equal to 8 kg. The heat released was 46*106*14=6.44*108 J. As a result, we obtain η ≈21%.
Power and efficiency
The power of a mechanism or device is equal to the work done per unit of time. Work (A) is measured in Joules, and time in the C system is measured in seconds. But do not confuse the concept of power and rated power. If a kettle says 1,700 watts, it doesn't mean it will transfer 1,700 Joules in one second to the water poured into it. This is the nominal power. To find out the η of an electric kettle, you need to find out the amount of heat (Q) that a certain amount of water should receive when heated by a certain number of degrees. This figure is divided by the work of the electric current performed during the heating of the water.
The value of A will be equal to the rated power multiplied by the time in seconds. Q will be equal to the volume of water times the temperature difference times the specific heat capacity. Then we divide Q by A current and get the efficiency of the electric kettle, approximately equal to 80 percent. Progress does not stand still, and the efficiency of various devices is increasing, including household appliances.
This begs the question why the efficiency of a device cannot be determined through power. The packaging with the equipment always indicates the rated power. It shows how much energy the device consumes from the network. But in each specific case it will be impossible to predict exactly how much energy will be required to heat even one liter of water.
For example, in a cold room, part of the energy will be spent on heating the space. This is due to the fact that as a result of heat exchange the kettle will cool. If, on the contrary, the room is hot, the kettle will boil faster. That is, the efficiency in each of these cases will be different.