4.6
Average rating: 4.6
Total ratings received: 185.
4.6
Average rating: 4.6
Total ratings received: 185.
The resistivity value characterizes the ability of a substance to limit electric current (provide resistance). Metal conductors have the lowest resistivity values, so they are used for transmitting electricity over long distances, and as connecting wires in electronic devices, and connecting tracks on microcircuit boards. Let's figure out why metals have this property and which of them are best suited for these purposes.
Why resistance occurs
Electrons, colliding with charged atoms (ions) that make up the crystal lattice of the conductor, lose speed. The mass of an atom significantly exceeds the mass of an electron, so their collision leads to a loss of speed (“braking”) and a change in the direction of motion of the electron. This creates resistance to the flow (increase) of current. This means that resistance is a physical quantity.
Collisions of electrons with atoms.
What is it measured in?
According to the international system of units, the value is measured in ohms multiplied by a meter. In some cases, the unit used is ohm multiplied by millimeter squared divided by meter. This is a designation for a conductor having a meter length and a square millimeter cross-sectional area.
Unit
Formula how to find
According to the provision from any textbook on electrodynamics, the resistivity of the conductor material formula is equal to the proportion of the total resistance of the conductor per cross-sectional area, divided by the conductor length. It is important to understand that the final indicator will be influenced by temperature and the degree of material purity. For example, if you add a little manganese to copper, the overall indicator will be increased several times.
Main calculation formula
Interestingly, there is a formula for inhomogeneous isotropic material. To do this, you need to know the electric field strength with the electric current density. To find it, you need to divide the first quantity by the other. In this case, the result is not a constant, but a scalar quantity.
Ohm's law in differential form
There is another, more difficult to understand formula for an inhomogeneous anisotropic material. Depends on the tensor coordinate.
It is important to note that the relationship between resistance and conductivity is also expressed by formulas. There are rules for finding isotropic and anisotropic materials through tensor components. They are shown in the diagram below.
Relationship with conductivity expressed in physical relations
What does it depend on
Resistance depends on temperature. It increases when the thermometer rises. This is explained by physicists in such a way that as the temperature increases, atomic vibrations in the crystalline conductor lattice increase. This prevents free electrons from moving around.
Note! As for semiconductors and dielectrics, the value decreases due to the fact that the structure of the concentration of charging carriers increases.
Temperature dependence as the main property of conductive resistance
What do resistivity numbers mean?
In order to be able to compare the resistivity of different materials, from products such as copper and aluminum to other metals and substances including bismuth, brass and even semiconductors, it is necessary to use a standard measurement.
The unit of resistivity in the International System of Units (SI) is Ohm m.
The SI unit of resistivity is equal to the resistivity of a substance such that a homogeneous conductor 1 m long with a cross-sectional area of 1 m2, made from this substance, has a resistance of 1 ohm. Accordingly, the resistivity of an arbitrary substance, expressed in SI units, is numerically equal to the resistance of a section of an electrical circuit made of a given substance with a length of 1 m and a cross-sectional area of 1 m2
The nature of resistance
Conductors are pure metals and their alloys. In a metal, atoms fixed in a single “strong” structure have free electrons (the so-called “electron gas”). It is these particles that in this case are the charge carriers. Electrons are in constant, random motion from one atom to another. When an electric field appears (connecting a voltage source to the ends of the metal), the movement of electrons in the conductor becomes ordered. Moving electrons encounter obstacles on their path caused by the peculiarities of the molecular structure of the conductor. When they collide with a structure, charge carriers lose their energy, giving it to the conductor (heating it). The more obstacles a conductive structure creates to charge carriers, the higher the resistance.
Thus, the basic formula for calculating resistance includes the length of the wire, the cross-sectional area and a certain coefficient that relates these dimensional characteristics to the electrical quantities of voltage and current (1). This coefficient is called resistivity. R= r*L/S (1)
Resistivity table for common conductors
The table below shows resistivity values for various materials, particularly metals used for electrical conductivity.
Resistivity indicators are given for such “popular” materials as copper, aluminum, nichrome, steel, lead, gold and others.
MaterialResistivity, ρ, at 20 °C (Ohm m)Source
| Brass | ~0.6 – 0.9 x 10-7 | |
| Silver | 1.59×10−8 | [3][4] |
| Copper | 1.68×10−8 | [5][6] |
| Burnt copper | 1.72×10−8 | [7] |
| Gold | 2.44×10−8 | [3] |
| Aluminum | 2.65×10−8 | [3] |
| Calcium | 3.36×10−8 | |
| Tungsten | 5.60×10−8 | [3] |
| Zinc | 5.90×10−8 | |
| Cobalt | 6.24×10−8 | |
| Nickel | 6.99×10−8 | |
| Ruthenium | 7.10×10−8 | |
| Lithium | 9.28×10−8 | |
| Iron | 9.70×10−8 | [3] |
| Platinum | 1.06×10−7 | [3] |
| Tin | 1.09×10−7 | |
| Tantalum | 1.3×10−7 | |
| Gallium | 1.40×10−7 | |
| Niobium | 1.40×10−7 | [8] |
| Carbon steel (1010) | 1.43×10−7 | [9] |
| Lead | 2.20×10−7 | [2][3] |
| Galinstan | 2.89×10−7 | [10] |
| Titanium | 4.20×10−7 | |
| Electrical steel | 4.60×10−7 | [11] |
| Manganin (alloy) | 4.82×10−7 | [2] |
| Constantan (alloy) | 4.90×10−7 | [2] |
| Stainless steel | 6.90×10−7 | |
| Mercury | 9.80×10−7 | [2] |
| Manganese | 1.44×10−6 | |
| Nichrome (alloy) | 1.10×10−6 | [2][3] |
| Carbon (amorphous) | 5×10−4 — 8×10−4 | [3] |
| Carbon (graphite) parallel-basal plane | 2.5×10−6 — 5.0×10−6 | |
| Carbon (graphite) perpendicular-basal plane | 3×10−3 | |
| Gallium arsenide | 10−3 to 108 | |
| Germanium | 4.6×10−1 | [3][4] |
| Sea water | 2.1×10−1 | |
| Swimming pool water | 3.3×10−1 — 4.0×10−1 | |
| Drinking water | 2×101 — 2×103 | |
| Silicon | 2.3×103 | [2][3] |
| Wood (wet) | 103 — 104 | |
| Deionized water | 1.8×105 | |
| Glass | 1011 — 1015 | [3][4] |
| Carbon (diamond) | 1012 | |
| Hard rubber | 1013 | [3] |
| Air | 109 — 1015 | |
| Wood (dry) | 1014 — 1016 | |
| Sulfur | 1015 | [3] |
| Fused quartz | 7.5×1017 | [3] |
| PAT | 1021 | |
| Teflon | 1023 — 1025 |
It can be seen that the resistivity of copper and the resistivity of brass are both low, and considering their cost relative to silver and gold, they become cost-effective materials to use for many wires. Copper's resistivity and ease of use have led to it being widely used as a conductor material on printed circuit boards.
Occasionally, aluminum and especially copper are used due to their low resistivity. Most wires used for interconnection today are made of copper because it provides low resistivity at an affordable cost.
The resistivity of gold is also important because gold is used in some critical applications despite its cost. Gold plating is often found on high quality low current connectors where it provides the lowest contact resistance. The gold coating is very thin, but even so it is able to provide the required characteristics of the connectors.
Silver has a very low level of resistivity, but is not widely used due to its cost and because it tarnishes, which can result in higher contact resistance.
However, it is used in some radio transmitter coils where silver's low electrical resistivity reduces losses. When used for such purposes, silver was usually applied only to the existing copper wire. Coating the wire with silver allowed for significant cost savings compared to solid silver wire without significantly compromising performance.
It will be interesting➡ Instrumentation. Types and scope
Other materials in the electrical resistivity table may not have such obvious uses. Tantalum appears in the table because it is used in capacitors—nickel and palladium are used in the end connections of many surface mount components such as capacitors.
Quartz finds its main application as a piezoelectric resonant element. Quartz crystals are used as frequency-determining elements in many oscillators, where the high Q value allows for very frequency-stable circuits. They are similarly used in high efficiency filters. Quartz has a very high level of resistivity and is not a good conductor of electricity, meaning it is classified as a dielectric.
Concept of electrical resistance of a conductor
The classical definition explains electric current by the movement of “free” (valence) electrons. It is provided by the electric field created by the source. Movement in the metal is hampered not only by the normal components of the crystal lattice, but also by defective areas, impurities, and inhomogeneous areas. During collisions with obstacles, due to the transition of momentum into thermal energy, the temperature increases.
A good example is heating water with a boiler.
In gases, electrolytes and other materials the physics of the phenomenon is somewhat different. Linear relationships are observed in metals and other conductors. The basic relationships are expressed by the well-known formula of Ohm's law:
R (electrical resistance) = U (voltage) / I (current).
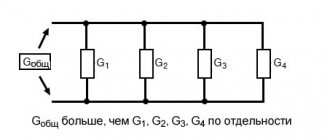
For convenience, the inverse quantity, conductivity (G = 1/R), is often used. It denotes the ability of a certain material to pass current with certain losses.
To simplify, the example of a water pipe is sometimes used. A moving fluid is an analogue of a current. Pressure is the equivalent of voltage. By decreasing (increasing) the cross section or position of the locking device, the conditions of movement are determined. In a similar way, the basic parameters of electrical circuits are changed using resistance (R).
For your information. The amount of liquid passing per unit time through the control section of the pipe is the equivalent of electrical power.
Temperature dependence ρ(T)
For most materials, numerous experiments have been carried out to measure resistivity values. Data for most conductors can be found in reference tables.
Specific resistance of metals and alloys, Ohm*mm2/m
(at T = 20C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
Most often, the values of ρ are given at normal, that is, room temperature 20C. But it turned out that with increasing temperature, the resistivity increases linearly in accordance with the formula:
$ ρ(T) = ρ0 * (1 + α*T)$ (6),
where: ρ is the resistivity of the conductor at a temperature of 0C, α is the temperature coefficient of resistivity, which also has its own individual meaning for each substance. From formula (6) it follows that the coefficient α has dimension or .
In accordance with the Joule-Lenz law, when an electric current flows, heat is released, which means the temperature of the conductor increases. In addition, depending on the area of application, electrical devices can operate at both low (minus) and high temperatures. For accurate calculations of electrical circuits, it is necessary to take into account the dependence ρ(T). The value of α for a specific material can be found in reference literature.
Selection of cable cross-section
Copper wire resistance
Because a wire has resistance, when electric current passes through it, heat is generated and a voltage drop occurs. Both of these factors must be taken into account when choosing cable cross-sections.
Selection by permissible heating
When current flows in a wire, energy is released. Its quantity can be calculated using the electric power formula:
In a copper wire with a cross section of 2.5 mm² and a length of 10 meters R = 10 * 0.0074 = 0.074 Ohm. At a current of 30A P=30²*0.074=66W.
This power heats the conductor and the cable itself. The temperature to which it heats up depends on the installation conditions, the number of cores in the cable and other factors, and the permissible temperature depends on the insulation material. Copper has greater conductivity, so the power output and the required cross-section are lower. It is determined using special tables or using an online calculator.
Table for selecting wire cross-section based on permissible heating
Permissible voltage loss
In addition to heating, when electric current passes through the wires, the voltage near the load decreases. This value can be calculated using Ohm's law:
Reference. According to PUE standards, it should be no more than 5% or in a 220V network - no more than 11V.
Therefore, the longer the cable, the larger its cross-section should be. You can determine it using tables or using an online calculator. In contrast to the choice of cross-section based on permissible heating, voltage losses do not depend on laying conditions and insulation material.
In a 220V network, voltage is supplied through two wires: phase and neutral, so the calculation is made using double the length of the cable. In the cable from the previous example it will be U=I*R=30A*2*0.074Ohm=4.44V. This is not much, but with a length of 25 meters it turns out to be 11.1V - the maximum permissible value, you will have to increase the cross-section.
Maximum permissible cable length of a given section
Electrical resistivity
Further research made it possible to establish a connection between the value of electrical resistance and its basic geometric dimensions. It turned out that the resistance of the conductor is directly proportional to the length of the conductor L and inversely proportional to the cross-sectional area of the conductor S.
This functional relationship is well described by the following formula:
$ R = ρ *{ Lover S} $ (4)
The constant value ρ for each substance was called resistivity. The value of this parameter depends on the density of the substance, its crystal structure, atomic structure and other internal characteristics of the substance. From formula (4) you can obtain a formula for calculating resistivity if experimental values for R, L and S are available:
$ ρ = R*{ Sover L } $ (5)
For most known substances, measurements were made and entered into reference tables of electrical resistance of conductors.
Specific resistance of metals, Ohm*mm2/m
(at T = 20C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
It was experimentally discovered that as the temperature decreases, the resistance of metals decreases. When approaching the temperature of absolute zero, which is -273C, the resistance of some metals tends to zero. This phenomenon is called superconductivity. Atoms and molecules seem to “froze”, stop any movement and offer no resistance to the flow of electrons.
How to calculate wire resistance - tips for novice electricians
Good day! I'm planning to connect an electric hob and oven at home myself.
Due to the fact that I heard that standard wiring may not withstand such a voltage and will begin to overheat, I decided to run separate wires from the panel through an additional circuit breaker. I already have the machine, but I don’t know how to select the wire cross-section.
Tell me how to calculate the resistance of the wires to suit my needs - I will have to throw 20 meters of wire, no less. The unit of electrical resistance was named after this man.
Reply to reader
Greetings, unfortunately unintroduced reader! Naturally, we will help you with the calculations, but we still recommend that you involve a specialist in the problem, because you will need to choose the right not only the conductor, but also the machine. However, if you know for sure that the parameters of the machine are suitable, then you have nothing left...
Theory and practice
So, if a person is even a little familiar with the basics of electrical engineering, he should know that the thicker the wire, the lower the resistance.
- Theoretically, this can be compared to a water pipe through which water flows. If the diameter of the pipe is sufficient, then the liquid flows through it without experiencing any hydraulic resistance, and vice versa, a small hole increases the pressure in the pipe, the throughput drops, and the hydraulic resistance increases.
- Also, the flow of electrons can be represented as water trying to flow inside the wire. However, electricity is a completely different nature, and accordingly its physical properties are different.
- What can cause too high resistance? The most common thing is a voltage drop, as a result of which some incandescent lamp will burn dimmer, and some electrical appliance will not be able to start.
- A direct consequence of the passage of a powerful current through a conductor with a sufficiently high resistance will be its overheating.
From the author! One day we connected the welding machine to, well, a very bad extension cord, and after a few minutes of work the wire literally caught fire. Fortunately, a short circuit did not occur, but it was very likely. As is clear, such situations are unacceptable in a residential area.
We recommend proceeding in the following sequence:
- First of all, find out exactly how much load both of your devices create when operating at maximum power. We are interested in current strength, measured in Amperes, or power - Watts.
- You can easily find these parameters in the product data sheets.
- If both devices are powered from the same line, then sum the resulting values.
- Next, use the table, which will allow you to accurately determine the wire cross-section.
The photo shows a table for selecting conductor cross-sections
- As can be seen from the table above, the maximum current for a copper wire with an area of 0.5 should not exceed 11 Amperes.
Advice! Today the use of aluminum wires is not allowed in residential premises. Only copper ones are used.
- In principle, one could limit ourselves to these data, adding some margin, but such tables do not show what the maximum resistance of the wire should be, that is, the length of the conductor is not taken into account. Therefore, for greater accuracy, calculations are indispensable.
Calculating resistance
All data can be obtained from tables
So, we remember - the wire is thicker, the resistance is less. The following will provide instructions on how to calculate everything accurately.
- To do this, we need to find out the resistivity of the conductor material. In ordinary networks you are unlikely to find silver wires, so we take standard copper as a basis. It is 0.017.
- The wire resistance itself is calculated using the following formula: ; where R is the resistance, p is the resistivity of the conductor, l is the length of the wire and s is its cross-sectional area.
- Let's assume that your stoves together can load a network of 16 Amps, this means that we can take a wire with an area of 0.75 mm2. We remember that you require a minimum of 20 meters. So, we consider: 0.017*20/0.75 = 0.45 Ohm
- You can use the table, but the result will not be as accurate. We see that 100 meters of copper wire of the cross-section we need has a resistance of 2.38 Ohms. We divide this value by five (up to 20 meters) and get 0.476 Ohms - the difference is at the level of error, but still.
- Due to the fact that electricity flows through two wires, we multiply the resulting value by 2 and get 0.9 Ohm.
- Now you can calculate the voltage loss using the formula: dU = R*I = 0.9*16 = 14.4 Volts.
- We convert the resulting voltage into a percentage: 14.4V/220V*100 = 6.54%
According to existing standards, 5% voltage loss is allowed. As you can see, in our case the value turned out to be larger, which means that the conductor resistance is too high, so we increase the cross-section of the wire and repeat the calculations.
So, we have found the resistance of the wire, and as you can see, doing this with your own hands and head is not so difficult. The attached video will help you understand the material further. Approach the matter wisely, because the price is the safety of you and your home.
Iron as a conductor in electrical engineering
Iron is the most common metal in nature and technology (after hydrogen, which is also a metal). It is the cheapest and has excellent strength characteristics, therefore it is used everywhere as the basis for the strength of various structures.
In electrical engineering, iron is used as a conductor in the form of flexible steel wires where physical strength and flexibility are needed, and the required resistance can be achieved through the appropriate cross-section.
Having a table of resistivities of various metals and alloys, you can calculate the cross-sections of wires made from different conductors.
As an example, let's try to find the electrically equivalent cross-section of conductors made of different materials: copper, tungsten, nickel and iron wire. Let's take aluminum wire with a cross-section of 2.5 mm as the initial one.
We need that over a length of 1 m the resistance of the wire made of all these metals is equal to the resistance of the original one. The resistance of aluminum per 1 m length and 2.5 mm section will be equal to
, where R is the resistance, ρ is the resistivity of the metal from the table, S is the cross-sectional area, L is the length.
Substituting the original values, we get the resistance of a meter-long piece of aluminum wire in ohms.
After this, let us solve the formula for S
, we will substitute the values from the table and obtain the cross-sectional areas for different metals.
So,
Since the resistivity in the table is measured on a wire 1 m long, in microohms per 1 mm2 section, then we got it in microohms. To get it in ohms, you need to multiply the value by 10-6. But we don’t necessarily need to get the number ohm with 6 zeros after the decimal point, since we still find the final result in mm2.
- Copper
- Tungsten
- Nikelin
- Iron
As you can see, the resistance of the iron is quite high, the wire is thick.
It will be interesting➡ How to connect an LED strip? Simple ways to connect LED strip
But there are materials for which it is even greater, for example, nickel or constantan.
Conductivity and electrical resistance
Since cable dimensions are measured in meters (length) and mm² (section), the electrical resistivity has the dimension Ohm mm²/m. Knowing the dimensions of the cable, its resistance is calculated using the formula:
In addition to electrical resistance, some formulas use the concept of “conductivity”. This is the reciprocal of resistance. It is designated “g” and is calculated using the formula:
Conductivity of liquids
The conductivity of liquids is different from the conductivity of metals. The charge carriers in them are ions. Their number and electrical conductivity increase when heated, so the power of the electrode boiler increases several times when heated from 20 to 100 degrees.
Interesting. Distilled water is an insulator. Dissolved impurities give it conductivity.
Electrical resistance of wires
The most common metals for making wires are copper and aluminum. Aluminum has a higher resistance, but is cheaper than copper. The resistivity of copper is lower, so the wire cross-section can be chosen smaller. In addition, it is stronger, and flexible stranded wires are made from this metal.
The following table shows the electrical resistivity of metals at 20 degrees. In order to determine it at other temperatures, the value from the table must be multiplied by a correction factor, different for each metal. You can find out this coefficient from the relevant reference books or using an online calculator.
Wire resistance
Why do metals have the lowest resistivities?
From the table above it can be seen that metals have the lowest resistivity values: silver, copper, gold, aluminum, etc. This property of metals is associated with a high concentration of free electrons, “not tied” to a specific atom, but wandering in the space of the crystal lattice. Voltage applied to the ends of a conductor creates an electric field that acts on the electrons, causing them to move in concert in the same direction.
Rice. 2. Electric current in metals, free electrons.
Silver has the lowest ρ value - 0.016 Ohm*mm2/m. But for widespread, mass use in power supply networks and equipment, this metal is not used due to its too high price. Silver is used to create the most critical contacts in special electrical devices. The following table shows the resistivity values of metals and alloys, commonly used metals in electrical engineering:
Table
Specific resistances of metals, Ohm*mm2/m
(at T = 200C)
| Silver | 0,016 | Bronze (alloy) | 0,1 |
| Copper | 0,017 | Tin | 0,12 |
| Gold | 0,024 | Steel (alloy) | 0,12 |
| Aluminum | 0,028 | Lead | 0,21 |
| Iridium | 0,047 | Nickelin (alloy) | 0,42 |
| Molybdenum | 0,054 | Manganin (alloy) | 0,45 |
| Tungsten | 0,055 | Constantan (alloy) | 0,48 |
| Zinc | 0,06 | Titanium | 0,58 |
| Brass (alloy) | 0,071 | Mercury | 0,958 |
| Nickel | 0,087 | Nichrome (alloy) | 1,1 |
| Platinum | 0,1 | Bismuth | 1,2 |
The most popular in electrical engineering are copper and aluminum. Copper and copper alloys are used to make cable products and shunts - parts that limit large currents through measuring instruments.
Resistivity of metals, electrolytes and substances (Table)
The reference table gives the values of resistivity p of some metals and insulators at a temperature of 18-20 ° C, expressed in ohm cm.
The value of p for metals strongly depends on impurities; the table shows the values of p for chemically pure metals, and for insulators they are given approximately.
Metals and insulators are arranged in the table in order of increasing p values.
Metal resistivity table
| Pure metals | 104 ρ (ohm cm) | Pure metals | 104 ρ (ohm cm) |
| Silver | 0,016 | Chromium | 0,131 |
| Copper | 0,017 | Tantalum | 0,146 |
| Gold | 0,023 | Bronze 1) | 0,18 |
| Aluminum | 0,029 | Thorium | 0,18 |
| Duralumin | 0,0335 | Lead | 0,208 |
| Magnesium | 0,044 | Platinit 2) | 0,45 |
| Calcium | 0,046 | Antimony | 0,405 |
| Sodium | 0,047 | Argentan | 0,42 |
| Manganese | 0,05 | Nikelin | 0,33 |
| Iridium | 0,063 | Manganin | 0,43 |
| Tungsten | 0,053 | Constantan | 0,49 |
| Molybdenum | 0,054 | Wood alloy 3) | 0,52 (0°) |
| Rhodium | 0,047 | Osmium | 0,602 |
| Zinc | 0,061 | Alloy Rose 4) | 0,64 (0°) |
| Potassium | 0,066 | Chromel | 0,70-1,10 |
| Nickel | 0,070 | ||
| Cadmium | 0,076 | Invar | 0,81 |
| Brass | 0,08 | Mercury | 0,958 |
| Cobalt | 0,097 | Nichrome 5) | 1,10 |
| Iron | 0,10 | Bismuth | 1,19 |
| Palladium | 0,107 | Fechral 6) | 1,20 |
| Platinum | 0,110 | Graphite | 8,0 |
| Tin | 0,113 |
Table of resistivity of insulators
| Insulators | ρ (ohm cm) | Insulators | ρ (ohm cm) |
| Asbestos | 108 | Mica | 1015 |
| Slate | 108 | Mikanite | 1015 |
| Dry wood | 1010 | Porcelain | 2·1015 |
| Marble | 1010 | Sealing wax | 5·1015 |
| Celluloid | 2·1010 | Shellac | 1016 |
| Bakelite | 1011 | Rosin | 1016 |
| Getinax | 5·1011 | Quartz _|_ axis | 3·1016 |
| Diamond | 1012 | Sulfur | 1017 |
| Soda glass | 1012 | Polystyrene | 1017 |
| Pyrex glass | 2·1014 | Ebonite | 1018 |
| Quartz || axes | 1014 | Paraffin | 3·1018 |
| Fused quartz | 2·1014 | Amber | 1019 |
Resistivity of pure metals at low temperatures
The table gives the resistivity values (in ohm cm) of some pure metals at low temperatures (0°C).
| Pure metals | t (°C) | Resistivity, 104 ρ (ohm cm) |
| Bismuth | -200 | 0,348 |
| Gold | -262,8 | 0,00018 |
| Iron | -252,7 | 0,00011 |
| Copper | -258,6 | 0,00014 1 |
| Platinum | -265 | 0,0010 |
| Mercury | -183,5 | 0,0697 |
| Lead | -252,9 | 0,0059 |
| Silver | -258,6 | 0,00009 |
Resistance ratio Rt/Rq of pure metals at temperatures T ° K and 273 ° K
The reference table gives the ratio Rt/Rq of the resistances of pure metals at temperatures T ° K and 273 ° K.
| Pure metals | T (°C) | RT/R0 |
| Aluminum | 77,7 | 1,008 |
| 20,4 | 0,0075 | |
| Bismuth | 77,8 | 0,3255 |
| 20,4 | 0,0810 | |
| Tungsten | 78,2 | 0,1478 |
| 20,4 | 0,0317 | |
| Iron | 78,2 | 0,0741 |
| 20,4 | 0,0076 | |
| Gold | 78,8 | 0,2189 |
| 20,4 | 0,0060 | |
| Copper | 81,6 | 0,1440 |
| 20,4 | 0,0008 | |
| Molybdenum | 77,8 | 0,1370 |
| 20,4 | 0,0448 | |
| Nickel | 78,8 | 0,0919 |
| 20,4 | 0,0066 | |
| Tin | 79,0 | 0,2098 |
| 20,4 | 0,0116 | |
| Platinum | 91,4 | 0,2500 |
| 20,4 | 0,0061 | |
| Mercury | 90,1 | 0,2851 |
| 20,4 | 0,4900 | |
| Lead | 73,1 | 0,2321 |
| 20,5 | 0,0301 | |
| Silver | 78,8 | 0,1974 |
| 20,4 | 0,0100 | |
| Antimony | 77,7 | 0,2041 |
| 20,4 | 0,0319 | |
| Chromium | 80,0 | 0,1340 |
| 20,6 | 0,0533 | |
| Zinc | 83,7 | 0,2351 |
| 20,4 | 0,0087 |
Specific resistance of electrolytes
The table gives the values of the resistivity of electrolytes in ohm cm at a temperature of 18 ° C. The concentration of solutions is given in percentages, which determine the number of grams of anhydrous salt or acid in 100 g of solution.
| c (%) | NH4Cl | NaCl | ZnSO4 | CuSO4 | CON | NaOH | H2SO4 |
| 5 | 10,9 | 14,9 | 52,4 | 52,9 | 5,8 | 5,1 | 4,8 |
| 10 | 5,6 | 8,3 | 31,2 | 31,3 | 3,2 | 3,2 | 2,6 |
| 15 | 3,9 | 6,1 | 24,1 | 23,8 | 2,4 | 2,9 | 1,8 |
| 20 | 3,0 | 5,1 | 21,3 | — | 2,0 | 3,0 | 1,5 |
| 25 | 2,5 | 4,7 | 20,8 | — | 1,9 | 3,7 | 1,4 |
_______________
Source of information: BRIEF PHYSICAL AND TECHNICAL GUIDE / Volume 1, - M.: 1960.
Effect of temperature on resistivity
In reference books, the values of ρ of metals are given at room temperature 200C. But experiments have shown that the dependence ρ(T) is linear and is described by the formula:
$ ρ(T) = ρ0 * (1 + α*T)$ (3),
where: ρ0 is the resistivity of the conductor at a temperature of 00C, α is the temperature coefficient of resistance, which is also individual for each substance. Values of α obtained experimentally can be found in reference books. Below are α values for some metals:
- Silver - 0.0035;
- Copper - 0.004;
- Aluminum - 0.004;
- Iron - 0.0066;
- Platinum - 0.0032;
- Tungsten - 0.0045.
Thus, as the temperature increases, the resistance of metals increases. This is explained by the fact that with increasing temperature, the number of defects in the crystal lattice increases due to more intense thermal vibrations of the ions, which inhibit the electron current.
Temperature dependence of resistivity of metals.
As the metal temperature approaches absolute zero, the resistivity drops sharply to zero. This phenomenon is called superconductivity, and materials that exhibit this ability are called superconductors. This effect was discovered in 1911 by the Dutch physicist Kamerlingh Onnes. In his experiment, the resistivity of mercury decreased to zero at 4.10K.
Properties of resistive materials
The resistivity of a metal depends on temperature. Their values are usually given for room temperature (20°C). The change in resistivity as a result of a change in temperature is characterized by a temperature coefficient.
For example, thermistors (thermistors) use this property to measure temperature. On the other hand, in precision electronics, this is a rather undesirable effect. Metal film resistors have excellent temperature stability properties. This is achieved not only due to the low resistivity of the material, but also due to the mechanical design of the resistor itself.
Many different materials and alloys are used in the manufacture of resistors. Nichrome (an alloy of nickel and chromium), due to its high resistivity and resistance to oxidation at high temperatures, is often used as a material for making wirewound resistors. Its disadvantage is that it cannot be soldered. Constantan, another popular material, is easy to solder and has a lower temperature coefficient.
Electrical resistance of other metals
Current resistance: formula
In addition to copper and aluminum, other metals and alloys are used in electrical engineering:
- Iron. Steel has a higher resistivity, but is stronger than copper and aluminum. Steel strands are woven into cables designed to be laid through the air. The resistance of iron is too high to transmit electricity, so the core cross-sections are not taken into account when calculating the cross-section. In addition, it is more refractory, and leads are made from it for connecting heaters in high-power electric furnaces;
- Nichrome (an alloy of nickel and chromium) and fechral (iron, chromium and aluminum). They have low conductivity and refractoriness. Wirewound resistors and heaters are made from these alloys;
- Tungsten. Its electrical resistance is high, but it is a refractory metal (3422 °C). It is used to make filaments in electric lamps and electrodes for argon-arc welding;
- Constantan and manganin (copper, nickel and manganese). The resistivity of these conductors does not change with changes in temperature. Used in high-precision devices for the manufacture of resistors;
- Precious metals – gold and silver. They have the highest specific conductivity, but due to their high price, their use is limited.
High conductivity materials
The most widespread materials of high conductivity include copper and aluminum (Superconducting materials, which have a typical resistance 10-20 times lower than ordinary conductive materials (metals), are discussed in the section Superconductivity).
Copper
The advantages of copper, which ensure its widespread use as a conductor material, are as follows:
- low resistivity;
- sufficiently high mechanical strength;
- corrosion resistance is satisfactory in most applications;
- good workability: copper is rolled into sheets, strips and drawn into wire, the thickness of which can be increased to thousandths of a millimeter;
- relative ease of soldering and welding.
Copper is most often obtained by processing sulfide ores. After a series of ore smelting and roasting with intense blasting, copper intended for electrical purposes must undergo a process of electrolytic purification.
Copper grades M1 and M0 are most often used as conductor material. M1 grade copper contains 99.9% Cu, and in the total amount of impurities (0.1%) oxygen should be no more than 0.08%. The presence of oxygen in copper worsens its mechanical properties. The best mechanical properties are found in M0 grade copper, which contains no more than 0.05% impurities, including no more than 0.02% oxygen.
Copper is a relatively expensive and scarce material, so it is increasingly being replaced by other metals, especially aluminum.
In some cases, alloys of copper with tin, silicon, phosphorus, beryllium, chromium, magnesium, and cadmium are used. Such alloys, called bronzes, with the correct composition, have significantly higher mechanical properties than pure copper.
Aluminum
Aluminum is the second most important conductor material after copper. This is the most important representative of the so-called light metals: the density of cast aluminum is about 2.6, and rolled aluminum is 2.7 Mg/m3. Thus, aluminum is approximately 3.5 times lighter than copper. The temperature coefficient of expansion, specific heat capacity and heat of fusion of aluminum are greater than those of copper. Due to the high values of specific heat capacity and heat of fusion, heating aluminum to the melting point and transferring it to a molten state requires more heat than heating and melting the same amount of copper, although the melting point of aluminum is lower than that of copper.
Aluminum has lower properties compared to copper - both mechanical and electrical. With the same cross-section and length, the electrical resistance of an aluminum wire is 1.63 times greater than that of a copper wire. It is very important that aluminum is less scarce than copper.
For electrical purposes, aluminum containing no more than 0.5% impurities, grade A1, is used. Even purer AB00 grade aluminum (no more than 0.03% impurities) is used for the manufacture of aluminum foil, electrodes and housings of electrolytic capacitors. Aluminum of the highest purity AB0000 has an impurity content of no more than 0.004%. Additives of Ni, Si, Zn or Fe at a content of 0.5% reduce the γ of annealed aluminum by no more than 2-3%. A more noticeable effect is exerted by Cu, Ag and Mg impurities, which, at the same mass content, reduce γ aluminum by 5-10%. Ti and Mn greatly reduce the electrical conductivity of aluminum.
Aluminum oxidizes very actively and becomes covered with a thin oxide film with high electrical resistance. This film protects the metal from further corrosion.
It will be interesting➡ Choke for fluorescent lamps
Aluminum alloys have increased mechanical strength. An example of such an alloy is Aldrey, containing 0.3-0.5% Mg, 0.4-0.7% Si and 0.2-0.3% Fe. In aldrey, the Mg2Si compound is formed, which imparts high mechanical properties to the alloy.
Iron and steel
Iron (steel), as the cheapest and most accessible metal, which also has high mechanical strength, is of great interest for use as a conductor material. However, even pure iron has a significantly higher resistivity compared to copper and aluminum; ρ steel, i.e. iron mixed with carbon and other elements is even higher. Ordinary steel has low corrosion resistance: even at normal temperatures, especially in conditions of high humidity, it quickly rusts; As the temperature rises, the corrosion rate increases sharply. Therefore, the surface of steel wires must be protected by a layer of more resistant material. Zinc coating is usually used for this purpose.
In some cases, to reduce the consumption of non-ferrous metals, the so-called bimetal is used. It is steel coated on the outside with a layer of copper, with both metals connected to each other firmly and continuously.
Sodium
Sodium metal is a very promising conductor material. Sodium can be obtained by electrolysis of molten sodium chloride NaCl in virtually unlimited quantities. From a comparison of the properties of sodium with the properties of other conductor metals, it is clear that the resistivity of sodium is approximately 2.8 times greater than ρ of copper and 1.7 times greater than ρ of aluminum, but due to the extremely low density of sodium (its density is almost 9 times less than the density of copper), a wire made of sodium for a given conductivity per unit length should be significantly lighter than a wire made of any other metal. However, sodium is extremely active chemically (it oxidizes intensely in air and reacts violently with water), which is why the sodium wire must be protected with a sealing sheath. The sheath must give the wire the necessary mechanical strength, since sodium is very soft and has a low tensile strength during deformation.
AISI 304
International standard American ASTM A240 European EN 10088-2 Russian GOST 5632-72
| Brand designation | AISI 304 | 1.4301 | 08Х18Н10 |
| 12Х18Н9 |
AMS 5513 ASTM A 240
ASTM A 666
Classification
corrosion-resistant, heat-resistant steel
Application
- Household items
- Sinks
- Frames for metal structures in the construction industry
- Kitchen utensils and catering equipment
- Dairy equipment, brewing
- Welded structures
- Tanks on ships and land tankers for food, beverages and some chemicals
Typically, steel manufacturers divide the grade into three main classes (grades) according to their drawing ability:
- AISI 304 - Main grade
- AISI 304 DDQ (Normal and deep drawing) - Deep drawing grade
- AISI 304 DDS (Extra deep drawing) - Extra deep drawing grade
Main characteristics
- good overall corrosion resistance
- good ductility
- excellent weldability
Chemical composition (% by weight)
standard grade C Si Mn PS Cr Ni
| ASTM A240 | AISI 304 | ≤0.080 | ≤0.75 | ≤2.0 | ≤0.045 | ≤0.030 | 18.00 — 20.00 | 8.00 — 10.50 |
Mechanical properties
AISI 304 Tensile strength (σв), N/mm² Yield strength (σ0.2), N/mm² Yield strength (σ1.0), N/mm² Elongation (σ), % Brinell hardness (HB) Rockwell hardness (HRB)
| According to EN 10088-2 | ≥520 | ≥210 | ≥250 | ≥45 | — | — |
| According to ASTM A 240 | ≥515 | ≥205 | — | ≥40 | 202 | 85 |
Mechanical properties at high temperatures
All these values refer only to AISI 304
.
Physical properties
Physical properties Symbols Unit of measurement Temperature Value
| Density | d | — | 4°C | 7.93 |
| Melting temperature | °C | 1450 | ||
| Specific heat | c | J/kg.K | 20°C | 500 |
| Thermal expansion | k | W/mK | 20°C | 15 |
| Average coefficient of thermal expansion | α | 10-6.K-1 | 0-100°C 0-200°C | 17.5 18 |
| Electrical resistivity | ρ | Ωmm2/m | 20°C | 0.80 |
| Magnetic permeability | μ | at 0.80 kA/m DC or h/h AC | 20°C μ μ discharge air | 1.02 |
| Elastic modulus | E | MPa x 103 | 20°C | 200 |
Corrosion resistance
304 steels have good resistance to general corrosive environments, but are not recommended where there is a risk of intergranular corrosion. They are well suited for use in fresh water and urban and rural environments. In all cases, regular cleaning of external surfaces is necessary to maintain their original condition.
304 steels have good resistance to various acids:
- phosphoric acid in all concentrations at ambient temperature,
- nitric acid up to 65% at temperatures 20°C - 50°C,
- formic and lactic acid at room temperature,
- acetic acid at a temperature of 20°C - 50°C.
They are recommended for the production of equipment in contact with cold or hot food products: wine, beer, milk (fermented milk products), alcohol, natural fruit juices, syrups, molasses, etc.
Acidic environments
Temperature, °C 20 80
| Concentration, % by weight | 10 | 20 | 40 | 60 | 80 | 100 | 10 | 20 | 40 | 60 | 80 | 100 |
| Sulfuric acid | 2 | 2 | 2 | 2 | 1 | 0 | 2 | 2 | 2 | 2 | 2 | 2 |
| Nitric acid | 0 | 0 | 0 | 0 | 2 | 0 | 0 | 0 | 0 | 0 | 1 | 2 |
| Phosphoric acid | 0 | 0 | 0 | 0 | 0 | 2 | 0 | 0 | 0 | 0 | 1 | 2 |
| Formic acid | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 2 | 2 | 1 | 0 |
Code: 0 = high degree of protection - Corrosion rate less than 100 µm/year 1 = partial protection - Corrosion rate from 100 to 1000 µm/year
2 = no protection - Corrosion rate more than 1000 µm/year
Atmospheric influences
Comparison of 304
grades with other metals in various environments (Corrosion rate calculated for 10-year exposure).
Environment Corrosion rate (µm/year)AISI 304 Aluminum-3S Carbon steel
| Rural | 0.0025 | 0.025 | 5.8 |
| Marine | 0.0076 | 0.432 | 34.0 |
| Industrial Marine | 0.0076 | 0.686 | 46.2 |
Resistant to corrosion in boiling chemicals
Boiling environment Metal condition Corrosion rate (mm/year)
| 20% acetic acid | Regular metal Welded |
What is the resistance of copper wire
In metals, a current is formed when an electric field appears. It “forces” electrons to move in an orderly manner, in one direction. Electrons from the distant orbits of an atom, weakly held by the nucleus, form a current.
Copper wires
As negative particles pass through the crystal lattice of copper molecules, they collide with atoms and other electrons. There is an obstacle or resistance to the directional movement of particles.
To evaluate the resistance to current, the value of “electrical resistance” or “electrical impedance” was introduced. It is designated by the letter “R” or “r”. Resistance is calculated using Georg Ohm's formula: R=, where U is the potential difference or voltage acting on a section of the circuit, I is the current strength.
Concept of resistance
Important! The higher the impedance value of a metal, the less current passes through it, and it is copper conductors that are so widespread in electrical engineering due to this property.
Based on Ohm's formula, the magnitude of the current is affected by the applied voltage at a constant R. But the resistance of copper wires varies depending on their physical characteristics and operating conditions.
What you need to know about electrical processes
In simple terms, resistance is usually understood as a property of the medium through which electric current flows, which reduces its magnitude.
This is how the high-voltage power line wires and insulators shown in the top picture, or indeed any substance, work.
Insulators have very high dielectric properties and isolate the high-voltage voltage present on the busbars from the ground circuit. This is their main purpose.
Wires must transmit the power transmitted through them as efficiently as possible. They are created so that they have minimal electrical resistance and operate with the least energy loss due to heating.
In this case, the transmission of electricity from the voltage source to the consumer over any distance will be efficient.
Let me give you an example of a picture from my previous article.
It, like the top one, can be represented in this generalized form.
In the external section of the circuit, the current-carrying conductors are separated from each other by an air environment and an insulation layer with high dielectric properties.
Current-carrying conductors have good conductivity. The electrical appliance connected to them functions optimally.
How does a resistor work?
Current in metals passes under the influence of applied voltage due to the directed movement of electrons. At the same time, they collide and meet with positively and negatively charged ions.
Such collisions increase the temperature of the medium and reduce the current strength. In electrical engineering, the direction of electric current is taken to be the movement of charged particles from plus to minus. Electrons move from the cathode to the anode.
The electrical resistance of a metal depends on its structure and geometric dimensions.
Similar processes occur in any other conductive medium, including gases or liquids.
What types of resistance are there?
Household electrical appliances use a wide variety of resistors, either fixed or adjustable.
They limit the current of all household devices, and in the most complex modules their number can reach a thousand or more. Resistors work in almost all circuits.
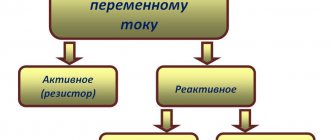
When used in AC circuits, they have active resistance, while capacitors and chokes have reactive resistance.
Moreover, capacitive resistance is created on capacitors, and inductive resistance is created on chokes.
The reactive component on capacitors and chokes strongly depends on the frequency of the electromagnetic oscillation.
2 Electricians joke about currents through a capacitor and inductor
I present them because they allow you to remember the nature of the passage of current through reactive elements.
Joke #1 about capacity
In the home network and inside many appliances, alternating and direct currents operate. They behave differently if they encounter a capacitor on their way.
Since it consists of two conductive plates separated by a dielectric layer, it is designated in the diagrams by two bold lines located in parallel. Wires drawn with perpendicular lines are connected to their centers.
Alternating current has the form of a harmonic sinusoid consisting of two symmetrical halves.
Such a harmonic moves from the origin of coordinates, encounters plates on its way, rolls over them and, having rolled off, begins to overtake the applied voltage.
Direct current does not have this property. Its blunt end simply rests against the trim and stops. It cannot pass through the capacitor. This is an insurmountable obstacle for him.
Joke #2 about the throttle
The inductance is made of turns of insulated wire. Any current passes through it. But the sinusoid, with its waves, gets confused in the turns of the coil and begins to lag behind the voltage.
The constant moves calmly inside the throttle wire without feeling any significant resistance. Therefore, a constant voltage can burn a choke with its current, designed to work at alternating periods.
What kind of beast is this: superconductivity
A hundred years ago, the ability of certain metals to completely lose their resistance to electric current at ultra-low temperatures was discovered. This process looks like this:
The DIYer does not work with superconductors. But I recommend paying attention to the upper part of the above graph: heating the metal increases its electrical resistance.
When performing electrical calculations that require obtaining an accurate result, it is necessary to take into account the temperature coefficient taken from reference books.
What affects the resistance of a copper wire
The electrical impedance of a copper cable depends on several factors:
- Specific resistance;
- Wire cross-sectional area;
- Wire lengths;
- External temperature.
The last point can be neglected in conditions of domestic use of the cable. A noticeable change in impedance occurs at temperatures above 100°C.
Resistance dependence
Resistivity in the SI system is denoted by the letter ρ. It is defined as the resistance value of a conductor having a cross-section of 1 m2 and a length of 1 m, measured in Ohm ∙ m2. This dimension is inconvenient in electrical calculations, so the unit of measurement Ohm ∙ mm2 is often used.
Important! This parameter is a characteristic of the substance - copper. It does not depend on the shape or cross-sectional area. The purity of the copper, the presence of impurities, the method of making the wire, and the temperature of the conductor are factors that affect the resistivity.
The dependence of the parameter on temperature is described by the following formula: ρt= ρ20[1+ α(t−20°C)]. Here ρ20 is the resistivity of copper at 20°C, α is an empirically found coefficient, from 0°C to 100°C for copper it has a value equal to 0.004 °C-1, t is the temperature of the conductor.
Below is a table of ρ values for different metals at a temperature of 20°C.
Resistivity table
According to the table, copper has a low resistivity, lower only for silver. This ensures good conductivity of the metal.
The thicker the wire, the lower its resistance. The dependence of R of the conductor on the cross-section is called “inversely proportional”.
Important! As the cross-sectional area of the cable increases, it is easier for electrons to pass through the crystal lattice. Therefore, with increasing load and increasing current density, the cross-sectional area should be increased.
An increase in the length of a copper cable entails an increase in its resistance. Impedance is directly proportional to the length of the wire. The longer the conductor, the more atoms there are in the path of free electrons.
conclusions
The last element that affects the resistance of copper is the temperature of the environment. The higher it is, the greater the amplitude of movement of the atoms of the crystal lattice. Thus, they create an additional obstacle for electrons participating in directed movement.
Important! If you lower the temperature to absolute zero, which has a value of 0° K or -273°C, then the opposite effect will be observed - the phenomenon of superconductivity. In this state, the substance has zero resistance.
Temperature correlation
Electrical conductivity
So far, we have considered the resistance of a conductor as the obstacle that the conductor provides to the electric current. But still, current flows through the conductor. Therefore, in addition to resistance (obstacle), the conductor also has the ability to conduct electric current, that is, conductivity.
The more resistance a conductor has, the less conductivity it has, the worse it conducts electric current, and, conversely, the lower the resistance of a conductor, the more conductivity it has, the easier it is for current to pass through the conductor. Therefore, the resistance and conductivity of a conductor are reciprocal quantities.
From mathematics we know that the reciprocal of 5 is 1/5 and, conversely, the reciprocal of 1/7 is 7. Therefore, if the resistance of a conductor is denoted by the letter r, then the conductivity is defined as 1/r. Conductivity is usually symbolized by the letter g.
Electrical conductivity is measured in (1/Ohm) or in siemens.
Example 8. The conductor resistance is 20 ohms. Determine its conductivity.
If r = 20 Ohm, then
Example 9. The conductivity of the conductor is 0.1 (1/Ohm). Determine its resistance
If g = 0.1 (1/Ohm), then r = 1 / 0.1 = 10 (Ohm)
Comparison of conductivity of different types of steel
The characteristics of steel depend on its composition and temperature:
- For carbon alloys, the resistance is quite low: it is 0.13-0.2 μOhm/m. The higher the temperature, the greater the value;
- Low-alloy alloys have a higher resistance - 0.2-0.43 μOhm/m;
- High-alloy steels have high resistance - 0.3-0.86 μOhm/m;
- Due to the high chromium content, the resistance of chromium stainless alloys is 0.5-0.6 μOhm/m;
- Chromium-nickel austenitic steels are stainless and, thanks to nickel, have a high resistance of 0.7-0.9 μOhm/m.
Copper ranks second in terms of electrical conductivity: it perfectly passes electric current and is widely used in the manufacture of wires. Aluminum is also used no less often: it is weaker than copper, but cheaper and lighter.
Active resistance of wires, cables and lines
Due to the fact that alternating current flows unevenly, under the same conditions, alternating and direct current R will be different. As already mentioned, steel electrical wires have a better active R compared to conductors made of non-ferrous metals, which have the same R at any current strength.
On the contrary, the active R of steel electrical cables always depends on the electric current, so DC conductivity is never used in this case. The active R of an electrical cable is determined using the formula: R=l/y*s.
Inductive reactance
Formulas for calculating the conductivity of wires are valid only in a direct current network or in straight conductors at low frequencies. Inductive reactance appears in coils and in high-frequency networks, many times higher than usual. In addition, high frequency current only travels along the surface of the wire. Therefore, it is sometimes coated with a thin layer of silver or Litz wire is used.
Reference. Litz wire is a stranded wire, each core in which is isolated from the rest. This is done to increase surface area and conductivity in high frequency networks.
Copper's resistivity, flexibility, relatively low price and mechanical strength make this metal, along with aluminum, the most common material for making wires.
Source